Digital representation of dynamic processes makes it difficult to perceive and complicates the calculation of output parameters after changes in input conditions or as a result of processing performed. A vector diagram of currents and voltages helps to successfully solve the indicated problems. Familiarization with theory and practical examples will help you master this technology.
Diagram explaining the short circuit process in a three-phase electricity meter circuit
Types of vector diagrams
Vector graphics are well suited for correctly displaying variables that determine the functionality of radio devices. This implies a corresponding change in the basic parameters of the signal along a standard sinusoidal (cosine) curve. To visualize the process, harmonic oscillation is represented as a projection of a vector onto the coordinate axis.
Using standard formulas, it is easy to calculate the length, which will be equal to the amplitude at a certain point in time. The tilt angle will indicate the phase. The total influences and corresponding changes in vectors obey the usual rules of geometry.
There are high-quality and accurate diagrams. The first ones are used to take into account mutual connections. They help make a preliminary assessment or are used to completely replace calculations. Others create taking into account the results obtained, which determine the size and direction of individual vectors.
Pie chart
Let us assume that we need to study the change in current parameters in a circuit for different values of resistor resistance in the range from zero to infinity. In this circuit, the output voltage (U) will be equal to the sum of the values (UR and UL) on each of the elements. The inductive nature of the second quantity implies a perpendicular relative position, which is clearly visible in part of figure b). The triangles formed fit perfectly into the 180 degree circle segment. This curve corresponds to all possible points through which the end of the vector UR passes with a corresponding change in electrical resistance. The second diagram c) shows a current lag in phase by an angle of 90°.
Line chart
Shown here is a two-pole element with active and reactive conductivity components (G and jB, respectively). A classic oscillatory circuit created using a parallel circuit has similar parameters. The parameters noted above can be represented by vectors that are constantly located at an angle of 90°. A change in the reactive component is accompanied by a movement of the current vector (I1...I3). The formed line is located perpendicular to U and at a distance Ia from the zero point of the coordinate axis.
Topographic vector diagram
A special case of a vector diagram is a topographic vector diagram , on which the complex potentials of individual points of the circuit are plotted with respect to one point, the potential of which is assumed to be zero. The order of the vectors on the topographic diagram corresponds to the order of the elements of the circuit.
Note that, by definition, a topographic diagram is used as a geometric interpretation of Kirchhoff’s second law (i.e., stress vectors are plotted on it).
There are two ways to construct a topographic diagram.
Method I.
They build by moving along the circuit elements in a direction coinciding with the direction of the current. In this case, the voltage vector on the diagram and the corresponding voltage arrow on the diagram are oriented in the same way - from the highest potential to the lowest.
Let us consider as an example the circuit in Fig. 3.15.
Rice. 3.15
Let us plot the current vector on the complex plane at an angle to the real axis (Fig. 3.16).
Rice. 3.16
Let us denote the intermediate points of the chain under consideration by the letters a, b, d, h. We will go around the circuit in the direction of the current (i.e., clockwise), taking the complex potential of point a equal to zero. The latter leads to the fact that on the complex plane point a is located at the origin of coordinates (Fig. 3.16).
When moving in the chosen direction along the elements of the chain from point a (Fig. 3.15), the first element of the chain is capacitance c. On the topographic diagram from point a we plot the voltage vector on the capacitance, which lags the current by an angle (Fig. 3.17). The end of the vector determines the value of the complex potential of point b on the vector diagram.
The next element of the circuit when moving in the direction of the current is resistance r (see Fig. 3.15). We plot on the topographic diagram the voltage vector across the resistance, which coincides in direction with the current vector (Fig. 3.18).
Rice. 3.17
Rice. 3.18
The end of the vector determines the value of the complex potential of point d on the vector diagram.
The next element of the circuit when moving in the direction of the current is inductance L (see Fig. 3.15). We plot on the topographic diagram the voltage vector across the inductance, which is ahead of the current vector (Fig. 3.19). The end of the vector determines the value of the complex potential of point h on the vector diagram.
The potential difference between points a and h is equal to the input voltage of the circuit (see Fig. 3.15). To obtain the corresponding vector on the diagram, you need to connect points a and h with a straight line. The end of the vector on the diagram should be directed in the same way as the voltage arrow on the diagram - from point a to point h (Fig. 3.20).
Rice. 3.19
Rice. 3.20
The angle between the voltage and current vectors (Fig. 3.20) is equal to the phase angle. In this case, the input voltage leads the current and the circuit has an active-inductive character.
Method 2.
They build by moving along the circuit elements in the direction opposite to the direction of the current. In this case, the voltage vector on the diagram is directed from the point of lowest potential to the point of highest potential. The same voltage is indicated on the diagram by an arrow in the opposite direction.
So, we will go around the circuit in Fig. 3.21 in the direction opposite to the current, taking the complex potential of point h equal to zero.
Rice. 3.21
The latter leads to the fact that on the complex plane point h is located at the origin of coordinates (Fig. 3.22).
Rice. 3.22
When moving in the chosen direction along the circuit elements from point h (Fig. 3.21), the first element of the circuit is inductance L. On the topographic diagram from point h we plot the voltage vector across the inductance, which is ahead of the current vector (Fig. 3.23). The end of the vector determines the value of the complex potential of point d on the vector diagram.
Rice. 3.23
Continuing the movement in the chosen direction along the elements of the chain and carrying out similar constructions, we obtain the resulting topographic diagram in Fig. 3.24.
Rice. 3.24
Comparison of vector diagrams Fig. 3.20 and 3.24, constructed in two ways, shows their complete identity (vectors of the same quantities in the diagrams have the same direction). The area of primary use of the second method is three-phase circuits.
A special case of a vector diagram is a topographic vector diagram , on which the complex potentials of individual points of the circuit are plotted with respect to one point, the potential of which is assumed to be zero. The order of the vectors on the topographic diagram corresponds to the order of the elements of the circuit.
Note that, by definition, a topographic diagram is used as a geometric interpretation of Kirchhoff’s second law (i.e., stress vectors are plotted on it).
There are two ways to construct a topographic diagram.
Method I.
They build by moving along the circuit elements in a direction coinciding with the direction of the current. In this case, the voltage vector on the diagram and the corresponding voltage arrow on the diagram are oriented in the same way - from the highest potential to the lowest.
Let us consider as an example the circuit in Fig. 3.15.
Rice. 3.15
Let us plot the current vector on the complex plane at an angle to the real axis (Fig. 3.16).
Rice. 3.16
Let us denote the intermediate points of the chain under consideration by the letters a, b, d, h. We will go around the circuit in the direction of the current (i.e., clockwise), taking the complex potential of point a equal to zero. The latter leads to the fact that on the complex plane point a is located at the origin of coordinates (Fig. 3.16).
When moving in the chosen direction along the elements of the chain from point a (Fig. 3.15), the first element of the chain is capacitance c. On the topographic diagram from point a we plot the voltage vector on the capacitance, which lags the current by an angle (Fig. 3.17). The end of the vector determines the value of the complex potential of point b on the vector diagram.
The next element of the circuit when moving in the direction of the current is resistance r (see Fig. 3.15). We plot on the topographic diagram the voltage vector across the resistance, which coincides in direction with the current vector (Fig. 3.18).
Rice. 3.17
Rice. 3.18
The end of the vector determines the value of the complex potential of point d on the vector diagram.
The next element of the circuit when moving in the direction of the current is inductance L (see Fig. 3.15). We plot on the topographic diagram the voltage vector across the inductance, which is ahead of the current vector (Fig. 3.19). The end of the vector determines the value of the complex potential of point h on the vector diagram.
The potential difference between points a and h is equal to the input voltage of the circuit (see Fig. 3.15). To obtain the corresponding vector on the diagram, you need to connect points a and h with a straight line. The end of the vector on the diagram should be directed in the same way as the voltage arrow on the diagram - from point a to point h (Fig. 3.20).
Rice. 3.19
Rice. 3.20
The angle between the voltage and current vectors (Fig. 3.20) is equal to the phase angle. In this case, the input voltage leads the current and the circuit has an active-inductive character.
Method 2.
They build by moving along the circuit elements in the direction opposite to the direction of the current. In this case, the voltage vector on the diagram is directed from the point of lowest potential to the point of highest potential. The same voltage is indicated on the diagram by an arrow in the opposite direction.
So, we will go around the circuit in Fig. 3.21 in the direction opposite to the current, taking the complex potential of point h equal to zero.
Rice. 3.21
The latter leads to the fact that on the complex plane point h is located at the origin of coordinates (Fig. 3.22).
Rice. 3.22
When moving in the chosen direction along the circuit elements from point h (Fig. 3.21), the first element of the circuit is inductance L. On the topographic diagram from point h we plot the voltage vector across the inductance, which is ahead of the current vector (Fig. 3.23). The end of the vector determines the value of the complex potential of point d on the vector diagram.
Rice. 3.23
Continuing the movement in the chosen direction along the elements of the chain and carrying out similar constructions, we obtain the resulting topographic diagram in Fig. 3.24.
Rice. 3.24
Comparison of vector diagrams Fig. 3.20 and 3.24, constructed in two ways, shows their complete identity (vectors of the same quantities in the diagrams have the same direction). The area of primary use of the second method is three-phase circuits.
Vector diagrams and complex representation
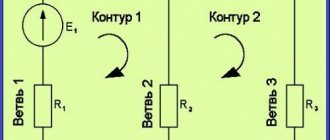
Loop current method
Such tools help to build visual graphic diagrams of oscillatory processes. A similar result is achieved by using complex numerical expressions. In this version, in addition to the axis with real values, an additional coordinate segment with imaginary values is used. To represent a vector, use the formula A * ei ( wt + f 0) , where:
- A – length;
- W – angular velocity;
- f0 – starting angle.
The value of the real part is A*cos*(w*t+f0). This expression describes a typical harmonic oscillation with basic characteristics.
Application examples
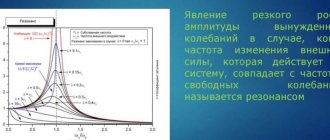
Resonance conditions
The following sections provide descriptions of the problems that can be solved using the presented methodology. It should be emphasized that the use of complex numbers is suitable for complex calculations with high accuracy. However, in practice, it is often quite common to use relatively simple vector graphics with a visual display of the original information in one picture.
Mechanics, harmonic oscillator
This term refers to a device that can be removed from an equilibrium state. After this, the system returns towards its original position, and the force (F) of the corresponding impact depends on the distance of the primary movement (d) in direct proportion. Its value can be clarified using a constant correction factor (k). The above definitions are related by the formula F=-d*k
Formulas for calculating the main parameters of a harmonic oscillator
For your information. Similar processes occur in systems of a different nature. An example is the creation of an analogue based on an electrical oscillatory circuit (series or parallel). The formulas remain the same with the corresponding parameters replaced.
Free harmonic oscillations without damping
Continuing the study of the topic using examples of mechanical processes, we can note the possibility of constructing a two-dimensional diagram. In this case, the speed is displayed on the X-axis in the same way as in the one-dimensional version. However, here we can additionally take into account the factor of acceleration, which is directed at an angle of 90° to the previous vector.
Harmonic oscillator with damping and external driving force
In this case, you can also use vector graphics to study the mutual influence of additional factors. As in the previous example, speed and other quantities are represented in two dimensions. To correctly model the process, the total influence of external forces is checked. It is directed to the center of the system (equilibrium point). Using geometric formulas, the amplitude of mechanical vibrations after the initial impact is calculated, taking into account the damping coefficient and other significant factors.
Calculation of electrical circuits
Vector graphics are used for relatively simple circuits that are created from a set of elements of the linear category: capacitors, resistors, inductors. For more complex circuits, the “Complex Amplitudes” calculation method is used, in which reactive components are determined using impedances.
Vector diagram for connection diagram without neutral wire - star
The vector diagram in this case serves as an auxiliary drawing that simplifies the solution of geometric problems. For coils and capacitors, in order not to use complex calculus, a special term is introduced - reactance. With a sinusoidal current, the change in voltage on the inductive element is described by the formula U=-L*w*I0sin(w*t+f0).
It is easy to see the similarity with the classical Ohm's law. However, in this example the phase changes. According to this parameter, the voltage on the capacitor lags behind the current by 90°. In inductance there is an inverse distribution. These features are taken into account when placing vectors in the figure. The formula takes into account the frequency, which affects the value of this element.
Circuits and vector diagrams for an ideal element and a lossy dielectric
Fourier transform
Vector technologies are used to analyze the spectra of radio signals in a certain range. Despite the simplicity of the technique, it is quite suitable for obtaining fairly accurate results.
Addition of two sinusoidal oscillations
When studying such signal sources, it is recommended to work with relatively small frequency differences. This will help create a graph at a user-friendly scale.
Fourier transform of a square wave
In this example, we operate with the sum of sinusoidal signals. Successive addition of vectors forms a polygon rotating around a single point. For correct calculations, the differences between continuous and discrete spectrum distributions should be taken into account.
Diffraction
For this case, use the same display of individual sinusoids in the form of vectors, as in the previous example. The total value also fits within the circle.
Construction of a vector diagram of voltages and currents
Series and parallel connection of batteries
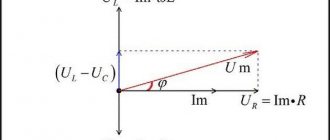
To study the technology, we will select a single-phase source of sinusoidal voltage (U). The current changes according to the formula I=Im*cos w*t. The connected circuit contains components connected in series with the following meanings:
- resistor: Ur=Im*R*cos w*t;
- capacitor: Uc=Im*Rc*cos (w*t-π/2), Rc=1/w*C;
- coil: UL= Im*RL*cos(w*t+π/2), RL=w*L.
When passing through an alternating current circuit, there will be a corresponding phase shift on the reactive elements. To construct vectors correctly, amplitudes are calculated and changes in directions are taken into account. Below is the sequence for creating graphics manually.
Diagram of voltages and currents on individual elements
Next, using elementary rules of geometry, the mutual influence of the vectors is checked.
Solving a vector equation
The first figure shows the result of adding two vectors under the condition that Uc is less than UL. By adding the value to the resistance, we get the resulting voltage Um. The third illustration shows the overall phase shift.
Vector display of processes in a parallel oscillatory circuit, voltage resonance
In a topographic diagram, the origin of coordinates is combined with the so-called “zero potential” point. This solution simplifies the study of individual sections of complex circuits.
Specialized online editor
On the Internet you can find a program for constructing vector diagrams online.
What is a vector diagram of currents and voltages? How to build a graph
The use of vector diagrams in the analysis and calculation of alternating current circuits makes it possible to consider the processes occurring in a more accessible and visual way, and also, in some cases, significantly simplify the calculations performed.
A vector diagram is usually called a geometric representation of directed segments varying according to a sinusoidal (or cosine) law - vectors that display the parameters and values of the operating sinusoidal currents, voltages or their amplitude values.
Vector diagrams are widely used in electrical engineering, vibration theory, acoustics, optics, etc.
There are 2 types of vector diagrams:
- accurate;
- quality.
Watch an interesting video about vector diagrams below:
Accurate ones are depicted based on the results of numerical calculations, provided that the scales of the actual values correspond. When constructing them, it is possible to geometrically determine the phases and amplitude values of the desired quantities.
Vasiliev Dmitry Petrovich
Professor of Electrical Engineering, St. Petersburg State Polytechnic University
Qualitative diagrams are depicted taking into account the mutual relationships between electrical quantities, without indicating numerical characteristics.
They are one of the main means of analyzing electrical circuits, allowing you to clearly illustrate and qualitatively control the progress of solving a problem and easily establish the quadrant in which the desired vector is located.
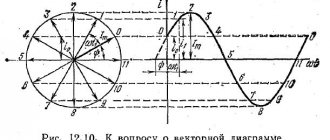
For convenience, when constructing diagrams, stationary vectors are analyzed for a certain point in time, which is selected in such a way that the diagram has a form that is easy to understand. The OX axis corresponds to the values of real numbers, the OY axis to the axis of imaginary numbers (imaginary unit). The sinusoid displays the movement of the end of the projection on the OY axis. Each voltage and current corresponds to an eigenvector on the plane in polar coordinates. Its length displays the amplitude value of the current, with the angle being equal to the phase.
The vectors depicted in such a diagram are characterized by an equal angular frequency ω. In view of this, when rotating, their relative position does not change.
Another useful video about vector diagrams:
Therefore, when depicting vector diagrams, one vector can be directed in any way (for example, along the OX axis).
And the rest should be depicted in relation to the original one at different angles, respectively equal to the phase shift angles.
Thus, the vector diagram gives a clear idea of the advance or lag of various electrical quantities.
Let's say we have a current, the value of which varies according to a certain law:
i = Im sin (ω t + φ).
From the origin of coordinates 0 at an angle φ we draw the vector Im, the value of which corresponds to Im. Its direction is chosen so that the vector makes an angle with the positive direction of the OX axis - corresponding to the phase φ.
Abrahamyan Evgeniy Pavlovich
Associate Professor, Department of Electrical Engineering, St. Petersburg State Polytechnic University
The projection of the vector onto the vertical axis determines the value of the instantaneous current at the initial moment of time.
Basically, vector diagrams are depicted for effective values, and not for amplitude values. Vectors of effective values differ quantitatively from amplitude values - in scale, because:
I = Im /√2.
The main advantage of vector diagrams is the ability to simply and quickly add and subtract 2 parameters when calculating electrical circuits.