Definition 1
In the vast majority of cases in electrical engineering, sinusoidal currents are used. Sinusoidal currents are currents whose instantaneous values change in accordance with the law:
\[I\left(t\right)=I_m{sin \left(\omega t- \Psi \right)\ \left(1\right).\ }\]
Such currents arise as a result of steady-state forced oscillations in the RLC circuit if it is acted upon by an alternating voltage of the form:
The current amplitude ($I_m$) is determined by the voltage amplitude as:
Expression:
called total electrical resistance or impedance.
Special cases of current amplitude values
In the event that the circuit consists only of active resistance $(R)$, then:
the current coincides with the voltage in phase, the amplitude of the current in this case is equal to:
Are you an expert in this subject area? We invite you to become the author of the Directory Working Conditions
If we compare equation (6) with expression (3), we can conclude that if, instead of a capacitor, a section of the circuit is simply short-circuited, this will mean a transition to a capacitance equal to infinity.
Let the resistance $(R=0)$ be neglected in the circuit, and the capacitance be considered equal to infinity, then:
The quantity $X_L$ is called inductive reactance (inductive reactance) if it is equal to:
From the formula it follows that the inductance does not resist direct current (at $\omega$=0, $X_L$=0).
Let us assume that $R=0,\ L=0.$ Then, according to formula (3), we obtain:
The quantity $X_C=\frac{1}{\omega C}$ is called reactance capacitance (capacitance) . If the current is constant, then $X_C=\infty $. This means that no direct current flows through the capacitor.
In the event that R=0, the amplitude of the current is equal to:
Reactance (reactance) $(X)$ is a value equal to:
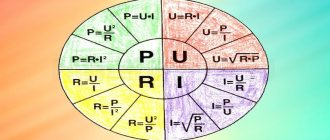
Basic parameters of sinusoidal current
LECTURE 2
SINUSOIDAL CURRENT. FORMS OF ITS REPRESENTATION.
In the practice of electrical engineering, sinusoidal current has found widespread use as alternating current. This is due to a number of advantages:
— sinusoidal current generators are much cheaper to produce than DC generators;
— alternating current is easily converted into direct current;
— transformation and transmission of electrical energy with alternating current is more economical than direct current;
— AC motors have a simple design, high reliability and low cost.
Currently, alternating current is used in industrial drives and electric lighting, in agriculture and transport, in communications technology and in everyday life. The production of electrical energy is also carried out using alternating current. Russian scientists P.N. Yablochkov and M.O. Dolivo-Dobrovolsky played a huge role in the introduction of alternating current.
Basic parameters of sinusoidal current
Variable current is a current (voltage, emf) that changes over time in magnitude and direction. A sinusoidal current can be represented by a real function of time - sine and cosine, for example:
(2.1)
where Im is the maximum current amplitude (amplitude value);
w is the angular frequency, and ;
f—oscillation frequency [Hz];
T—period [C];
ji is the initial phase, determines the current value at time t=0, i.e.
i(t=0) = Im× sin ji.
In Fig. Figure 2.1 shows a graph of two oscillations with different initial phases j1 and j2, and j1 > j2. The harmonic amplitude passes through zero when:
wt + j = pn (n = 0,1,2...), i.e. in moments
.
Since j1> j2, then t1 occurs before t2:
Fig.2.1
The initial phase is often specified in degrees. Therefore, when determining the instantaneous value of the current, the argument of the sine (the terms wt and j) must be reduced to one unit of measurement (rad. or degree).
Sometimes harmonic vibration is represented in cosine form. It is easy to see that to go to this form in (2.1) it is enough to change only the initial phase, i.e.:
The industrial frequency of alternating current in Russia and all European countries is 50 Hz, in the USA and Japan - 60 Hz, in aviation - 400 Hz. Reducing the frequency below 50 Hz degrades the lighting quality. An increase in frequency worsens the conditions for transmitting electricity over long distances.
The expression for sinusoidal voltage is similar to (2.1), i.e.:
u(t) = Um × sin (wt + ju) (2.2)
The main voltage parameters are determined similarly to (2.1).
In addition to the parameters already mentioned, in electrical engineering practice the concepts of average and effective values of current and voltage are often used. Let's look at them.
The average value of a sinusoidal current is understood as its average value over a half-cycle:
(2.3)
We see that the average value of the sinusoidal current is 2/p » 0.64 of the amplitude one. The average value of the sinusoidal voltage is determined similarly
.
The effective value is the mean square value of the sinusoidal current (voltage) over the period:
.
Because:
,
That:
.
We see that the effective value of the sinusoidal current is 0.707 of the amplitude one. The effective value of the sinusoidal voltage is determined similarly:
.
If they talk about the values of alternating current or voltage, then, as a rule, they mean their effective values. For example, the voltage in a single-phase AC network is 220 V effective. In this case, the amplitude value Um @ 310 V.
LECTURE 2
SINUSOIDAL CURRENT. FORMS OF ITS REPRESENTATION.
In the practice of electrical engineering, sinusoidal current has found widespread use as alternating current. This is due to a number of advantages:
— sinusoidal current generators are much cheaper to produce than DC generators;
— alternating current is easily converted into direct current;
— transformation and transmission of electrical energy with alternating current is more economical than direct current;
— AC motors have a simple design, high reliability and low cost.
Currently, alternating current is used in industrial drives and electric lighting, in agriculture and transport, in communications technology and in everyday life. The production of electrical energy is also carried out using alternating current. Russian scientists P.N. Yablochkov and M.O. Dolivo-Dobrovolsky played a huge role in the introduction of alternating current.
Basic parameters of sinusoidal current
Variable current is a current (voltage, emf) that changes over time in magnitude and direction. A sinusoidal current can be represented by a real function of time - sine and cosine, for example:
(2.1)
where Im is the maximum current amplitude (amplitude value);
w is the angular frequency, and ;
f—oscillation frequency [Hz];
T—period [C];
ji is the initial phase, determines the current value at time t=0, i.e.
i(t=0) = Im× sin ji.
In Fig. Figure 2.1 shows a graph of two oscillations with different initial phases j1 and j2, and j1 > j2. The harmonic amplitude passes through zero when:
wt + j = pn (n = 0,1,2...), i.e. in moments
.
Since j1> j2, then t1 occurs before t2:
Fig.2.1
The initial phase is often specified in degrees. Therefore, when determining the instantaneous value of the current, the argument of the sine (the terms wt and j) must be reduced to one unit of measurement (rad. or degree).
Sometimes harmonic vibration is represented in cosine form. It is easy to see that to go to this form in (2.1) it is enough to change only the initial phase, i.e.:
The industrial frequency of alternating current in Russia and all European countries is 50 Hz, in the USA and Japan - 60 Hz, in aviation - 400 Hz. Reducing the frequency below 50 Hz degrades the lighting quality. An increase in frequency worsens the conditions for transmitting electricity over long distances.
The expression for sinusoidal voltage is similar to (2.1), i.e.:
u(t) = Um × sin (wt + ju) (2.2)
The main voltage parameters are determined similarly to (2.1).
In addition to the parameters already mentioned, in electrical engineering practice the concepts of average and effective values of current and voltage are often used. Let's look at them.
The average value of a sinusoidal current is understood as its average value over a half-cycle:
(2.3)
We see that the average value of the sinusoidal current is 2/p » 0.64 of the amplitude one. The average value of the sinusoidal voltage is determined similarly
.
The effective value is the mean square value of the sinusoidal current (voltage) over the period:
.
Because:
,
That:
.
We see that the effective value of the sinusoidal current is 0.707 of the amplitude one. The effective value of the sinusoidal voltage is determined similarly:
.
If they talk about the values of alternating current or voltage, then, as a rule, they mean their effective values. For example, the voltage in a single-phase AC network is 220 V effective. In this case, the amplitude value Um @ 310 V.
AC networks
Based on their purpose and application, these networks can be classified as follows:
- general systems: power supply for industrial, transport, agricultural and domestic facilities;
- autonomous networks: supplying mobile and stationary autonomous entities.
General three-phase alternating current networks are built according to a four-wire circuit, where three wires are “phase” and the fourth is “zero”. Transformer substations are built according to a scheme with a solidly grounded neutral. Long-distance transmission is carried out at high voltage, which is then reduced at substations to a voltage of 0.4 kV and distributed to consumers.
Household objects are connected using a single-phase circuit. In this case, two wires are required: “phase” and “neutral”.
Complex method for calculating sinusoidal electrical modes. chains.
Sinusoidal mode el. chains
This is a mode in which all voltages and currents in the circuit change according to a sinusoidal law with the same frequency.
Sinusoidal voltages and currents are widely used mainly for the following reasons:
1. They are easily obtained using various generators.
2. They are easily converted by transformers.
3. With their help, rotating and traveling magnetic fields used in electric motors are easily created.
4. By adding sinusoidal oscillations, you can obtain various non-sinusoidal voltages and currents.
| Rice. 11.1. |
Consider a sinusoidal voltage. It is characterized by three parameters: amplitude
,
circular frequency
and
initial phase
(Fig. 11.1).
Amplitude values in electrical engineering are denoted by capital letters with the index m
.
The characteristics of a sinusoid also include the effective value of U
,
cyclic frequency
(i.e. the number of oscillations per second), and
period .
Sinusoidal current is characterized by similar parameters.
For any sinusoid, the effective value and amplitude are related by the coefficient:.
Electrical status circuits in sinusoidal modes can be described using time functions. However, this is cumbersome and time-consuming. Therefore, a complex method is used to calculate sinusoidal modes. It allows you to replace the differential and integral equations of electrical elements. circuits are algebraic, and also very clearly represent sinusoids in the form of vectors on vector diagrams.
The basis of the method is that each sinusoid is associated with a complex number, called a complex
. This correspondence is one-to-one. It is determined by the rule:
,
where is the effective value of the sinusoid, y is the initial phase of the sinusoid, is the imaginary unit (in electrical engineering it is denoted by this letter). Information about frequency is not included in the complex and must be taken into account separately. Complexes are designated in capital letters with a dot: , or underlined capital letters: .
Examples: ,
.
General scheme of the method:
1. Transition from sinusoids to complexes.
2. Solving the problem in complexes.
3. Transition from complexes to sinusoids (if necessary).
Let us consider arbitrary sinusoids and , their complexes and , as well as an arbitrary real number A
. Operations on a set of sinusoids and operations on a set of complexes have the following correspondence:
| These two properties are called linearity |
This correspondence of operations allows us to consider the set of sinusoids and the set of complex numbers as essentially the same mathematical object. The proof is simple and relies on the properties of sinusoids and complex numbers.
Complexes are represented by vectors on the plane according to the usual rules adopted for complex numbers. In electrical engineering, such drawings are called vector diagrams.
The arrows on the vector diagram are images of sinusoids, and the arrows on electrical diagrams. circuits are the directions for calculating voltages and currents!
Kirchhoff's laws, as well as all other properties and methods of calculating linear electrical systems. chains are preserved during the transition to complexes .
This is a consequence of the linearity of the correspondence between sinusoids and complexes.
Note 1: As modules of the complexes, we took the effective values of the sinusoids: . Such complexes are called effective value complexes
.
However, sometimes it is convenient to take the amplitude values of sinusoids as modules of complexes: . Such complexes are called complex amplitudes.
Note 2: Any sinusoid can also be represented in the form of sine and cosine components:
,
Where , . Wherein , .
Since for complex amplitudes , , the representation of a sine wave in the form of sine and cosine components allows us to associate it with a complex amplitude in algebraic form:
.
Note 3: The complex method is used not only in electrical engineering, but wherever sinusoidal oscillations are studied.
Sinusoidal mode el. chains
This is a mode in which all voltages and currents in the circuit change according to a sinusoidal law with the same frequency.
Sinusoidal voltages and currents are widely used mainly for the following reasons:
1. They are easily obtained using various generators.
2. They are easily converted by transformers.
3. With their help, rotating and traveling magnetic fields used in electric motors are easily created.
4. By adding sinusoidal oscillations, you can obtain various non-sinusoidal voltages and currents.
| Rice. 11.1. |
Consider a sinusoidal voltage. It is characterized by three parameters: amplitude
,
circular frequency
and
initial phase
(Fig. 11.1).
Amplitude values in electrical engineering are denoted by capital letters with the index m
.
The characteristics of a sinusoid also include the effective value of U
,
cyclic frequency
(i.e. the number of oscillations per second), and
period .
Sinusoidal current is characterized by similar parameters.
For any sinusoid, the effective value and amplitude are related by the coefficient:.
Electrical status circuits in sinusoidal modes can be described using time functions. However, this is cumbersome and time-consuming. Therefore, a complex method is used to calculate sinusoidal modes. It allows you to replace the differential and integral equations of electrical elements. circuits are algebraic, and also very clearly represent sinusoids in the form of vectors on vector diagrams.
The basis of the method is that each sinusoid is associated with a complex number, called a complex
. This correspondence is one-to-one. It is determined by the rule:
,
where is the effective value of the sinusoid, y is the initial phase of the sinusoid, is the imaginary unit (in electrical engineering it is denoted by this letter). Information about frequency is not included in the complex and must be taken into account separately. Complexes are designated in capital letters with a dot: , or underlined capital letters: .
Examples: ,
.
General scheme of the method:
1. Transition from sinusoids to complexes.
2. Solving the problem in complexes.
3. Transition from complexes to sinusoids (if necessary).
Let us consider arbitrary sinusoids and , their complexes and , as well as an arbitrary real number A
. Operations on a set of sinusoids and operations on a set of complexes have the following correspondence:
| These two properties are called linearity |
This correspondence of operations allows us to consider the set of sinusoids and the set of complex numbers as essentially the same mathematical object. The proof is simple and relies on the properties of sinusoids and complex numbers.
Complexes are represented by vectors on the plane according to the usual rules adopted for complex numbers. In electrical engineering, such drawings are called vector diagrams.
The arrows on the vector diagram are images of sinusoids, and the arrows on electrical diagrams. circuits are the directions for calculating voltages and currents!
Kirchhoff's laws, as well as all other properties and methods of calculating linear electrical systems. chains are preserved during the transition to complexes .
This is a consequence of the linearity of the correspondence between sinusoids and complexes.
Note 1: As modules of the complexes, we took the effective values of the sinusoids: . Such complexes are called effective value complexes
.
However, sometimes it is convenient to take the amplitude values of sinusoids as modules of complexes: . Such complexes are called complex amplitudes.
Note 2: Any sinusoid can also be represented in the form of sine and cosine components:
,
Where , . Wherein , .
Since for complex amplitudes , , the representation of a sine wave in the form of sine and cosine components allows us to associate it with a complex amplitude in algebraic form:
.
Note 3: The complex method is used not only in electrical engineering, but wherever sinusoidal oscillations are studied.
General concept of alternating current
Unlike electrons constantly moving in one direction, alternating current changes both direction and value several times per unit of time. Changes occur according to a harmonic law. If you observe a similar signal using an oscilloscope, you can see a picture in the form of a sine wave.
Relative to the ordinate axis OY, the current changes its direction from positive to negative and does this periodically. Therefore, its instantaneous value in the first position is considered positive, in the second - negative.
Important! Since alternating current is an algebraic quantity, we can talk about its sign of charge only for a specific instantaneous value, depending on the direction in which it flows at that moment.
Signal on the oscilloscope screen
AC generation
In addition to standard generators, inverters and phase splitters are used to produce alternating current.
Inverter
This is a device with the help of which direct current is converted into alternating current. During this process, the output voltage also changes. The device circuit is an electronic generator of a sinusoidal pulse voltage of a periodic nature. There are options for inverters that operate with a discrete signal. Inverters are used for autonomous power supply of equipment from constant voltage batteries.
Inverter 12/220 V, power 1500 W
Phase splitter
Another way to get multiple phases from a signal is to split it into several phases. This is done using a phase splitter. Forced processing of digital or analog signals is used both in radio electronics and power electrical engineering.
To supply power to three-phase asynchronous motors, a phase splitter based on them is used. To do this, the windings of a three-phase motor are connected not by a star, but in a different way. Two coils are connected to each other in series, the third is connected to the midpoint of the second winding. The motor is started as a single-phase one, after acceleration, an EMF is induced in its third winding.
Interesting. In the case of phase splitting using this method, the phase shift between windings 2 and 3 is not 1200, as it should ideally be, but 900.