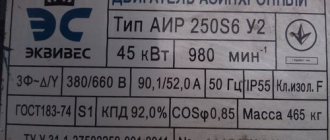
Electricity
- Electric current
is the directional (ordered) movement of charged particles.
Electric current in conductors is:
in metals
— directed movement of electrons (conductors of the first kind);
in electrolytes
— directed movement of positive and negative ions (conductors of the second kind);
in plasma
- directional movement of electrons and ions of both signs (conductors of the third kind).
For the direction of electric current
agreed to calculate the direction of movement
positively charged
particles.
The movement of charged particles inside a conductor cannot be observed, but the presence of an electric current can be judged by its actions:
- thermal
- the current-carrying conductor heats up; - magnetic
- a magnetic field appears around a current-carrying conductor; - luminous
- a conductor carrying current can glow; - chemical
- the chemical composition in a conductor carrying current changes (such conductors are called second-class conductors).
For the continued existence of electric current in a closed circuit, the following conditions must be met:
- the presence of free charged particles (current carriers);
- the presence of an electric field, the forces of which, acting on charged particles, cause them to move in an orderly manner;
- the presence of a current source within which external forces move free charges against electrostatic (Coulomb) forces.
The quantitative characteristics of electric current are current strength I
and current density
j
.
- Current strength
is a scalar physical quantity equal to the ratio of the charge Δ
q
passing through the cross section of the conductor over a certain period of time Δ
t
to this interval:
\(~I= \dfrac{\Delta q}{\Delta t}.\)
The SI unit of current is the ampere (A).
If the current strength and its direction do not change over time, then the current is called constant
.
- Current density j
is a vector physical quantity, the modulus of which is equal to the ratio of the current
I
in the conductor to the cross-sectional area
S
of the conductor:
$$~j = \frac {I}{S}.$$
The SI unit of current density is ampere per square meter (A/m2).
*Dependence of current strength on charge speed
Let us consider how the current strength depends on the speed of the ordered movement of free charges.
Let us select a section of the conductor with cross-sectional area S
and length Δ
l
(Fig. 1).
The charge of each particle is q
0. The volume of the conductor, limited by sections
1
and
2
, contains
n∙S
∙Δ
l
particles, where
n
is the concentration of particles. Their total charge is \(~\Delta q = q_0 \cdot n \cdot S \cdot \Delta l\).
Rice. 1
If the average speed of the ordered movement of free charges is \(~\left\langle \upsilon \right\rangle\), then over a period of time \(~\Delta t = \dfrac{\Delta l}{\left\langle \upsilon \right \rangle}\) all particles contained in the volume under consideration will pass through the section 2
.
Therefore, the current strength: \(~I = \dfrac{\Delta q}{\Delta t} = \dfrac{q_0 \cdot n \cdot \left\langle \upsilon \right\rangle \cdot S \cdot \Delta l} {\Delta l} = q_0 \cdot n \cdot \left\langle \upsilon \right\rangle \cdot S. \qquad (1)\)
Thus, the current strength in the conductor depends on the charge carried by one particle, their concentration , the average speed of directional movement of particles and the cross-sectional area of the conductor.
Note that in metals the magnitude of the vector of the average speed of the ordered movement of electrons \(~\left\langle \upsilon \right\rangle\) at the maximum permissible current values is ~ 10-4 m/s, while the average speed of their thermal movement ~ 106 m/s.
As follows from formula (1), the current density is \(~\vec j = q_0 \cdot n \cdot \left\langle \vec \upsilon \right\rangle\).
- The direction of the current density vector \(~\vec j\) coincides with the direction of the speed vector of ordered motion \(~\left\langle \vec \upsilon \right\rangle\) of positively charged particles. The DC current density is constant over the entire cross-section of the conductor.
Premium
The entire part 2 of the Unified State Examination in mathematics, from task 13 to task 19. What even your tutors don’t tell you about. All techniques for solving problems in part 2. Formatting problems for the exam. Dozens of real Unified State Exam problems, from simple to the most complex.
The Premium video course consists of 7 courses for mastering Part 2 of the Unified State Exam in mathematics (problems 13-19). The duration of each course is from 3.5 to 4.5 hours.
- Equations (problem 13)
- Stereometry (problem 14)
- Inequalities (problem 15)
- Geometry (problem 16)
- Financial mathematics (problem 17)
- Parameters (task 18)
- Non-standard problem on numbers and their properties (problem 19).
Here is something that is not in textbooks. What they won't tell you at school. Techniques, methods and secrets for solving problems of part 2.
Each topic is analyzed from scratch. Dozens of specially selected tasks, each of which helps to understand the pitfalls and tricks of the solution. The author of the Premium video course is professional tutor Anna Malkova.
Current source
To maintain electric current in the circuit, it is necessary that at its ends (Fig. 2) there is a constant potential difference φ1 – φ2. Let at the initial moment of time φ1 > φ2, then the transfer of positive charge q
from the source terminal “+” to the “–” terminal will lead to a decrease in the potential difference between them.
To maintain a constant potential difference, it is necessary to transfer exactly the same charge from the “–” terminal to the “+” terminal. If in the direction from “+” to “–” positive charges move under the influence of Coulomb forces Fk
, then in the direction from “–” to “+” the movement of charges occurs against the direction of action of Coulomb forces, i.e.
under the influence of another force F
st, which is called an external force.
Rice. 2
- Third-party forces
are any forces acting on electrically charged particles, with the exception of electrostatic (Coulomb) forces.
External forces arise in the current source.
- A current source
is a device capable of maintaining a potential difference between the ends of an electrical circuit and ensuring the orderly movement of electrical charges in an external circuit.
Sources of electric current may vary in design, but in any of them work is done to separate positively and negatively charged particles. The separation of charges occurs under the influence of external forces. We list the most common current sources:
- galvanic cells
(batteries) (Fig. 3, a) and
accumulators
- third-party forces use the energy of chemical reactions; - generators
(dynamos) - third-party forces use the mechanical energy of falling water, wind, steam, etc.; - photocells
(solar batteries) (Fig. 3, b) - external forces use the energy of electromagnetic radiation (light).
- A
- b
Rice.
3 The source of electric current has two poles (two terminals) to which the ends of the wires are connected.
The conductor connecting the terminals of the source from the outside is called the external section of the circuit
.
The resistance of this source is denoted by R
and is called
external resistance
.
Inside the source itself, charges move along the internal section of the circuit
.
The source resistance is denoted by r
and is called
internal resistance
.
The sum of external and internal resistances ( R + r
) is called
the total resistance of the circuit
.
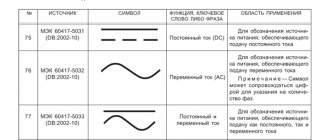
On electrical diagrams, the current source is designated as shown in Fig. 4. The positive pole (terminal) of the source is conventionally represented by a longer line than the negative one.
Rice. 4
Any current source is characterized by electromotive force - EMF.
- EMF (Electromotive force) ε of a current source is a physical scalar quantity that is numerically equal to the work of external forces Ast
to move a single positive charge inside the current source:
\(~\varepsilon = \dfrac{A_{st}}{q} .\)
The SI unit of electromotive force is the volt (V).
EMF is an energy characteristic of a current source.
- The term “ electric motor force
” was introduced by Ampere in 1822. The abbreviation EMF is usually read without decoding.
see also
All about chemical current sources
Energy transformations in an oscillatory circuit
We continue to consider undamped oscillations in the circuit, considering the coil resistance to be zero. The capacitor has a capacitance and the inductance of the coil is equal to .
Since there are no heat losses, energy does not leave the circuit: it is constantly redistributed between the capacitor and the coil.
Let's take a moment in time when the charge of the capacitor is maximum and equal to , and there is no current. The energy of the magnetic field of the coil at this moment is zero. All the energy of the circuit is concentrated in the capacitor:
Now, on the contrary, let’s consider the moment when the current is maximum and equal to , and the capacitor is discharged. The energy of the capacitor is zero. All the circuit energy is stored in the coil:
At an arbitrary moment in time, when the charge of the capacitor is equal and current flows through the coil, the energy of the circuit is equal to:
Relationship (1) is used to solve many problems.
Ohm's law for a closed circuit
Let's consider the simplest complete electrical circuit containing a source of emf ε with internal resistance r
R
connected to them (Fig. 5).
Rice.
5 Then
\(~I = \dfrac{\varepsilon}{R + r} . \qquad (2)\)
- This formula represents Ohm's law for a complete circuit
: The current in a complete circuit is directly proportional to the source emf and inversely proportional to the total resistance of the circuit.
Note that the maximum possible current in a circuit with a given current source occurs if the resistance of the external circuit tends to zero.
Connecting a conductor with negligible resistance to the poles of a current source is called a short circuit
, and the maximum current for a given source is called
short circuit current
:
\(~I_{kz} = \dfrac{\varepsilon}{r} .\)
For sources with a small value of r
(for example, for lead batteries
r
= 0.1 - 0.01 Ohm), the short circuit current is very high.
A short circuit in lighting networks powered from substations ( ε
> 100 V) is especially dangerous;
I
kz can reach thousands of amperes. To avoid fires, fuses are included in such circuits.
*Derivation of Ohm's law
Let's consider the simplest complete electrical circuit containing a source of emf ε with internal resistance r
R
connected to them (see Fig. 5).
From the definitions of current strength and EMF of a current source it follows that the work performed by the source
\(A_{st} =\varepsilon \cdot \Delta q=\varepsilon \cdot I\cdot \Delta t.\)
When current passes, the conductors heat up, and energy is released in both the external circuit Q1 and the internal circuit Q2. Then the amount of heat Q released in the entire complete chain is equal to the sum of these energies. According to the Joule-Lenz law
\(Q=Q_{1} +Q_{2} =I^{2} \cdot R\cdot \Delta t+I^{2} \cdot r\cdot \Delta t=I^{2} \cdot \ left(R+r\right)\cdot \Delta t.\)
From the law of conservation of energy we find that in such a circuit the work of external forces over a period of time Δt is equal to the amount of heat released in the circuit:
\(\begin{array}{c} {A_{st} =Q,\; \; \; \varepsilon \cdot I\cdot \Delta t=I^{2} \cdot \left(R+r\right )\cdot \Delta t,} \\ {\varepsilon =I\cdot \left(R+r\right),\; \; \; I=\dfrac{\varepsilon }{R+r} .} \end {array}\)
Get an A
The video course “Get an A” includes all the topics necessary to successfully pass the Unified State Exam in mathematics with 60-65 points. Completely all tasks 1-13 of the Profile Unified State Exam in mathematics. Also suitable for passing the Basic Unified State Examination in mathematics. If you want to pass the Unified State Exam with 90-100 points, you need to solve part 1 in 30 minutes and without mistakes!
Preparation course for the Unified State Exam for grades 10-11, as well as for teachers. Everything you need to solve Part 1 of the Unified State Exam in mathematics (the first 12 problems) and Problem 13 (trigonometry). And this is more than 70 points on the Unified State Exam, and neither a 100-point student nor a humanities student can do without them.
All the necessary theory. Quick solutions, pitfalls and secrets of the Unified State Exam. All current tasks of part 1 from the FIPI Task Bank have been analyzed. The course fully complies with the requirements of the Unified State Exam 2018.
The course contains 5 large topics, 2.5 hours each. Each topic is given from scratch, simply and clearly.
Hundreds of Unified State Exam tasks. Word problems and probability theory. Simple and easy to remember algorithms for solving problems. Geometry. Theory, reference material, analysis of all types of Unified State Examination tasks. Stereometry. Tricky solutions, useful cheat sheets, development of spatial imagination. Trigonometry from scratch to problem 13. Understanding instead of cramming. Clear explanations of complex concepts. Algebra. Roots, powers and logarithms, function and derivative. A basis for solving complex problems of Part 2 of the Unified State Exam.
Immediately after payment you will receive links to download video courses and unique keys to them.
The tasks of the “Mathematical Trainings - 2019” set are not easy. Each contains interesting tricks, pitfalls, and useful secrets.
The options are designed to cover all possible complex problems of both the first and second parts of the Unified State Examination in mathematics.
Current source efficiency
For closed circuit, power Pp
, allocated in the external section of the circuit, is called
useful power
.
It is equal to \(~P_p = I^2 \cdot R\) .
Taking into account Ohm's law for the circuit section \(~I = \dfrac{U}{R}\), the useful power can be found if any two of the three quantities are known: I
,
U
,
R
.
\(~P_p = U \cdot I\) , \(~P_p = I^2 \cdot R\) , \(~P_p = \dfrac{U^2}{R}\) .
For closed circuit, power Pt
, released at the internal resistance of the source, is called
lost power
.
It is equal to \(~P_t = I^2 \cdot r\) .
The total power
P
of the current source is equal to
\(~P = P_p + P_t = I^2 \cdot R + I^2 \cdot r = I^2 \cdot \left( R + r \right). \)
Efficiency of the current source
\(~\eta = \dfrac{P_p}{P}= \dfrac{I^2 \cdot R}{I^2 \cdot \left( R + r \right)} = \dfrac{R}{R + r}\).
How to use?
- There is no need to immediately review the problems (and solutions) of all options. Such cheating will only hinder you. Take one at a time! Solve problems one at a time and try to get to the answer.
- If almost nothing works out, you need to start not with solving the options, but with studying mathematics. A book for preparing for the Unified State Examination and an Annual Online Course will help you.
- If you correctly solved 5-7 problems from the first version of Matttraining, it means that you lack knowledge. See point 1: Books and Annual Online Course!
- Be sure to figure out the right solutions. Watch the video analysis - it also has a lot of useful information.
- You can decide on your own or with friends. Or the whole class. And then watch a video analysis of the option.
The cost of the “Mathematical Trainings – 2019” set is only 1,100 rubles. For 5 options with solutions and video analysis of each.
This is a trial version of an online course on specialized mathematics.
You will get access to 3 topics that will help you understand the principle of training, the operation of the platform and evaluate the course leader Anna Malkova.
— 3 course topics (out of 50). — Text textbook with video examples. — Master class by Anna Malkova. — A simulator for practicing tasks.
Register, it's free!
By clicking on the button, you consent to the processing of your personal data
Literature
- Aksenovich L. A. Physics in secondary school: Theory. Tasks. Tests: Textbook. allowance for institutions providing general education. environment, education / L. A. Aksenovich, N. N. Rakina, K. S. Farino; Ed. K. S. Farino. - Mn.: Adukatsiya i vyakhavanne, 2004. - P. 252-253, 259-260, 262-264, 267-269.
- Zhilko, V.V. Physics: textbook. allowance for 11th grade. general education institutions with Russian language training with a 12-year period of study (basic and advanced levels) /V. V. Zhilko, L. G. Markovich. — 2nd ed., revised. — Minsk: Nar. Asveta, 2008. - pp. 118-123, 132-141.