AC circuit impedance

Electrical circuit.

A source of electric current connected by wires to various electrical appliances and consumers of electrical energy forms an electrical circuit.

It is customary to depict an electrical circuit using diagrams in which the elements of the electrical circuit (resistances, current sources, switches, lamps, devices, etc.) are indicated with special icons.

The direction of current in a circuit is the direction from the positive pole of the current source to the negative pole. This rule was established in the 19th century. and has been observed ever since. The movement of real charges may not coincide with the conventional direction of the current. Thus, in metals, current carriers are negatively charged electrons, and they move from the negative pole to the positive one, that is, in the opposite direction. In electrolytes, the actual movement of charges may coincide with or be opposite to the direction of the current, depending on whether the ions are charge carriers - positive or negative.

The inclusion of elements in an electrical circuit can be serial or parallel.

What is resistance

Current flowing through wires and various radio components wastes its energy. This phenomenon is quantitatively expressed by the magnitude of the resistance. In electrical engineering, it is divided into active and reactive resistance. In the first case, when a current passes, part of its energy is converted into a thermal form, and sometimes into others (for example, it manifests itself in chemical reactions). The value of active resistance depends on the frequency of alternating electric current and increases with its increase.

The second type of resistance has a more complex nature and occurs when a consumer of electricity is turned on or off in an AC or DC network. In a circuit with reactance, the energy of the electric current is partially converted into another form, and then transferred back, that is, a periodic oscillatory process is observed. Circuit impedance includes active and reactive types, which are taken into account according to special rules.

Calculation

Having found out what formula is used to make the necessary calculations and understanding the meaning of capacitance, you can start calculating this value.

For example, let's make a calculation based on the following data:

Capacitance of the capacitor C=1uF;
The circuit also has an active resistance R, which is equal to 5 kOhm;
The inductive reactance of the xL circuit is 4.5 kOhm;
AC frequency is 50 Hz;
Voltage 50 volts.

Based on these data, it will be necessary to find the resistance of the capacitor.

We define capacitance as follows:

xC=1/(2πfC)=1/(2×3.14×50×1×10-6)=3184 Ohm or rounded 3.2 kOhm.

To determine the current value in this circuit, we use Ohm’s law:

I=U/xC=50/3184=0.0157 ampere or 15.7mA.

After this, the parameters of the total resistance are determined:

Z=(R²+(xL-xC)²)½=(5000²+(4500–3184)²)½=5170 Ohm or 5.1 kOhm.

According to these calculations, it is possible to determine the influence of the capacitive element on the electrical circuit. The main thing is to understand what physical quantities are used in these formulas to perform correct calculations.

Types of resistance

In electrical engineering, active electrical resistance is considered, as well as two types of reactive resistance: inductive and capacitive.

Active resistance You can imagine an electrical circuit in which a resistor and a light bulb are connected in series to the battery terminals through a wire. If you short the wires, the light will light up. You can use a voltmeter or multimeter in the appropriate operating mode, which measures the potential difference between two points in the circuit.

By measuring the voltage between the terminals and comparing it with that on the wires connected to the light bulb, you can see that the latter is less. This is due to a voltage drop across the radio component soldered into the circuit. The latter counteracts the electric current, making it difficult to pass.

Each part through which current passes has active resistance. For metal wires it is very small. To find out the resistance value of a radio component, you need to study the designation on its body. If a resistor is removed from the electrical circuit in question, the current passing through the light bulb will increase.

The formula for calculating active resistance corresponds to Ohm's law:

R = U / I, where

R is the value of active resistance between two points in the circuit;
U is the voltage or potential difference between them;
I is the current strength in the section of the circuit under consideration.

To calculate the active resistance of the conductor, the formula will be different:

where K is the surface effect coefficient, which is equal to 1,

l is the length of the conductor,
s is the cross-sectional area,
p - “po” resistivity.

Resistance is usually measured in Ohms. It significantly depends on the shape and size of the object through which the current flows: cross-section, length, material, as well as temperature. The action of active resistance reduces the energy of electric current, converting it into other forms (mainly heat).

Reactance

This type occurs when alternating current passes through an element that has inductance or capacitance. The main feature of reactance is the conversion of electrical energy into another form in the forward and reverse directions. This often happens cyclically. Reactance only appears when current and voltage change. There are two types: inductive and capacitive.

Inductive reactance

As the current increases, a magnetic field with different characteristics is generated. The most important of these is inductance. The magnetic field, in turn, affects the conductor through which current flows. The effect is opposite to the direction of current change. That is, if the current strength has increased, then the magnetic field will reduce it, and vice versa, if it has decreased, then the field will strengthen it. When the current does not change, the reactance of the inductor will be zero.

Inductive reactance depends on the frequency of the current. The higher it is, the higher the rate of change of this parameter. This means that a stronger magnetic field will be generated. The resulting EMF prevents a change in the electric current.

Impedance

When using several varieties, it is important to know how they fit together. Active resistance is present in any circuit. It helps convert part of the electrical energy into heat. Reactance occurs only in an alternating current circuit. To determine its value, it is necessary to subtract capacitive from inductive. This characteristic shows the energy that pulsates in a circuit, moving from one form to another.

Impedance is the sum of active and reactance in an AC circuit, but this addition must be done in a special way. To do this, you need to draw a right triangle, the legs of which should have a length equal to the value of active and reactive resistance, respectively.

It will be interesting➡ Determining the direction of the magnetic induction vector

The length of the hypotenuse will numerically express the total resistance of the electrical circuit. To determine it, a rule is used that says that the sum of the squares of the legs is equal to the square of the hypotenuse. This rule is called the Pythagorean theorem. Therefore, the formula that can be used to find the total resistance is:

Z = √(R^2+〖(XL-XC)〗^2 ), where

Z—impedance;
R is the value of the active component;
XL and XC are the values of the inductive and capacitive parameters, respectively.

Therefore, when calculating impedance or impedance, you need to consider what capacitance and inductance are and how they may appear in electrical circuits. These quantities are also called parasitic, since they can negatively affect the operation of the electrical appliance. Their occurrence is attributed to unpredictable factors. In this case, capacitive or inductive reactance, which has a small value, can be neglected when performing calculations.

Measurement

Measuring the impedance of devices and transmission lines is a practical task in radio technology and other fields. Impedance measurements can be made at a single frequency, or the change in device impedance over a range of frequencies may be of interest. Impedance may be measured or displayed directly in ohms, or other impedance-related values may be displayed; for example, in a radio antenna, the standing wave ratio or reflection coefficient may be more useful than impedance alone. Impedance measurements require measuring the magnitude of voltage and current, as well as the phase difference between them. Impedance is often measured by "bridge" methods, similar to a DC Wheatstone bridge; The calibrated reference impedance is adjusted to compensate for the effect of the device under test impedance. Measuring impedance in power electronic devices may require simultaneous measurement and application of power to the operating device.

The impedance of a device can be calculated by a complex division of voltage and current. The impedance of a device can be calculated by applying a sine wave voltage to the device in series with a resistor and measuring the voltage across the resistor and across the device. Making this measurement by sweeping the frequencies of the applied signal provides the phase and magnitude of the impedance.[12]

The use of impulse response can be used in conjunction with fast Fourier transform (FFT) to quickly measure the electrical resistance of various electrical devices.[12]

An LCR (Inductance (L), Capacitance (C), and Resistance (R)) meter is a device commonly used to measure the inductance, resistance, and capacitance of a component; From these values, the impedance at any frequency can be calculated.

example

Consider an LC tank circuit. The complex impedance of the circuit is

Z ( ω ) = j ω L 1 − ω 2 LC . { displaystyle Z ( omega) = { j omega L over 1- omega ^ {2} LC}.}

It is immediately clear that the value 1 | Z | { displaystyle {1 over | Z |}} is minimal (actually equal to 0 in this case) whenever

ω 2 LC = 1. { displaystyle omega ^ {2} LC = 1.}

Therefore, the angular frequency of the main resonance is equal to

ω = 1 LC. { displaystyle omega = {1 over { sqrt {LC))}.}

The total resistance of a circuit with a series connection of active and reactive resistance.

In any section of the circuit shown in Figure 2a, the instantaneous current values must be the same, since otherwise accumulations and rarefaction of electrons would be observed at some points in the circuit. In other words, the phases of the current along the entire length of the circuit must be the same. In addition, we know that the voltage phase across the inductive reactance leads the current phase by 90°, and the voltage phase across the active resistance coincides with the current phase (Figure 2b). It follows that the radius vector of voltage UL (voltage across inductive reactance) and voltage UR (voltage across active resistance) are shifted relative to each other by an angle of 90°.

Figure 2. Impedance of a circuit with active resistance and inductance. a) – circuit diagram; b) – phase shift of current and voltage; c) – stress triangle; e) – resistance triangle.

To obtain the radius vector of the resulting voltage at terminals A and B (Fig. 2,a), we will perform a geometric addition of the radius vectors UL and UR. This addition is performed in Fig. 2,c, from which it is clear that the resulting vector UAB is the hypotenuse of a right triangle.

From geometry it is known that the square of the hypotenuse is equal to the sum of the squares of the legs.

According to Ohm's law, voltage must equal current times resistance.

Since the current strength at all points of the circuit is the same, the square of the total resistance of the circuit (Z2) will also be equal to the sum of the squares of the active and inductive resistance, i.e.

(1)

Taking the square root of both sides of this equality, we get,

(2)

Thus, the total resistance of the circuit shown in Fig. 2a is equal to the square root of the sum of the squares of the active and inductive resistance

The total resistance can be found not only by calculation, but also by constructing a resistance triangle, similar to the voltage triangle (Figure 2,e), i.e. the total resistance of the circuit to alternating current can be obtained by measuring the hypotenuse, a right triangle, the legs of which are active and reactance. Of course, measurements of the legs and hypotenuse must be made on the same scale. So, for example, if we agreed that 1 cm of the length of the legs corresponds to 1 ohm, then the number of ohms of total resistance will be equal to the number of centimeters that fit on the hypotenuse.

The total resistance of the circuit shown in Fig. 2a is neither purely active nor purely reactive; it contains both of these types of resistance. Therefore, the phase angle of current and voltage in this circuit will differ from both 0° and 90°, that is, it will be greater than 0°, but less than 90°. Which of these two values it is closer to will depend on which of these resistances is dominant in the circuit. If the inductive reactance is greater than the active one, then the phase angle will be closer to 90°, and vice versa, if the active resistance is predominant, then the phase angle will be closer to 0°.

In the circuit shown in Fig. 3a, active and capacitive resistances are connected in series. The total resistance of such a circuit can be determined using a resistance triangle in the same way as we determined above the total resistance of an active-inductive circuit.

Figure 3. Circuit impedance with resistive resistance and capacitance. a) – circuit diagram; b) – resistance triangle.

The only difference between both cases is that the resistance triangle for the active-capacitive circuit will be turned in the other direction (Figure 3, b) due to the fact that the current in the capacitive circuit does not lag behind the voltage, but leads it.

For this case:

(3)

In the general case, when a circuit contains all three types of resistance (Fig. 4a), the reactance of this circuit is first determined, and then the total resistance of the circuit.

Figure 4. Impedance of a circuit containing R, L and C. a) – circuit diagram; b) – resistance triangle.

The reactance of this circuit consists of inductive and capacitive reactance. Since these two types of reactance are opposite in nature, the total reactance of the circuit will be equal to their difference, i.e.

(4)

The total reactance of the circuit can be inductive or capacitive, depending on which of these two resistances (XL or XC) predominates.

After we have determined the total reactance of the circuit using formula (4), determining the total resistance will not present any difficulties. The total resistance will be equal to the square root of the sum of the squares of the active and reactive resistance, i.e.

(5)

(6)

The method for constructing a resistance triangle for this case is shown in Fig. 4 b.

The total resistance of a circuit with a parallel connection of active and reactive resistance.

Concept of current and voltage

The scientist’s rule establishes the relationship between three electrical quantities: current, voltage and resistance. Therefore, in order to understand the essence of Ohm's law for a complete electrical circuit, it is necessary to understand what they are.

In any body there are free elementary particles that have a certain amount of energy - charge. If the body is in a calm state, that is, there is no impact on it, then their chaotic movement occurs. If an electric field is applied to the body, then their movement becomes ordered, and they begin to move in one direction.

This directed movement is called electric current. Its measure is the current strength, a scalar quantity determined by the ratio of the number of charges passing through the cross section of the conductor per unit time: I = dq/dt. The unit for measuring current is the ampere.

If the direction of movement of charges remains unchanged, then the movement of current is considered constant, and if it changes, it is considered variable. Current can only occur in a closed circuit. In order for the charge to move, the applied field must do work. That is, to expend some energy to move a charge from one point to another. If we assume that in the initial position the particle has zero charge, then having moved, it will already have a different value. The difference between these quantities is called potential difference or voltage.

To maintain current strength in a complete circuit, a source is required that constantly acts on free charges and maintains potential differences in different parts of the circuit. The amount of force that acts on the circuit is called emf. Physically, it represents the ratio of the work spent on moving a charge from one pole to another to the value of the charge: E = A/q. EMF, like voltage, is measured in volts.

When a charge moves, due to the structural features of the crystal lattice of a substance, it encounters various defects and impurities. As a result of this, its potential is partially dissipated, and the speed of movement slows down. Energy loss is characterized by an electrical quantity called resistance. In other words, resistance is the amount that prevents the flow of current.

The total resistance of the circuit when the active and reactive elements are connected in parallel.

In order to calculate the total resistance of a circuit composed of active and inductive resistances connected to each other in parallel (Fig. 5, a), you must first calculate the conductivity of each of the parallel branches, then determine the total conductivity of the entire circuit between points A and B and then calculate the total resistance of the circuit between these points.

Figure 5. Circuit impedance when connecting active and reactive elements in parallel. a) – parallel connection of R and L; b) – parallel connection of R and C.

The conductivity of the active branch, as is known, is equal to 1/R, similarly, the conductivity of the inductive branch is equal to 1/ωL, and the total conductance is equal to 1/Z

Total conductivity is equal to the square root of the sum of the squares of active and reactive conductivity, i.e.

(7)

Reducing the radical expression to a common denominator, we get:

(8)

where:

(9)

Formula (9) is used to calculate the total resistance of the circuit shown in Fig. 5a.

Finding the total resistance for this case can also be done geometrically. To do this, you need to construct a resistance triangle on the appropriate scale, and then divide the product of the lengths of the legs by the length of the hypotenuse. The result obtained will correspond to the total resistance.

Similar to the case discussed above, the total resistance when connecting R and C in parallel will be equal to:

(10)

The total resistance can also be found in this case by constructing a resistance triangle.

In radio engineering, the most common case is the parallel connection of inductance and capacitance, for example, an oscillatory circuit for tuning receivers and transmitters. Since the inductor always has, in addition to inductive resistance, also active resistance, the equivalent (equivalent) circuit of the oscillatory circuit will contain active resistance in the inductive branch (Fig. 7).

Figure 6. Equivalent circuit of an oscillating circuit.

The impedance formula for this case will be:

It will be interesting➡ Calculation of cable cross-section by diameter

(11)

Since usually the active resistance of the coil (R) is very small compared to its inductive resistance (ωL), we have the right to rewrite formula (11) in the following form:

(12)

In an oscillating circuit, the values of L and C are usually selected so that the inductive reactance is equal to the capacitive reactance, i.e., so that the condition is met

(13)

If this condition is met, the total resistance of the oscillatory circuit will be equal to:

(14)

where L is the inductance of the coil in H;

C is the capacitance of the capacitor in F;

R is the active resistance of the coil in Ohms.

Application

In electronic circuits, a capacitor is very often used as a filter element. In this case, engineers take into account the method of connecting this element:

When a capacitor is connected in parallel to a circuit, the device is capable of blocking high frequency current. This filter operates on the principle that resistance depends on current frequency. The higher the frequency, the lower the resistance will be.
When switched on in series, the filter already filters out low-frequency pulses. The second property of such a filter is the ability to prevent direct current from passing through.

Also, a large share of the use of such devices is for audio amplifiers. The capacitor is able to separate alternating and direct current, and therefore work as a low-frequency amplifier. In this case, elements with the smallest capacity are selected.

The devices are also used for DC power supplies or stabilizers. Here the property of separating the constant and variable components is applied. For example, dividing it between consumers using separate outputs for direct and alternating current. In such devices, the capacitor discharges if the load on the circuit is increased by connecting a new device. Thus, the overall ripple in the circuit is smoothed out. If necessary, current of both values can be transmitted through one conductor. This is done as follows - contacts with constant voltage are connected to the terminal of the capacitor for direct contact with alternating voltage. In this way, frequency filtering occurs, pulses are smoothed, and direct current is transferred to the consumer. This circuit is used in antenna amplifiers that are connected to televisions.

Ohm's law for a complete circuit.

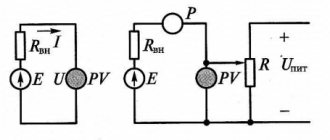
Consider an electrical circuit consisting of a current source and a resistor R.

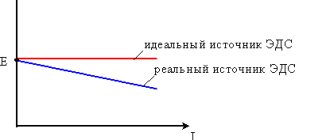
Ohm's law for a complete circuit establishes the relationship between the current in the circuit, the emf and the total resistance of the circuit, consisting of the external resistance R and the internal resistance of the current source r.

The work of external forces Ast of the current source, according to the definition of EMF (ɛ), is equal to Ast = ɛq, where q is the charge moved by the EMF. According to the definition of current, q = It, where t is the time during which the charge was transferred. From here we have:

Ast =ɛIt.

The heat released when doing work in a circuit, according to the Joule-Lenz law, is equal to:

Q = I2Rt + I2rt.

According to the law of conservation of energy, A = Q. Equating (Ast =ɛIt) and (Q = I2Rt + I2rt), we obtain:

ɛ = IR + Ir.

Ohm's law for a closed circuit is usually written as:

The current strength in a complete circuit is equal to the ratio of the circuit's emf to its total resistance.

If a circuit contains several series-connected sources with emf ɛ1, ɛ2, ɛ3, etc., then the total emf of the circuit is equal to the algebraic sum of the emf of the individual sources. The sign of the source emf is determined in relation to the direction of the circuit bypass, which is chosen arbitrarily, for example, in the figure below - counterclockwise.

In this case, external forces inside the source perform positive work. Conversely, the following equation holds true for the circuit:

ɛ = ɛ1 + ɛ2 + ɛ3 = | ɛ1| – | ɛ2| -| ɛ3| .

In accordance with the current strength is positive with a positive EMF - the direction of the current in the external circuit coincides with the direction of bypassing the circuit. The impedance of a circuit with multiple sources is equal to the sum of the external and internal resistances of all sources of EMF, for example, for the figure above:

Rn = R + r1 + r2 + r3.

Formulas, dependencies and types of inductance

Electrical inductance L is a quantity equal to the proportionality coefficient between the current I flowing in a closed circuit and the magnetic flux it creates, otherwise called the flux linkage Y:

Y = LI.

If voltage is applied to the coil terminals for some time, current I will begin to flow in it and a magnetic field will form. The lower the inductance L, the faster this process occurs. As a result, the two-terminal network under consideration will accumulate a certain amount of potential energy. When the power is turned off, it will try to return it. As a result, a self-inductive emf E is formed at the coil terminals, which is many times higher than the initially applied voltage. A similar technology was previously used in magnetos of internal combustion engine ignition systems, and is now widely found in boost DC-DC converters.

Formula for self-induction EMF, here t is the time during which the current I decreases to zero

Simple DC-DC boost converter

A coil (also known as a choke) is a radio component with pronounced inductance, because that’s exactly what it was created for. However, in principle all elements have this property. For example, a capacitor, a resistor, a cable, just a piece of wire, and even the human body also has some inductance. In the calculations of RF circuits, this must be taken into account.

Important! When measuring inductance with a specialized device, it is worth remembering that you cannot hold both of its terminals with your hands. Otherwise, the readings may change and be incorrect. This is caused by the inclusion of the human body with its own inductance in the measured circuit.

How to measure electrical impedance

Let's connect all the parts together into a simple electrical circuit. Let's take a look at the figure below: This is a circuit with a DC power supply. Current flows through a resistor. Quite simple, right? The greater the resistance of the resistor in the circuit, the less current will be.

Simple DC circuit with resistor

at 100 ohms to limit current What happens if we add an AC power supply, an inductor, and a capacitor to an electrical circuit? Now there are two additional components in the circuit, each of which offers resistance to electrical current in its own way. Like a resistor, they both prevent the passage of electrical current while also influencing the current. If you add up the active resistance of the resistor and the active and reactance of the capacitor and inductor, you get the total electrical resistance or impedance.

In an AC circuit, a resistor, an inductor and a capacitor are connected in series

Wait! To calculate the total electrical resistance, it is not enough to simply add the active and reactive resistances. Usually, in most textbooks, an abundance of mathematical formulas begins from this point, so read on slowly.

Combining impedances

Main article: Series and parallel circuits

The total impedance of many simple component networks can be calculated using the rules for combining series and parallel impedances. The rules are identical to those for combining resistances, except that in general the numbers are complex numbers. However, the general case requires equivalent impedance conversions in addition to series and parallel.

Series combination

For components connected in series, the current through each circuit element is the same; Total impedance is the sum of the component impedances.

Z eq = Z 1 + Z 2 + ⋯ + Z n { displaystyle Z_{ text{eq)) = Z_{1} + Z_{2} + cdots + Z_{n} quad}

Or explicitly in real and imaginary terms:

Z eq = r + j x = ( r 1 + r 2 + ⋯ + r p ) + j ( x 1 + x 2 + ⋯ + x p ) { displaystyle Z_{ text{eq)) = R + jX = ( R_{1} + R_{2} + cdots + R_{n}) + j (X_{1} + X_{2} + cdots + X_{n}) quad}

Parallel combination

For components connected in parallel, the voltage across each circuit element is the same; the ratio of currents through any two elements is the reciprocal of their impedances.

Therefore, the reverse total impedance is the sum of the reverse impedances of the components:

1 Z eq = 1 Z 1 + 1 Z 2 + ⋯ + 1 Z p { displaystyle { frac {1} {Z_{ text {eq)))} = { frac {1} {Z_{1))} + { frac {1} {Z_{2}}} + cdots + { frac {1} {Z_{n}}}}

or, when n = 2:

1 Z eq = 1 Z 1 + 1 Z 2 = Z 1 + Z 2 Z 1 Z 2 { displaystyle { frac {1} {Z_{ text {eq)))} = { frac {1} {Z_{1} }} + { frac {1} {Z_ {2}}} = { frac {Z_ {1} + Z_ {2}} {Z_ {1} Z_ {2}}}} Z eq = Z 1 Z 2 Z 1 + Z 2 { displaystyle Z_{ text {eq)) = { frac {Z_ {1} Z_ {2)) {Z_ {1} + Z_ {2)))}

The equivalent impedance Z eq { displaystyle Z_{ text{eq))} can be calculated in terms of equivalent series resistance R eq { displaystyle R_{ text{eq))} and reactance X eq { displaystyle X_{ text{eq} }} .[eleven]

Z eq = r eq + j x eq eq = ( x 1 r 2 + x 2 r 1 ) ( x 1 + x 2 ) + ( r 1 r 2 − x 1 x 2 ) ( r 1 + r 2 ) ( r 1 + r 2 ) 2 + ( X 1 + X 2 ) 2 X eq = ( X 1 r 2 + X 2 r 1 ) ( r 1 + r 2 ) − ( r 1 r 2 − X 1 X 2 ) ( X 1 + X 2 ) ( R 1 + R 2 ) 2 + ( X 1 + X 2 ) 2 { displaystyle { begin{align} Z_{ text{eq)) & = R_{ text{eq)) + jX _{ text{eq}} R_{ text{eq}} & = { fracturing {(X_{1} R_{2} + X_{2} R_{1}) (X_{1} + X_{2}) + (R_ {1} R_ {2} -X_ {1} X_ {2 }) (R_ {1} + R_ {2})} {(R_ {1} + R_ {2}) ^ {2} + ( X_{1} + X_{2})^{2}}} X_{ text{eq}} & = { frac {(X_{1} R_{2} + X_{2} R_{1}) (R_ {1} + R_{2}) — (R_ {1} R_ {2} -X_ {1} X_ {2}) (X_ {1} + X_ {2})} {(R_ {1} + R_ { 2}) ^ {2} + (X_ {1} + X_ {2}) ^ {2}}} end {align}}}

Practical application of electrical impedance

It becomes clear, in the end, that after all our explanations, figuring out what total electrical resistance is is not difficult, isn’t it? There are dozens of free calculators to help you do the calculations. What you really need is to know that impedance works in the same way as resistive resistance to limit the current in an AC circuit.

The ability of components such as capacitors and inductors to respond to constant changes in alternating current makes them unique. Thanks to the impedance in your circuit, you can organize something similar to an electrical panel with circuit breakers that respond to unexpected surges in electricity, protecting your home electrical wiring from burning out. You can also thank impedance for allowing you to carry your laptop with a fully charged battery without fear of it exploding.

When it comes to working with AC powered devices, be it a laptop or the electrical panel in your home, you have electrical impedance to thank. And remember, electrical impedance is simply the big brother of the familiar active resistance, which combines active and reactance into one simple formula.

Calculation of the inductive reactance of the coil

Any inductance, incl. coil provides some resistance to alternating current. How to calculate it was described above. From the formula XL=2pfL it is clear that the resistance of the inductor primarily depends on the frequency of the current flowing through it and its inductance. Moreover, the relationship with both parameters is directly proportional.

Frequency is a characteristic of the external environment; the inductance of the coil depends on a number of its geometric properties:

Where:

u0 – magnetic permeability of vacuum – 4p*10-7 H/m;
ur – relative permeability of the core;
N – number of throttle turns;
S – its cross section in m2;
l is the length of the coil in meters.

Having the above-described formulas and information about the material and dimensions of the coil, you can fairly accurately estimate its inductive reactance without any measuring instruments.

Additional Information. Some digital multimeters have an inductance mode. This feature is rare, but sometimes it turns out to be very useful. Therefore, when choosing a device, you should pay attention to whether it is capable of measuring inductance.

Principle of operation

A capacitor with a certain capacity operates on the principle of a period, which consists of charging and discharging an element. The period is divided into 4 parts:

The first part involves increasing tension. At this moment, the capacitor resistance is minimal and the charging current is very high.
In the second quarter, its capacity is filled due to the charging current.
In the third quarter, the capacitor is fully charged, and the current decreases down to 0. The EMF increases with the effect of changing its direction.
In the last quarter the element is discharged. At this stage, the EMF will be within 0, and the current will gradually increase.

All the processes described in one period determine a further phase shift of 90 degrees.

The nature of the occurrence of capacitance depends entirely on several factors:

It is necessary to have a capacitor in the circuit.
Only alternating current should flow through the circuit.
The conductor resistance must be less than the capacitance of the capacitor.

All these factors help to calculate the most correct value of capacitance characteristics for the most efficient operation of the electrical circuit.