Why resistance occurs
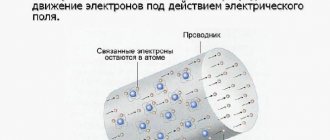
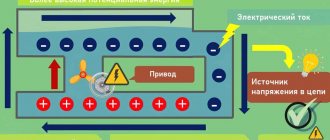
Electrons, colliding with charged atoms (ions) that make up the crystal lattice of the conductor, lose speed. The mass of an atom significantly exceeds the mass of an electron, so their collision leads to a loss of speed (“braking”) and a change in the direction of motion of the electron. This creates resistance to the flow (increase) of current. This means that resistance is a physical quantity.
Collisions of electrons with atoms.
What is it measured in?
According to the international system of units, the value is measured in ohms multiplied by a meter. In some cases, the unit used is ohm multiplied by millimeter squared divided by meter. This is a designation for a conductor having a meter length and a square millimeter cross-sectional area.
Unit
What is electrical resistance
Positioned as the resistance of some element that serves as a conductor. It has a unit length, an indicator of cross-sectional area. This is the physical meaning. Electrical resistance is a conductor parameter based on:
- material structure;
- form parameter;
- product dimensions.
Formula how to find
According to the provision from any textbook on electrodynamics, the resistivity of the conductor material formula is equal to the proportion of the total resistance of the conductor per cross-sectional area, divided by the conductor length. It is important to understand that the final indicator will be influenced by temperature and the degree of material purity. For example, if you add a little manganese to copper, the overall indicator will be increased several times.
Main calculation formula
Interestingly, there is a formula for inhomogeneous isotropic material. To do this, you need to know the electric field strength with the electric current density. To find it, you need to divide the first quantity by the other. In this case, the result is not a constant, but a scalar quantity.
Ohm's law in differential form
There is another, more difficult to understand formula for an inhomogeneous anisotropic material. Depends on the tensor coordinate.
It is important to note that the relationship between resistance and conductivity is also expressed by formulas. There are rules for finding isotropic and anisotropic materials through tensor components. They are shown in the diagram below.
Relationship with conductivity expressed in physical relations
Example task No. 1
The length of the copper wire used in the lighting network is $100 \space m$, its cross-sectional area is $2 \space mm^2$. What is the resistance of such a wire?
In order to calculate the resistance of such a conductor, we need the value of its resistivity. The resistivity of copper is $0.017 \frac{Ohm \cdot mm^2}{m}$. Since this value is given to us precisely in these units of measurement, we will not convert the cross-sectional area value expressed in $mm^2$ to SI.
Now we can write down the condition of the problem and solve it.
Given: $l = 100 \space m$ $S = 2 \space mm^2$ $\rho = 0.017 \frac{Ohm \cdot mm^2}{m}$
$R — ?$
Solution:
The formula for calculating the resistance of a conductor is: $R = \frac{\rho l}{S}$.
Let's calculate it: $R = \frac{0.017 \frac{Ohm \cdot mm^2}{m} \cdot 100 \space m}{2 \space mm^2} = \frac{1.7 \space Ohm}{2} = 0.85 \space Ohm$.
Answer: $R = 0.85 \space Ohm$.
What does it depend on
Resistance depends on temperature. It increases when the thermometer rises. This is explained by physicists in such a way that as the temperature increases, atomic vibrations in the crystalline conductor lattice increase. This prevents free electrons from moving around.
Note! As for semiconductors and dielectrics, the value decreases due to the fact that the structure of the concentration of charging carriers increases.
Temperature dependence as the main property of conductive resistance
What do resistivity numbers mean?
In order to be able to compare the resistivity of different materials, from products such as copper and aluminum to other metals and substances including bismuth, brass and even semiconductors, it is necessary to use a standard measurement.
The unit of resistivity in the International System of Units (SI) is Ohm m.
The SI unit of resistivity is equal to the resistivity of a substance such that a homogeneous conductor 1 m long with a cross-sectional area of 1 m2, made from this substance, has a resistance of 1 ohm. Accordingly, the resistivity of an arbitrary substance, expressed in SI units, is numerically equal to the resistance of a section of an electrical circuit made of a given substance with a length of 1 m and a cross-sectional area of 1 m2
Resistivity values of some substances
Table 1 shows the resistivity values of some substances.
| Substance | $\rho$, $\frac{Ohm \cdot mm^2}{m}$ | Substance | $\rho$, $\frac{Ohm \cdot mm^2}{m}$ |
| Silver | $0.016$ | Manganin (alloy) | $0.43$ |
| Copper | $0.017$ | Constantan (alloy) | $0.50$ |
| Gold | $0.024$ | Mercury | $0.96$ |
| Aluminum | $0.028$ | Nichrome (alloy) | $1.1$ |
| Tungsten | $0.055$ | Fechral (alloy) | $1.3$ |
| Iron | $0.10$ | Graphite | $13$ |
| Lead | $0.21$ | Porcelain | $10^{19}$ |
| Nickelin (alloy) | $0.40$ | Ebonite | $10^{20}$ |
Table 1. Resistivity of some substances (at $t = 20 \space \degree C$)
Please note that these values are valid at a temperature of $20 \space \degree C$.
The resistivity of a substance depends on temperature.
Experiments have shown that at a certain temperature for each substance, a dielectric can become a conductor (semiconductor). It has also been experimentally proven that with increasing temperature the resistivity of metals increases.
Resistivity table for common conductors
The table below shows resistivity values for various materials, particularly metals used for electrical conductivity.
Resistivity indicators are given for such “popular” materials as copper, aluminum, nichrome, steel, lead, gold and others.
MaterialResistivity, ρ, at 20 °C (Ohm m)Source
| Brass | ~0.6 – 0.9 x 10-7 | |
| Silver | 1.59×10−8 | [3][4] |
| Copper | 1.68×10−8 | [5][6] |
| Burnt copper | 1.72×10−8 | [7] |
| Gold | 2.44×10−8 | [3] |
| Aluminum | 2.65×10−8 | [3] |
| Calcium | 3.36×10−8 | |
| Tungsten | 5.60×10−8 | [3] |
| Zinc | 5.90×10−8 | |
| Cobalt | 6.24×10−8 | |
| Nickel | 6.99×10−8 | |
| Ruthenium | 7.10×10−8 | |
| Lithium | 9.28×10−8 | |
| Iron | 9.70×10−8 | [3] |
| Platinum | 1.06×10−7 | [3] |
| Tin | 1.09×10−7 | |
| Tantalum | 1.3×10−7 | |
| Gallium | 1.40×10−7 | |
| Niobium | 1.40×10−7 | [8] |
| Carbon steel (1010) | 1.43×10−7 | [9] |
| Lead | 2.20×10−7 | [2][3] |
| Galinstan | 2.89×10−7 | [10] |
| Titanium | 4.20×10−7 | |
| Electrical steel | 4.60×10−7 | [11] |
| Manganin (alloy) | 4.82×10−7 | [2] |
| Constantan (alloy) | 4.90×10−7 | [2] |
| Stainless steel | 6.90×10−7 | |
| Mercury | 9.80×10−7 | [2] |
| Manganese | 1.44×10−6 | |
| Nichrome (alloy) | 1.10×10−6 | [2][3] |
| Carbon (amorphous) | 5×10−4 — 8×10−4 | [3] |
| Carbon (graphite) parallel-basal plane | 2.5×10−6 — 5.0×10−6 | |
| Carbon (graphite) perpendicular-basal plane | 3×10−3 | |
| Gallium arsenide | 10−3 to 108 | |
| Germanium | 4.6×10−1 | [3][4] |
| Sea water | 2.1×10−1 | |
| Swimming pool water | 3.3×10−1 — 4.0×10−1 | |
| Drinking water | 2×101 — 2×103 | |
| Silicon | 2.3×103 | [2][3] |
| Wood (wet) | 103 — 104 | |
| Deionized water | 1.8×105 | |
| Glass | 1011 — 1015 | [3][4] |
| Carbon (diamond) | 1012 | |
| Hard rubber | 1013 | [3] |
| Air | 109 — 1015 | |
| Wood (dry) | 1014 — 1016 | |
| Sulfur | 1015 | [3] |
| Fused quartz | 7.5×1017 | [3] |
| PAT | 1021 | |
| Teflon | 1023 — 1025 |
It can be seen that the resistivity of copper and the resistivity of brass are both low, and considering their cost relative to silver and gold, they become cost-effective materials to use for many wires. Copper's resistivity and ease of use have led to it being widely used as a conductor material on printed circuit boards.
Occasionally, aluminum and especially copper are used due to their low resistivity. Most wires used for interconnection today are made of copper because it provides low resistivity at an affordable cost.
The resistivity of gold is also important because gold is used in some critical applications despite its cost. Gold plating is often found on high quality low current connectors where it provides the lowest contact resistance. The gold coating is very thin, but even so it is able to provide the required characteristics of the connectors.
Silver has a very low level of resistivity, but is not widely used due to its cost and because it tarnishes, which can result in higher contact resistance.
However, it is used in some radio transmitter coils where silver's low electrical resistivity reduces losses. When used for such purposes, silver was usually applied only to the existing copper wire. Coating the wire with silver allowed for significant cost savings compared to solid silver wire without significantly compromising performance.
It will be interesting➡ Thermal relay for an electric motor
Other materials in the electrical resistivity table may not have such obvious uses. Tantalum appears in the table because it is used in capacitors—nickel and palladium are used in the end connections of many surface mount components such as capacitors.
Quartz finds its main application as a piezoelectric resonant element. Quartz crystals are used as frequency-determining elements in many oscillators, where the high Q value allows for very frequency-stable circuits. They are similarly used in high efficiency filters. Quartz has a very high level of resistivity and is not a good conductor of electricity, meaning it is classified as a dielectric.
Concept of electrical resistance of a conductor
The classical definition explains electric current by the movement of “free” (valence) electrons. It is provided by the electric field created by the source. Movement in the metal is hampered not only by the normal components of the crystal lattice, but also by defective areas, impurities, and inhomogeneous areas. During collisions with obstacles, due to the transition of momentum into thermal energy, the temperature increases.
A good example is heating water with a boiler.
In gases, electrolytes and other materials the physics of the phenomenon is somewhat different. Linear relationships are observed in metals and other conductors. The basic relationships are expressed by the well-known formula of Ohm's law:
R (electrical resistance) = U (voltage) / I (current).
For convenience, the inverse quantity, conductivity (G = 1/R), is often used. It denotes the ability of a certain material to pass current with certain losses.
To simplify, the example of a water pipe is sometimes used. A moving fluid is an analogue of a current. Pressure is the equivalent of voltage. By decreasing (increasing) the cross section or position of the locking device, the conditions of movement are determined. In a similar way, the basic parameters of electrical circuits are changed using resistance (R).
For your information. The amount of liquid passing per unit time through the control section of the pipe is the equivalent of electrical power.
Formulation of Ohm's law
The current strength in a particular section of the circuit will be directly proportional to the voltage and inversely proportional to the resistance.
Ohm's law for a circuit section
Given according to the classical formula: \( I=U/R \)
This does not take into account the resistance of the entire circuit, but it is possible to measure the resistance indicator in each individual section.
Ohm's law for a complete circuit
Determined by the formula:
\[ I= E/R+r \]
As a definition, this formulation will sound like this: the current strength is directly proportional to the emf and inversely proportional to the total resistance. Total resistance means the sum of external and internal resistance.
Ohm's law in differential and integral forms
- In differential form it is used when it is necessary to determine the parameters of a negligibly small section of a circuit.
- In integral form, a circuit is considered, with the expectation of the presence of a current source, as well as without it.
Temperature dependence ρ(T)
For most materials, numerous experiments have been carried out to measure resistivity values. Data for most conductors can be found in reference tables.
Specific resistance of metals and alloys, Ohm*mm2/m
(at T = 20C)
| Silver | 0,016 | Bronze (alloy) | 0,1 |
| Copper | 0,017 | Tin | 0,12 |
| Gold | 0,024 | Steel (alloy) | 0,12 |
| Aluminum | 0,028 | Lead | 0,21 |
| Iridium | 0,047 | Nickelin (alloy) | 0,42 |
| Molybdenum | 0,054 | Manganin (alloy) | 0,45 |
| Tungsten | 0,055 | Constantan (alloy) | 0,48 |
| Zinc | 0,06 | Titanium | 0,58 |
| Brass (alloy) | 0,071 | Mercury | 0,958 |
| Nickel | 0,087 | Nichrome (alloy) | 1,1 |
| Platinum | 0,1 | Bismuth | 1,2 |
Most often, the values of ρ are given at normal, that is, room temperature 20C. But it turned out that with increasing temperature, the resistivity increases linearly in accordance with the formula:
$ ρ(T) = ρ0 * (1 + α*T)$ (6),
where: ρ is the resistivity of the conductor at a temperature of 0C, α is the temperature coefficient of resistivity, which also has its own individual meaning for each substance. From formula (6) it follows that the coefficient α has dimension or .
In accordance with the Joule-Lenz law, when an electric current flows, heat is released, which means the temperature of the conductor increases. In addition, depending on the area of application, electrical devices can operate at both low (minus) and high temperatures. For accurate calculations of electrical circuits, it is necessary to take into account the dependence ρ(T). The value of α for a specific material can be found in reference literature.
2021 Reference books – Mobile version – Electrical engineering
• Electrical fuses.
• Modern magnets (determination of polarity, measurement of induction). • Uninterruptible Power Supply (UPS) • Solar batteries and thermogenerators (autonomous power supply). • Table of electrical resistivities (for metals, graphite, water and earth). • “Ground electric battery” – free energy (FE). • Batteries of various sizes - how to choose and test. Reminder on electrical engineering
Ohm's law establishes the relationship between the current strength in a conductor and the potential difference (voltage) at its ends. The formulation for a section of an electrical circuit (conductor) that does not contain sources of electromotive force (EMF): the current strength is directly proportional to the voltage and inversely proportional to the resistance of the conductor. Ohm's laws for a closed unbranched circuit: the current strength is directly proportional to the electromotive force and inversely proportional to the total resistance of the circuit. Ohm's law is valid for direct and quasi-stationary currents. It was discovered by the German physicist Georg Ohm in 1826. * Modern Encyclopedia
In the case of alternating current, the quantities included in the calculation formulas become complex.
Ohm's law in differential form describes exclusively the electrically conductive properties of a material, regardless of geometric dimensions.
The electrical resistivity of a substance is the electrical resistance of a cube made from it with sides equal to one (1 meter), when the current flows perpendicular to its two opposite faces, each with an area of 1 square meter.
Specific resistance depends on the concentration of free electrons in the conductor and on the distance between the ions of the crystal lattice, in other words, on the material of the conductor.
Dimension of electrical resistivity in system. SI (International System of Units) – Ohm m [Ohm*m^2/m]
(SI – Ω·m, Russian – Ohm-meter, English – ohm-meter).
To measure conductor materials, it is allowed to use a non-system unit - Ohm mm2/m
(for a millimeter cross-section of a conductor, 1 m long, that is, a millionth of an Ohm meter).
The physical meaning of resistivity: a material (homogeneous and isotropic*) has a specific electrical resistivity of one Ohm m, if a cube with a side of 1 meter made from this material has a resistance of 1 Ohm when measured on opposite faces of the cube. * Isotropy – identical physical properties in all directions.
Specific resistance characterizes the ability of a substance to conduct electric current and does not depend on the shape and size of the substance, but changes when its temperature differs from 20 °C (that is, from room temperature, at which the tabular values for reference books were determined).
In practice, in technology a unit is more often used that is a million times smaller (millimeter current-carrying cross-section) than Ohm m:
1 µOhm m (SI – µΩ m, Russian – microohm-meter, English – microhm-meter) = 1*10^-6 Ohm*m 1 µOhm m = 1 Ohm mm2/m
At the same time, the resistivity of a homogeneous piece of conductor 1 meter long and a current-carrying cross-sectional area of 1 square millimeter is equal to 1 Ohm mm2/m, if its resistance is 1 Ohm. For example, the resistivity value of electrical copper is approximately 1.72 * 10^-8 Ohm m = 0.0172 μOhm m (determined at a temperature of 20 degrees Celsius).
Depending on the resistivity, all substances are divided into conductors, dielectrics and semiconductors. Dielectrics (insulators, for example, porcelain) have very high electrical resistivity values, exceeding 10^12 Ohm m, and conductors (for example, silver, copper) - less than 10^-2 Ohm m (
Ratios:
1 Ohm mm2/m = 1 μOhm m ( 1 * 10^-6 Ohm * m ) 1 Ohm cm = 0.01 Ohm m 1 Ohm m = 100 Ohm cm (Ohm-centimeter) )
Electrical conductivity is the reciprocal of electrical resistance. The SI unit of electrical conductivity is Siemens (denoted by Sm). For example, copper has an electrical conductivity of approximately 58,100,000 S/m (1 / 58100000 ~ 0.0172 x 10-6 ohm.m), measured at 20 °C
Formula for calculating electrical resistance at constant current
R = (p * L) / S
where: R – electrical resistance of the wire; p – resistivity: p [Ohm mm2/m] = (R * S) / L [ Om * mm^2 / m ] L – length, m; S – cross section: square meter or millimeter (m2 or mm2). S = 3.14 * (radius)^2
If the electrical resistivity is in Ohm mm2/m, then S (section) should be in mm2, L (length) should be in meters. If in Ohm cm (Ohm-centimeter, abbreviated from Ohm*cm^2 / cm), then S is in cm2, L is in centimeters. If the specific resistance is in Om·m (Ohm-meter, from Ohm*m^2 / m), then S is in m2, L is in meters.
1 Ohm mm2/m = 1 μOhm m (derived submultiple unit of electrical resistivity in the SI system, used in practice in technical calculations – millionth part Ohm•m)
For an electrician and an experienced radio amateur, the ability to estimate by eye the cross-section of an electrical wire, taking into account the insulation layer, is like the absolute pitch of a musician who immediately determines the pitch of the sounds heard and writes them down in the form of musical notes and register keys.
Example, as a sample for the ratio of quantities. Electrical resistivity of pure electrical copper (after treatment by recrystallization annealing), measured at a temperature of 20 °C:
0.0172 µOhm (micro-ohm meter, 10^-6 Ohm•m)
1.72*10^-2 Ohm*mm^2/m (actual electrical resistance of a copper conductor, 1 meter long and 1 mm2 cross-section)
1.72*10^-6 Ohm•cm (wire dimensions - in centimeters)
1.72*10^-8 Ohm•m (abbreviated from Ohm*m^2/m – meter cube, current-carrying cross-sectional area – 1m2, i.e. between opposite faces)
17.2 nOhm•m (nanohm-meter, 10^-9 Ohm•m)
Highly conductive metals (no more than 0.1 μOhm.m) are used for the manufacture of wires, conductive cores of cables, windings of electrical machines and transformers, etc. Metals and alloys of high resistance (at least 0.3 microohm-meter) are used for the production of standard resistors, rheostats, electrical measuring instruments, electric heating devices, incandescent lamp filaments, etc. Heating alloys must withstand prolonged operation in the open air without destruction at temperatures of at least 1000 °C.
Table of electrical resistivity values, µOhm m (microohm meter) = Ohm mm2/m (equal numerical values) at an ambient temperature of 20 degrees Celsius Silver – 0.015-0.016 Copper – 0.0172-0.0180 Gold – 0.024 Aluminum – 0.026-0.030 Tungsten – 0.053-0.055 Zinc 0.053-0.062 Nickel – 0.068-0.073 Brass (copper-zinc alloy) – 0.043-0.108 Iron – 0.098 Steel – 0.10-0.14 Tin – 0.12 Tin-pig tsovy solder – 0.14-0.16 Bronze alloys – 0.02-0.2 Lead – 0.217-0.227 Nickel – 0.4 Manganin – 0.42-0.48 Constantan – 0.48-0.52 Nichrome – 1 .05-1.40 Fechral – 1.15-1.35 Carbon-graphite brushes for electrical machines – 20-50 Carbon welding electrode – 50-90 µOhm m
Mineral water (with water mineralization – 2-7 grams per liter) – 1-4 *10^6 μOhm m = 1-4 Ohm•m Ground water – 10-50 *10^6 Wet / damp garden soil (top layer of soil , soil – after watering) – 20-60 *10^6
Why are high voltages used in electrical networks?
In a power transmission line, with constant transmitted power, its losses increase in direct proportion to the length of the power line and inversely proportional to the square of the emf. Thus, it is considered desirable to increase the voltage to values of tens (intra-city overhead and cable power transmission networks at 380 volts, 6, 10, 20, 35, 110, 220 and 330 kV) and hundreds of kilovolts (main ultra-high power transmission lines - power lines 500-750 kV and ultra-high voltage, 1150 kV and above) on alternating and direct current lines (150, 400, 800 kV). But, with such operating parameters, constantly growing consumption of electrical energy and frequent peak overloads, wear and tear of equipment, lack of reserve capacity, weather anomalies, local non-compliance with safety requirements, unprofessionalism and basic sloppiness can cause emergency situations and system accidents (now called English manner - blackout). For this reason, municipal authorities of any village and city have a constant headache in providing backup power sources (batteries and diesel generators) for uninterrupted power supply to social facilities under a backup scheme.
Special alloys based on copper, in electrical engineering
For high currents, up to 10 A, a high-power wire resistor called a rheostat is used. As a winding, a wire made of a thermostable (with a minimum temperature coefficient) alloy with high resistivity, for example, constantan (40% Ni, 1.2% Mn, 58.8% Cu), is used. If the voltage between adjacent turns does not exceed 1 volt, such a wire can be wound tightly, turn to turn, without special insulation between turns, due to the presence of a natural oxide film formed on the surface of a given metal, with rapid (no more than three seconds) heating to a sufficiently high temperature (about 900 °C).
In high-precision devices, manganin (3%Ni, 12%Mn, 85%Cu) is used, which is less heat-resistant, but, unlike constantan wire, has a very low thermoEMF (contact electrical potential difference) when paired with copper.
Designations of recommended multiples and sub-multiple values from SI units
10^9 Ohm – gigaohm GOhm GΩ 10^6 Ohm – megaohm MOhm MΩ 10^3 Ohm = 1000 Ohm – kiloohm kOhm kΩ. 10^-2 Ohm – centiohm cOhm cΩ 10^-3 Ohm – milliohm mOhm mΩ. 10^-6 Ohm – microohm µOhm µΩ 10^-9 Ohm – nanoohm nOhm nΩ
Dependence of resistance on temperature
When heated, the electrical resistance of metal conductors increases, and when cooled, it decreases. To calculate, according to the formula, electrical resistance at a certain temperature, the so-called “temperature coefficient of resistance” (TCR) is used. Calculations are carried out from a certain initial temperature level. For a temperature range within normal weather conditions (in winter and summer) of the environment, the dependence for the conductor is described by the mathematical formula:
R2 = R1 * (1 + α * (t2 – t1)),
where R1 (initial, known value, at zero or 20 degrees Celsius, measured or calculated) and R2 (desired) are the resistor resistances, respectively, at temperatures t1 (0 ° C or 20 ° C) and t2; α – temperature coefficient of resistance (from the reference table), equal to the relative change in electrical power. resistance (specific or absolute) when the temperature changes by 1 °C. Since TCR values are very small, in reference books they are indicated in units of thousandths or millionths (ppm/°C - Parts Per Million) of a relative change in resistance per degree.
Usually, the initial, tabulated values of various physical constants are given either to normal room temperature +20 ° C or to zero (in reference tables of conductor and rheostatic materials used in electrical devices).
In metal thermometers made from copper or platinum wire, the electrical resistance increases almost linearly with increasing temperature (without extremely high values for these materials). But, when, for example, a thin copper wire is heated excessively to a red-hot temperature, its active electrical resistance to direct current increases many times over.
Calculation example for a hundred-meter aluminum busbar, with a radius of 40 mm, heated to 95°C: R = (R1 * (1 + α * (t2–t1))) * L / S = = 2.62*10-8 Ohm•m * (1 + 0.0042*95) * 100 / (3.14 * 402 * 10-6) = 7.3 * 10-4 Ohm where: S – cross-sectional area in m2 (minus the thickness of the insulation layers), L – length of the conductor in meters.
Temperature coefficient of resistance x10-3, 1/degree: Aluminum – 4.2 Hard-drawn tin bronze – 0.6-0.7 Tungsten – 4.2 Graphite – -1.3 Dural – 2.2 Constantan – 0.003-0.005 Brass – 1.5 Manganin – 0.03-0.06 (at temperatures up to 250-300°C) Copper – 4.3 Nichrome – 0.14 Silver – 4.0 Steel – 9.0 Zinc – 4.2
Fixed resistors and their markings
In the alphanumeric (code) marking of resistors - the numerical value of the electrical resistance and the letters are applied to their body, the first of which indicates the multiplier (R or E - Ohm, K - kiloohm, M - megohm) and, at the same time, determines the position of the decimal point sign. The second letter means the accuracy class, that is, the permissible deviation from the specified value. Ratings for small parts are applied in the form of markings with colored rings, stripes or dots (depending on the standard used). Each color corresponds to a specific number indicating the number of ohms, multiplier/power or percentage of accuracy. To quickly determine the resistor value by color coding, special computer programs are used. Read more…
An example of a calculation based on a school physics problem from the 9th grade curriculum.
Task: determine (find in the table), based on the known resistivity p = 0.017 Ohm mm2/m - what material is this? Calculate the wire diameter. Calculate the electrical resistance of a wire with a length L = 80 cm, cross section S = 0.2 mm2 Solution to the problem: From the table we determine that copper can have a resistivity equal to 0.017 Ohm mm2/m.
From the formula S = 3.1416 * (radius)^2 = 3.142 * ((diameter)^2)/4 using your calculator, find the diameter (in millimeters) = square root of (4 * S / 3.14)
Wire length, in SI units (converted to meters): 80 cm = 0.8 m
We find the electric resistance according to the formula: R = (p * L) / S = (0.017 * 0.8) / 0.2 = 0.068 Ohm
Answer: accurate to the second decimal place, R = 0.07 Ohm
[ to Home Page ]
Place an ad
The latest financial news from the site Finversiya.ru Electrical work - electrical installation, connection and maintenance of electrical wiring. | Mini-handbook on electrical parameters: ratios of Ohm x mm2/m and µOhm x m (micro-ohm), in technical calculations for metals, alloys and powder mixtures.
Copyright © 2007-2021, KAKRAS.RU
Electrical resistivity
Further research made it possible to establish a connection between the value of electrical resistance and its basic geometric dimensions. It turned out that the resistance of the conductor is directly proportional to the length of the conductor L and inversely proportional to the cross-sectional area of the conductor S.
This functional relationship is well described by the following formula:
$ R = ρ *{ Lover S} $ (4)
The constant value ρ for each substance was called resistivity. The value of this parameter depends on the density of the substance, its crystal structure, atomic structure and other internal characteristics of the substance. From formula (4) you can obtain a formula for calculating resistivity if experimental values for R, L and S are available:
$ ρ = R*{ Sover L } $ (5)
For most known substances, measurements were made and entered into reference tables of electrical resistance of conductors.
Specific resistance of metals, Ohm*mm2/m
(at T = 20C)
| Silver | 0,016 | Bronze (alloy) | 0,1 |
| Copper | 0,017 | Tin | 0,12 |
| Gold | 0,024 | Steel (alloy) | 0,12 |
| Aluminum | 0,028 | Lead | 0,21 |
| Iridium | 0,047 | Nickelin (alloy) | 0,42 |
| Molybdenum | 0,054 | Manganin (alloy) | 0,45 |
| Tungsten | 0,055 | Constantan (alloy) | 0,48 |
| Zinc | 0,06 | Titanium | 0,58 |
| Brass (alloy) | 0,071 | Mercury | 0,958 |
| Nickel | 0,087 | Nichrome (alloy) | 1,1 |
| Platinum | 0,1 | Bismuth | 1,2 |
It was experimentally discovered that as the temperature decreases, the resistance of metals decreases. When approaching the temperature of absolute zero, which is -273C, the resistance of some metals tends to zero. This phenomenon is called superconductivity. Atoms and molecules seem to “froze”, stop any movement and offer no resistance to the flow of electrons.
Content
The current strength in the circuit is determined by the electric charge passing through the cross section of the conductor per unit time: $I = \frac{q}{t}$.
Electrical voltage is another physical quantity that characterizes the electric field. It is equal to the ratio of the work done by the current in a given section to the electric charge passing through this section: $U = \frac{A}{q}$.
Electrical resistance is a value that depends on the properties of the conductor. The resistance value is not affected by either the current in the conductor or the voltage at its ends. It can be calculated using the formula $R = \frac{\rho l}{S}$, where $\rho$ is the resistivity of the conductor, $l$ is the length of the conductor, $S$ is its cross-sectional area. The resistivity value for a specific substance can be found in Table 1 in the lesson “Calculating conductor resistance. Resistivity".
These three physical quantities (current, voltage and resistance) are interconnected by Ohm's law for a section of a circuit: $I = \frac{U}{R}$. The current strength in a conductor is directly proportional to the voltage at its ends and inversely proportional to its resistance.
In this lesson you will learn how to use this knowledge to solve problems. We'll look at a few examples and then move on to the exercises and their detailed solutions.
Iron as a conductor in electrical engineering
Iron is the most common metal in nature and technology (after hydrogen, which is also a metal). It is the cheapest and has excellent strength characteristics, therefore it is used everywhere as the basis for the strength of various structures.
In electrical engineering, iron is used as a conductor in the form of flexible steel wires where physical strength and flexibility are needed, and the required resistance can be achieved through the appropriate cross-section.
Having a table of resistivities of various metals and alloys, you can calculate the cross-sections of wires made from different conductors.
As an example, let's try to find the electrically equivalent cross-section of conductors made of different materials: copper, tungsten, nickel and iron wire. Let's take aluminum wire with a cross-section of 2.5 mm as the initial one.
We need that over a length of 1 m the resistance of the wire made of all these metals is equal to the resistance of the original one. The resistance of aluminum per 1 m length and 2.5 mm section will be equal to
, where R is the resistance, ρ is the resistivity of the metal from the table, S is the cross-sectional area, L is the length.
Substituting the original values, we get the resistance of a meter-long piece of aluminum wire in ohms.
After this, let us solve the formula for S
, we will substitute the values from the table and obtain the cross-sectional areas for different metals.
So,
Since the resistivity in the table is measured on a wire 1 m long, in microohms per 1 mm2 section, then we got it in microohms. To get it in ohms, you need to multiply the value by 10-6. But we don’t necessarily need to get the number ohm with 6 zeros after the decimal point, since we still find the final result in mm2.
- Copper
- Tungsten
- Nikelin
- Iron
As you can see, the resistance of the iron is quite high, the wire is thick.
It will be interesting➡ Total current law for a magnetic field
But there are materials for which it is even greater, for example, nickel or constantan.
Exercises
Exercise No. 1
The length of one wire is $20 \space cm$, the other is $1.6 \space m$.
The cross-sectional area and material of the wires are the same. Which wire has more resistance and by how much? Please note that if the material of the conductors is the same, then the resistivity values $\rho$ for these conductors are also the same.
Given: $l_1 = 20 \space cm$ $l_2 = 1.6 \space m$ $S_1 = S_2 = S$ $\rho_1 = \rho_2 = \rho$
SI: $l_1 = 0.2 \space m$
$\frac{R_2}{R_1} — ?$
Show solution and answer
Hide
Solution: The conductor resistance is calculated using the formula $R = \frac{\rho \cdot l}{S}$.
Resistance of the first wire: $R_1 = \frac{\rho_1 l_1}{S_1} = \frac{\rho l_1}{S}$.
Resistance of the second wire: $R_2 = \frac{\rho_2 l_2}{S_2} = \frac{\rho l_2}{S}$.
Now let's compare them to each other: $\frac{R_2}{R_1} = \frac{\frac{\rho l_2}{S}}{\frac{\rho l_1}{S}} = \frac{l_2}{l_1 }$.
Let's substitute the numerical values of the wire lengths: $\frac{R_2}{R_1} = \frac{1.6 \space m}{0.2 \space m} = 8$.
It turns out that the resistance of the second wire is 8 times greater than the resistance of the first .
This is logical, because you know that the longer the conductor, the greater its resistance .
Answer: the resistance of the second wire is 8 times greater than the resistance of the first.
Exercise No. 2
Calculate the resistance of the following conductors made:
- Made of aluminum wire with a length of $80 \space cm$ and a cross-sectional area of $0.2 \space mm^2$
- Made of nickel wire with a length of $400 \space cm$ and a cross-sectional area of $0.5 \space mm^2$
- Made of constantan wire with a length of $50 \space cm$ and a cross-sectional area of $0.005 \space cm^2$
To solve this problem, we will need tabulated resistivity values for the substances from which the wires are made. Resistivity of aluminum - $0.028 \frac{Ohm \cdot mm^2}{m}$, nickel - $0.4 \frac{Ohm \cdot mm^2}{m}$, constantan - $0.5 \frac{Ohm \cdot mm^2 }{m}$.
Pay attention to the units of measurement of resistivity, lengths and areas of conductors. For correct calculations, we will convert the length of each wire to SI (in $m^2$). Cross-sectional areas should be expressed in $mm^2$. For the third task, let's convert $cm^2$ to $cm^2$.
Given: $l_1 = 80 \space cm$ $l_2 = 400 \space cm$ $l_3 = 50 \space cm$ $S_1 = 0.2 \space mm^2$ $S_2 = 0.5 \space mm^2$ $S_3 = 0.005 \space cm^2 = 0.5 \space mm^2$ $\rho_1 = 0.028 \frac{Ohm \cdot mm^2}{m}$ $\rho_2 = 0.4 \frac{Ohm \cdot mm^2}{m} $ $\rho_3 = 0.5 \frac{Ohm \cdot mm^2}{m}$
SI: $l_1 = 0.8 \space m$ $l_2 = 4 \space m$ $l_3 = 0.5 \space m$
$R_1 — ?$ $R_2 — ?$ $R_3 — ?$
Show solution and answer
Hide
Solution:
The formula for calculating the resistance of a conductor is: $R = \frac{\rho l}{S}$. Let us calculate the resistance of each conductor from it.
$R_1 = \frac{\rho_1 l_1}{S_1}$, $R_1 = \frac{0.028 \frac{Ohm \cdot mm^2}{m} \cdot 0.8 \space m}{0.2 \space mm^2} = \frac{0.0224 \space Ohm}{0.2} = 0.112 \space Ohm$.
$R_2 = \frac{\rho_2 l_2}{S_2}$, $R_2 = \frac{0.4 \frac{Ohm \cdot mm^2}{m} \cdot 4 \space m}{0.5 \space mm^2} = \frac{1.6 \space Ohm}{0.5} = 3.2 \space Ohm$.
$R_3 = \frac{\rho_3 l_3}{S_3}$, $R_2 = \frac{0.5 \frac{Ohm \cdot mm^2}{m} \cdot 0.5 \space m}{0.5 \space mm^2} = \frac{0.25 \space Ohm}{0.5} = 0.5 \space Ohm$.
Answer: $R_1 = 0.112 \space Ohm$, $R_2 = 3.2 \space Ohm$, $R_3 = 0.5 \space Ohm$.
Exercise #3
The spiral of the electric hotplate is made of nichrome wire with a length of $13.75 \space m$ and a cross-sectional area of $0.1 \space mm^2$.
The tile is designed for a voltage of $220 \space V$. Determine the current strength in the tile spiral. The resistivity of nichrome is $1.1 \frac{Ohm \cdot mm^2}{m}$.
Given: $l = 13.75 \space m$ $S = 0.1 \space mm^2$ $U = 220 \space V$ $\rho = 1.1 \frac{Ohm \cdot mm^2}{m}$
$I — ?$
Show solution and answer
Hide
Solution:
We can calculate the current strength in the tile spiral using the formula of Ohm's law for a section of the circuit: $I = \frac{U}{R}$.
Let us calculate the unknown resistance of the nichrome wire using the formula $R = \frac{\rho l}{S}$. $R = \frac{1.1 \frac{Ohm \cdot mm^2}{m} \cdot 13.75 \space m}{0.1 \space mm^2} = \frac{15.125 \space Ohm}{0.1} = 151.25 \ space Ohm$.
Let us now calculate the current strength: $I = \frac{220 \space V}{151.25 \space Ohm} \approx 1.5 \space A$.
Answer: $I \approx 1.5 \space A$.
Why do metals have the lowest resistivities?
From the table above it can be seen that metals have the lowest resistivity values: silver, copper, gold, aluminum, etc. This property of metals is associated with a high concentration of free electrons, “not tied” to a specific atom, but wandering in the space of the crystal lattice. Voltage applied to the ends of a conductor creates an electric field that acts on the electrons, causing them to move in concert in the same direction.
Rice. 2. Electric current in metals, free electrons.
Silver has the lowest ρ value - 0.016 Ohm*mm2/m. But for widespread, mass use in power supply networks and equipment, this metal is not used due to its too high price. Silver is used to create the most critical contacts in special electrical devices. The following table shows the resistivity values of metals and alloys, commonly used metals in electrical engineering:
Table
Specific resistances of metals, Ohm*mm2/m
(at T = 200C)
| Silver | 0,016 | Bronze (alloy) | 0,1 |
| Copper | 0,017 | Tin | 0,12 |
| Gold | 0,024 | Steel (alloy) | 0,12 |
| Aluminum | 0,028 | Lead | 0,21 |
| Iridium | 0,047 | Nickelin (alloy) | 0,42 |
| Molybdenum | 0,054 | Manganin (alloy) | 0,45 |
| Tungsten | 0,055 | Constantan (alloy) | 0,48 |
| Zinc | 0,06 | Titanium | 0,58 |
| Brass (alloy) | 0,071 | Mercury | 0,958 |
| Nickel | 0,087 | Nichrome (alloy) | 1,1 |
| Platinum | 0,1 | Bismuth | 1,2 |
The most popular in electrical engineering are copper and aluminum. Copper and copper alloys are used to make cable products and shunts - parts that limit large currents through measuring instruments.
Effect of temperature on resistivity
In reference books, the values of ρ of metals are given at room temperature 200C. But experiments have shown that the dependence ρ(T) is linear and is described by the formula:
$ ρ(T) = ρ0 * (1 + α*T)$ (3),
where: ρ0 is the resistivity of the conductor at a temperature of 00C, α is the temperature coefficient of resistance, which is also individual for each substance. Values of α obtained experimentally can be found in reference books. Below are α values for some metals:
- Silver - 0.0035;
- Copper - 0.004;
- Aluminum - 0.004;
- Iron - 0.0066;
- Platinum - 0.0032;
- Tungsten - 0.0045.
Thus, as the temperature increases, the resistance of metals increases. This is explained by the fact that with increasing temperature, the number of defects in the crystal lattice increases due to more intense thermal vibrations of the ions, which inhibit the electron current.
Temperature dependence of resistivity of metals.
As the metal temperature approaches absolute zero, the resistivity drops sharply to zero. This phenomenon is called superconductivity, and materials that exhibit this ability are called superconductors. This effect was discovered in 1911 by the Dutch physicist Kamerlingh Onnes. In his experiment, the resistivity of mercury decreased to zero at 4.10K.
Conductivity and resistance
U.S. shows the ability of a substance to prevent the passage of current. But in physics there is also an inverse quantity - conductivity. It shows the ability to conduct electric current. It looks like this:
σ=1/ρ, where ρ is the resistivity of the substance.
If we talk about conductivity, it is determined by the characteristics of charge carriers in this substance. So, metals have free electrons. There are no more than three of them on the outer shell, and it is more profitable for the atom to “give them away,” which is what happens in chemical reactions with substances from the right side of the periodic table. In a situation where we have a pure metal, it has a crystalline structure in which these outer electrons are shared. They are the ones that transfer charge if an electric field is applied to the metal.
In solutions, charge carriers are ions.
If we talk about substances such as silicon, then in its properties it is a semiconductor and works on a slightly different principle, but more on that later. In the meantime, let’s figure out how these classes of substances differ:
- Conductors;
- Semiconductors;
- Dielectrics.
Conductors and dielectrics
There are substances that almost do not conduct current. They are called dielectrics. Such substances are capable of polarization in an electric field, that is, their molecules can rotate in this field depending on how electrons . But since these electrons are not free, but serve for communication between atoms, they do not conduct current.
The conductivity of dielectrics is almost zero, although there are no ideal ones among them (this is the same abstraction as an absolutely black body or an ideal gas).
The conventional limit of the concept of “conductor” is ρ<10^-5 Ohm, and the lower threshold of that for a dielectric is 10^8 Ohm.
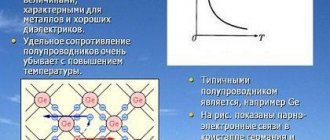
In between these two classes there are substances called semiconductors. But their classification into a separate group of substances is associated not so much with their intermediate state in the “conductivity-resistance” line, but with the features of this conductivity under different conditions.
Dependence on environmental factors
Conductivity is not a completely constant value. The data in the tables from which ρ is taken for calculations exists for normal environmental conditions, that is, for a temperature of 20 degrees. In reality, it is difficult to find such ideal conditions for the operation of a circuit; actually US (and therefore conductivity) depend on the following factors:
- temperature;
- pressure;
- presence of magnetic fields;
- light;
- state of aggregation.
Different substances have their own schedule for changing this parameter under different conditions. Thus, ferromagnets (iron and nickel) increase it when the direction of the current coincides with the direction of the magnetic field lines. As for temperature, the dependence here is almost linear (there is even a concept of temperature coefficient of resistance, and this is also a tabular value). But the direction of this dependence is different: for metals it increases with increasing temperature, and for rare earth elements and electrolyte solutions it increases - and this is within the same state of aggregation.
For semiconductors, the dependence on temperature is not linear, but hyperbolic and inverse: with increasing temperature, their conductivity increases. This qualitatively distinguishes conductors from semiconductors. This is what the dependence of ρ on temperature for conductors looks like:
The resistivities of copper, platinum and iron are shown here. Some metals, for example, mercury, have a slightly different graph - when the temperature drops to 4 K, it loses it almost completely (this phenomenon is called superconductivity).
And for semiconductors this dependence will be something like this:
Upon transition to the liquid state, the ρ of the metal increases, but then they all behave differently. For example, for molten bismuth it is lower than at room temperature, and for copper it is 10 times higher than normal. Nickel leaves the linear graph at another 400 degrees, after which ρ falls.
But tungsten has such a high temperature dependence that it causes incandescent lamps to burn out. When turned on, the current heats the coil, and its resistance increases several times.
Also y. With. alloys depends on the technology of their production. So, if we are dealing with a simple mechanical mixture, then the resistance of such a substance can be calculated using the average, but for a substitution alloy (this is when two or more elements are combined into one crystal lattice) it will be different, as a rule, much greater. For example, nichrome, from which spirals for electric stoves are made, has such a value for this parameter that when connected to the circuit, this conductor heats up to the point of redness (which is why, in fact, it is used).
Here is the characteristic ρ of carbon steels:
As can be seen, as it approaches the melting temperature, it stabilizes.
Properties of resistive materials
The resistivity of a metal depends on temperature. Their values are usually given for room temperature (20°C). The change in resistivity as a result of a change in temperature is characterized by a temperature coefficient.
For example, thermistors (thermistors) use this property to measure temperature. On the other hand, in precision electronics, this is a rather undesirable effect. Metal film resistors have excellent temperature stability properties. This is achieved not only due to the low resistivity of the material, but also due to the mechanical design of the resistor itself.
Many different materials and alloys are used in the manufacture of resistors. Nichrome (an alloy of nickel and chromium), due to its high resistivity and resistance to oxidation at high temperatures, is often used as a material for making wirewound resistors. Its disadvantage is that it cannot be soldered. Constantan, another popular material, is easy to solder and has a lower temperature coefficient.
High conductivity materials
The most widespread materials of high conductivity include copper and aluminum (Superconducting materials, which have a typical resistance 10-20 times lower than ordinary conductive materials (metals), are discussed in the section Superconductivity).
Copper
The advantages of copper, which ensure its widespread use as a conductor material, are as follows:
- low resistivity;
- sufficiently high mechanical strength;
- corrosion resistance is satisfactory in most applications;
- good workability: copper is rolled into sheets, strips and drawn into wire, the thickness of which can be increased to thousandths of a millimeter;
- relative ease of soldering and welding.
Copper is most often obtained by processing sulfide ores. After a series of ore smelting and roasting with intense blasting, copper intended for electrical purposes must undergo a process of electrolytic purification.
Copper grades M1 and M0 are most often used as conductor material. M1 grade copper contains 99.9% Cu, and in the total amount of impurities (0.1%) oxygen should be no more than 0.08%. The presence of oxygen in copper worsens its mechanical properties. The best mechanical properties are found in M0 grade copper, which contains no more than 0.05% impurities, including no more than 0.02% oxygen.
Copper is a relatively expensive and scarce material, so it is increasingly being replaced by other metals, especially aluminum.
In some cases, alloys of copper with tin, silicon, phosphorus, beryllium, chromium, magnesium, and cadmium are used. Such alloys, called bronzes, with the correct composition, have significantly higher mechanical properties than pure copper.
Aluminum
Aluminum is the second most important conductor material after copper. This is the most important representative of the so-called light metals: the density of cast aluminum is about 2.6, and rolled aluminum is 2.7 Mg/m3. Thus, aluminum is approximately 3.5 times lighter than copper. The temperature coefficient of expansion, specific heat capacity and heat of fusion of aluminum are greater than those of copper. Due to the high values of specific heat capacity and heat of fusion, heating aluminum to the melting point and transferring it to a molten state requires more heat than heating and melting the same amount of copper, although the melting point of aluminum is lower than that of copper.
Aluminum has lower properties compared to copper - both mechanical and electrical. With the same cross-section and length, the electrical resistance of an aluminum wire is 1.63 times greater than that of a copper wire. It is very important that aluminum is less scarce than copper.
For electrical purposes, aluminum containing no more than 0.5% impurities, grade A1, is used. Even purer AB00 grade aluminum (no more than 0.03% impurities) is used for the manufacture of aluminum foil, electrodes and housings of electrolytic capacitors. Aluminum of the highest purity AB0000 has an impurity content of no more than 0.004%. Additives of Ni, Si, Zn or Fe at a content of 0.5% reduce the γ of annealed aluminum by no more than 2-3%. A more noticeable effect is exerted by Cu, Ag and Mg impurities, which, at the same mass content, reduce γ aluminum by 5-10%. Ti and Mn greatly reduce the electrical conductivity of aluminum.
Aluminum oxidizes very actively and becomes covered with a thin oxide film with high electrical resistance. This film protects the metal from further corrosion.
It will be interesting➡ The strength of electric current and everything about it
Aluminum alloys have increased mechanical strength. An example of such an alloy is Aldrey, containing 0.3-0.5% Mg, 0.4-0.7% Si and 0.2-0.3% Fe. In aldrey, the Mg2Si compound is formed, which imparts high mechanical properties to the alloy.
Iron and steel
Iron (steel), as the cheapest and most accessible metal, which also has high mechanical strength, is of great interest for use as a conductor material. However, even pure iron has a significantly higher resistivity compared to copper and aluminum; ρ steel, i.e. iron mixed with carbon and other elements is even higher. Ordinary steel has low corrosion resistance: even at normal temperatures, especially in conditions of high humidity, it quickly rusts; As the temperature rises, the corrosion rate increases sharply. Therefore, the surface of steel wires must be protected by a layer of more resistant material. Zinc coating is usually used for this purpose.
In some cases, to reduce the consumption of non-ferrous metals, the so-called bimetal is used. It is steel coated on the outside with a layer of copper, with both metals connected to each other firmly and continuously.
Sodium
Sodium metal is a very promising conductor material. Sodium can be obtained by electrolysis of molten sodium chloride NaCl in virtually unlimited quantities. From a comparison of the properties of sodium with the properties of other conductor metals, it is clear that the resistivity of sodium is approximately 2.8 times greater than ρ of copper and 1.7 times greater than ρ of aluminum, but due to the extremely low density of sodium (its density is almost 9 times less than the density of copper), a wire made of sodium for a given conductivity per unit length should be significantly lighter than a wire made of any other metal. However, sodium is extremely active chemically (it oxidizes intensely in air and reacts violently with water), which is why the sodium wire must be protected with a sealing sheath. The sheath must give the wire the necessary mechanical strength, since sodium is very soft and has a low tensile strength during deformation.
What is the resistance of copper wire
In metals, a current is formed when an electric field appears. It “forces” electrons to move in an orderly manner, in one direction. Electrons from the distant orbits of an atom, weakly held by the nucleus, form a current.
Copper wires
As negative particles pass through the crystal lattice of copper molecules, they collide with atoms and other electrons. There is an obstacle or resistance to the directional movement of particles.
To evaluate the resistance to current, the value of “electrical resistance” or “electrical impedance” was introduced. It is designated by the letter “R” or “r”. Resistance is calculated using Georg Ohm's formula: R=, where U is the potential difference or voltage acting on a section of the circuit, I is the current strength.
Concept of resistance
Important! The higher the impedance value of a metal, the less current passes through it, and it is copper conductors that are so widespread in electrical engineering due to this property.
Based on Ohm's formula, the magnitude of the current is affected by the applied voltage at a constant R. But the resistance of copper wires varies depending on their physical characteristics and operating conditions.
Application of copper conductors
Copper is not only a good conductor of electric current, but also a very ductile material. Thanks to this property, copper wiring fits better and is resistant to bending and stretching.
Copper is in great demand on the market. Many different products are made from this material:
- A huge variety of conductors;
- Auto parts (eg radiators);
- Clock mechanisms;
- Computer components;
- Parts of electrical and electronic devices.
The electrical resistivity of copper is one of the best among current-conducting materials, so many electrical industry products are created based on it. In addition, copper is easy to solder, so it is very common in amateur radio.
The high thermal conductivity of copper allows it to be used in cooling and heating devices, and its plasticity makes it possible to create the smallest parts and the thinnest conductors.
What affects the resistance of a copper wire
The electrical impedance of a copper cable depends on several factors:
- Specific resistance;
- Wire cross-sectional area;
- Wire lengths;
- External temperature.
The last point can be neglected in conditions of domestic use of the cable. A noticeable change in impedance occurs at temperatures above 100°C.
Resistance dependence
Resistivity in the SI system is denoted by the letter ρ. It is defined as the resistance value of a conductor having a cross-section of 1 m2 and a length of 1 m, measured in Ohm ∙ m2. This dimension is inconvenient in electrical calculations, so the unit of measurement Ohm ∙ mm2 is often used.
Important! This parameter is a characteristic of the substance - copper. It does not depend on the shape or cross-sectional area. The purity of the copper, the presence of impurities, the method of making the wire, and the temperature of the conductor are factors that affect the resistivity.
The dependence of the parameter on temperature is described by the following formula: ρt= ρ20[1+ α(t−20°C)]. Here ρ20 is the resistivity of copper at 20°C, α is an empirically found coefficient, from 0°C to 100°C for copper it has a value equal to 0.004 °C-1, t is the temperature of the conductor.
Below is a table of ρ values for different metals at a temperature of 20°C.
Resistivity table
According to the table, copper has a low resistivity, lower only for silver. This ensures good conductivity of the metal.
The thicker the wire, the lower its resistance. The dependence of R of the conductor on the cross-section is called “inversely proportional”.
Important! As the cross-sectional area of the cable increases, it is easier for electrons to pass through the crystal lattice. Therefore, with increasing load and increasing current density, the cross-sectional area should be increased.
An increase in the length of a copper cable entails an increase in its resistance. Impedance is directly proportional to the length of the wire. The longer the conductor, the more atoms there are in the path of free electrons.
conclusions
The last element that affects the resistance of copper is the temperature of the environment. The higher it is, the greater the amplitude of movement of the atoms of the crystal lattice. Thus, they create an additional obstacle for electrons participating in directed movement.
Important! If you lower the temperature to absolute zero, which has a value of 0° K or -273°C, then the opposite effect will be observed - the phenomenon of superconductivity. In this state, the substance has zero resistance.
Temperature correlation
Table of electrical resistivity of some metals
| Wire type | ρ at 20℃, Ohm-m |
| Silver | 1,59×10⁻⁸ |
| Copper | 1,67×10⁻⁸ |
| Gold | 2,35×10⁻⁸ |
| Aluminum | 2,65×10⁻⁸ |
| Tungsten | 5,65×10⁻⁸ |
| Nickel | 6,84×10⁻⁸ |
| Iron | 9,7×10⁻⁸ |
| Platinum | 1,06×10⁻⁷ |
| Steel | 1,6×10⁻⁷ |
| Lead | 2,06×10⁻⁷ |
| Duralumin | 4,0×10⁻⁷ |
| Nichrome | 1,05×10⁻⁶ |
The resistivity is absolutely independent of the shape and size of the conductor, but varies over a wide range when the temperature deviates from the standard value of 20 degrees Celsius. Practical electrical engineering has proven that an increase in temperature increases the resistance of metals to current flow; on the other hand, along with a decrease in temperature, it decreases. It is possible to approximately calculate how significant the change will be, taking into account the fact that all metals have almost the same level of increase in loss of a given value, on average 0.4% per 1°C.
Resistance chart
If this indicator needs to be determined accurately, then you can use this formula:
ρ = ρ0 x (1 + α x (t - t))
, where ρ and ρ0 are, respectively, resistivities at temperatures t and t (20°C, table value), α is the temperature coefficient of resistance.
| Wire type | α |
| Nickel | 0,005866 |
| Iron | 0,005671 |
| Molybdenum | 0,004579 |
| Tungsten | 0,004403 |
| Aluminum | 0,004308 |
| Copper | 0,004041 |
| Silver | 0,003819 |
| Platinum | 0,003729 |
| Gold | 0,003715 |
| Zinc | 0,003847 |
| Steel | 0,003 |
| Nichrome | 0,00017 |
So, for example, having found in the tables the resistivity of copper at 20 degrees Celsius and its temperature coefficient, you can calculate that when heated to 100℃ its resistance will increase by 32%. Almost the same thing will happen with the resistivity of an aluminum cable with the same coefficient (0.004). But the resistivity of steel will increase less significantly - by 24%.
Heat
As the temperature increases, the conductor is saturated with thermal energy, which is transferred to all atoms of the substance. This causes an increase in the intensity of their thermal movement. The latter factor leads to an increase in resistance to the movement of free electrons in a certain direction, since the probability of free electrons meeting atoms increases. When the temperature decreases, fewer atoms can impede the directional movement of electrons, hence the opposite occurs. As a result of a colossal drop in temperature, an interesting phenomenon occurs, called “superconductivity of metals”: resistance decreases to zero in conditions close to absolute zero (-273.15℃). In such conditions, the metal atoms freeze in their positions, and the electrons move without any obstacles.
Superconductivity
Comparison of conductivity of different types of steel
The characteristics of steel depend on its composition and temperature:
- For carbon alloys, the resistance is quite low: it is 0.13-0.2 μOhm/m. The higher the temperature, the greater the value;
- Low-alloy alloys have a higher resistance - 0.2-0.43 μOhm/m;
- High-alloy steels have high resistance - 0.3-0.86 μOhm/m;
- Due to the high chromium content, the resistance of chromium stainless alloys is 0.5-0.6 μOhm/m;
- Chromium-nickel austenitic steels are stainless and, thanks to nickel, have a high resistance of 0.7-0.9 μOhm/m.
Copper ranks second in terms of electrical conductivity: it perfectly passes electric current and is widely used in the manufacture of wires. Aluminum is also used no less often: it is weaker than copper, but cheaper and lighter.
Active resistance of wires, cables and lines
Due to the fact that alternating current flows unevenly, under the same conditions, alternating and direct current R will be different. As already mentioned, steel electrical wires have a better active R compared to conductors made of non-ferrous metals, which have the same R at any current strength.
On the contrary, the active R of steel electrical cables always depends on the electric current, so DC conductivity is never used in this case. The active R of an electrical cable is determined using the formula: R=l/y*s.
Formulas for calculating electrical resistance
Let's consider formulas for different types of resistance.
Active Resistance Formula
As follows:
\[ Im=Um/R \]
Inductive reactance formula
Look like:
\[ Xl=2пFL \]
L represents the AC current rating.
F represents frequency
Capacitance formula
A conductor connected directly to an electrical circuit, which has no resistance, but provides the presence of capacitance.
The formula looks like this:
\[ Xc = 1/ωC \]
- ω is an indicator of cyclic frequency.
- C capacity indicator.
Determination of impedance
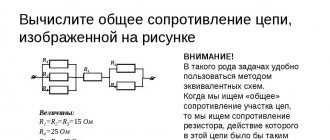
To obtain this value, you will need to take into account the resistance of all sections.
Looks like that:
\[ R=R1+R2 \]
In this case, there may be several areas.