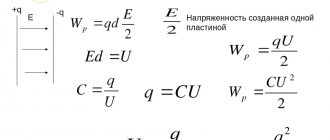In electrical circuits, various methods of connecting capacitors are used. The connection of capacitors can be made: series , parallel and series-parallel (the latter is sometimes called a mixed connection of capacitors). Existing types of capacitor connections are shown in Figure 1.
Figure 1. Methods for connecting capacitors.
Parallel connection of capacitors.
If a group of capacitors is included in the circuit in such a way that the plates of all capacitors are directly connected to the switching points, then such a connection is called a parallel connection of capacitors (Figure 2.).
Figure 2. Parallel connection of capacitors.
When charging a group of capacitors connected in parallel, there will be the same potential difference between the plates of all capacitors, since they are all charged from the same current source. The total amount of electricity on all capacitors will be equal to the sum of the amounts of electricity placed on each of the capacitors, since the charge of each capacitor occurs independently of the charge of other capacitors in this group. Based on this, the entire system of parallel-connected capacitors can be considered as one equivalent (equal) capacitor. Then the total capacitance of the capacitors in a parallel connection is equal to the sum of the capacitances of all connected capacitors.
Let us denote the total capacitance of the capacitors connected to the battery by the letter Ctot, the capacitance of the first capacitor C1, the capacitance of the second C2 and the capacitance of the third C3. Then for parallel connection of capacitors the following formula will be valid:
The last + sign and the ellipsis indicate that this formula can be used with four, five, and generally any number of capacitors.
Capacitors - tasks
In problems involving capacitors, you often need to be able to find the capacitance of series and parallel connections of capacitors. With the parallel connection of containers, everything is simple: with a parallel connection, the containers are folded. Why is this so? The fact is that with a parallel connection, all capacitors have the same voltage, and their charges are proportional to the capacitances:
etc.
Total charge on capacitors:
Then the capacitance of the capacitor system is: .
With a serial connection everything is more complicated. By the way, when I was preparing this article, I came across this gem that I couldn’t help but quote:
The charges in a series connection are the same on all capacitors:
etc.
Then the voltage between the extreme points is:
Capacity of the entire system: , or
1. The potential difference between points A and B B. The capacitance of the capacitors is equal to μF and μF, respectively. Determine the charges and potential differences on the plates of the first and second capacitors.
Problem 1
Let us determine the total capacity of such a connection: ,
µF (we immediately count in microfarads, so as not to describe powers of ten)
Then the charge is equal to:
µC
When connected in series, the charges on all capacitors are the same, which means for the first:
, for the second.
Capacitor voltages:
V, V.
Answer: 18 µC, 6 V, 3 V. 2. Determine the capacity of the capacitor bank shown in the figure. The capacitance of each capacitor is µF.
Problem 2
Capacitances and, since they are connected in parallel, must be added: µF. Then we get a series connection of three identical capacitors and , the capacity of which is twice as large. When connecting containers in series, their equivalent capacity is calculated by the formula: . Then we get: , µF.
Answer: 286 nF
3. The capacity of a capacitor bank formed by two capacitors connected in series is 100 pF, and the charge is 20 nC. Determine the capacitance of the second capacitor, as well as the potential difference across the plates of each of them, if pF.
Let's use it. Then (we count in picofarads)
Hence , , pF.
When connected in series, the charge on the capacitors is equal: nC.
The voltages will also be the same:
V, V.
Answer: B, nCl.
4. The largest capacitance of the capacitor is 60 µF. What charge will it accumulate when connected to a 60V DC source?
Since , then mC.
Answer: 0.36 mC
5. When a solid dielectric was introduced into the space between the plates of an air capacitor, the voltage across the capacitor decreased from 400 to 100 V. What is the dielectric constant of the dielectric?
Let us first consider the capacitor before introducing the dielectric: , . The charge remained the same when the dielectric was introduced, but the voltage changed: .
Then, or
The capacitance of a capacitor, as is known, depends on the area of the plates and the distance between them, as well as on the dielectric:
In the first case, without a dielectric: , and in the second
Let us find in this case the ratio of capacities: , or
Answer: Cl*Cl/N*m*m
6. The area of the capacitor plates is 520 cm2. At what distance should the plates be placed in the air so that the capacitance of the capacitor is equal to 50 pF?
Capacitance of a capacitor without dielectric:
The area must be expressed in square meters. meters:
Electrical constant.
Let us express from the first expression the value we need: m, or 9.2 mm.
Answer: 9.2 mm
7. A 20 µF capacitor was given a charge of 5 µC. What is the energy of a charged capacitor?
The electric field energy accumulated by the capacitor can be calculated using the formula:
– and in this task it would be good to use the second entry.
Then: µJ
8. The distance between the plates of a flat capacitor with a dielectric made of paper impregnated with paraffin is 2 mm, and the voltage is 200 V. Find the field energy density.
To find the energy density, you must first determine the field energy and then calculate in what volume it is concentrated.
, on the other hand, capacity , then . The volume of space between the plates of a capacitor is the product of the area of the plates and the distance between them: . Then the field energy density is equal to
Let's look in the reference book to see what the dielectric constant of paper with paraffin is, express the distance between the plates in meters, and substitute the numbers:
mJ
Series connection of capacitors.
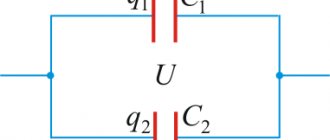
If the connection of capacitors into a battery is made in the form of a chain and the plates of only the first and last capacitors are directly connected to the connection points in the circuit, then such a connection of capacitors is called serial ( Figure 3).
Figure 2. Series connection of capacitors.
When connected in series, all capacitors are charged with the same amount of electricity, since only the outer plates (1 and 6) are charged directly from the current source, and the remaining plates (2, 3, 4 and 5) are charged through influence. In this case, the charge of plate 2 will be equal in magnitude and opposite in sign to the charge of plate 1, the charge of plate 3 will be equal in magnitude and opposite in sign to the charge of plate 2, etc.
The voltages on different capacitors will, generally speaking, be different, since charging capacitors of different capacities with the same amount of electricity always requires different voltages. The smaller the capacitance of the capacitor, the greater the voltage required in order to charge this capacitor with the required amount of electricity, and vice versa.
Thus, when charging a group of capacitors connected in series, the voltages on small capacitors will be greater, and on large capacitors - less.
Similar to the previous case, we can consider the entire group of capacitors connected in series as one equivalent capacitor, between the plates of which there is a voltage equal to the sum of the voltages on all capacitors of the group, and the charge of which is equal to the charge of any of the capacitors of the group.
Let's take the smallest capacitor in the group. There should be the greatest tension on it. But the voltage across this capacitor is only a fraction of the total voltage that exists across the entire group of capacitors. The voltage across the entire group is greater than the voltage across the capacitor with the smallest capacitance. And from here it directly follows that the total capacitance of a group of capacitors connected in series is less than the capacitance of the smallest capacitor in the group.
To calculate the total capacitance when connecting capacitors in series, it is most convenient to use the following formula:
For the special case of two series-connected capacitors, the formula for calculating their total capacitance will be:
Problems on capacitors and electrical capacity with solutions
If you don't know how to solve problems with capacitors, first look at the theory and remember the physics problem solving guide and useful formulas.
Problem No. 1 on the electrical capacity of a capacitor bank
Condition
A flat capacitor with a capacity of 16 μF is cut into 4 equal parts along planes perpendicular to the plates. The resulting capacitors are connected in series. What is the capacitance of a capacitor bank?
Solution
It follows from the condition that the area of the resulting capacitors is 4 times smaller than that of the original one. Knowing this, you can find the capacitance of each resulting capacitor:
Connecting 4 such capacitors in series, we get:
Answer: 1 µF.
Problem No. 2 on the energy of a flat capacitor
Condition
A flat capacitor is filled with a dielectric with a dielectric constant of 2. The energy of a capacitor without a dielectric is 20 μJ. What is the energy of the capacitor after filling with a dielectric? Assume that the power supply is disconnected from the capacitor.
Solution
The energy of the capacitor before filling with a dielectric is equal to:
After filling, the capacitance of the capacitor will change:
Capacitor energy after filling:
Answer: 40 µF.
Task No. 3 on series and parallel connection of capacitors
Condition
The figure shows a bank of capacitors. Each capacitor has a capacity of 1 µF. Find the battery capacity.
Solution
As you can see, some of the capacitors are connected in parallel, and some in series. This is a typical example of a mixed capacitor connection. The algorithm for solving problems with a mixed connection of capacitors comes down to simplifying the circuit and reducing everything to just a parallel or series connection.
Capacitors 3 and 4 are connected in parallel. Adding up their capacitance, we end up with a series connection of four capacitors: 1, 2, 5 and 3-4. For parallel connection:
For serial connection:
Answer: 0.285 µF.
Problem No. 4 on the passage of a particle in a capacitor
The capacitor charge is 0.3 nC, and the capacitance is 10 pF. What speed does an electron acquire when flying in a capacitor from one plate to another? The initial speed of the electron is zero.
Solution
According to the law of conservation of energy, the difference between the kinetic energies of the electron at the beginning and end of the path will be equal to the work of the field in moving it. According to the condition, the initial kinetic energy of the electron is 0. Let us write:
Taking this into account, we get:
Answer: 10^7 m/s.
Problem No. 5 to calculate the energy of the electric field of a capacitor
Condition
The capacitor is connected to a constant voltage source U=1 kV. The capacitance of the capacitor is 5 pF. How does the charge on the capacitor plates and its energy change if the distance between the plates is reduced by three times.
Solution
The capacitor charge is equal to:
The change in charge will be equal to:
Energy Change:
Answer: 5 µJ.
Series-parallel (mixed) connection of capacitors
A series-parallel connection of capacitors is a circuit that includes sections with both parallel and series connections of capacitors.
Figure 4 shows an example of a circuit section with a mixed connection of capacitors.
Figure 4. Series-parallel connection of capacitors.
When calculating the total capacitance of such a section of a circuit with a series-parallel connection of capacitors, this section is divided into the simplest sections, consisting only of groups with series or parallel connection of capacitors. Next, the calculation algorithm looks like:
1. Determine the equivalent capacitance of sections with series connection of capacitors.
2. If these sections contain series-connected capacitors, then first calculate their capacitance.
3. After calculating the equivalent capacitances of the capacitors, redraw the circuit. Typically, a circuit of equivalent capacitors connected in series is obtained.
4. Calculate the capacity of the resulting circuit.
One example of calculating capacitance for a mixed connection of capacitors is shown in Figure 5.
Figure 5. An example of calculating a series-parallel connection of capacitors.
More information about calculating the connection of capacitors can be found in the multimedia textbook on the basics of electrical engineering and electronics:
DID YOU LIKE THE ARTICLE? SHARE WITH YOUR FRIENDS ON SOCIAL NETWORKS!
Related materials:
- Electrical capacity
- Capacitor capacity
- Capacitor field energy
- Types of capacitors
- Capacitor designation
Comments
#20 Vladimir Nikolaevich 10/06/2019 09:48 In fact, if you connect 3 capacitors in series and each is 3 microfarads, and the voltage in the network is 100 volts, then the output will be 1 microforad and 300 volts. If in parallel, then the capacity will be 9 micro farads and the voltage will be 100 volts
Quote
#19 AlexeyDu 09/19/2019 07:03 In practice, as a rule, there is not exactly 110V at both ends, but approximately 120V at one end and 100V at the other, respectively, it is better to ground the end with the lower voltage and take it as zero. You can determine the end with less voltage using a simple indicator screwdriver with a tip with a closing thumb, i.e. the end that will glow with less brightness and must be taken as zero. If you cannot determine the difference in the indicator light, then you have an ideal generator and can ground either end of the outlet. I hope I helped you.
Quote
#18 AlexeyDu 09/19/2019 07:02 I quote Volodya:
I bought a gas generator, but the light blinks! and the stabilizer doesn't help. Maybe capacitors will help the problem? Thank you
If the gas generator is single-phase and has alternating voltage, then it operates on the principle of two half-cycles, i.e.
at each end (phase and zero) there is a voltage of 110V, respectively, in total they give 220V in the socket (with a frequency of 50 Hz, which you observe as a light bulb blinking). To get rid of the blinking effect, it is necessary to connect the generator housing and one end of the socket to a grounding circuit, then at the grounded end the voltage will become zero (0V), and at the other it will become equal to 220 (220V) of a pure sine wave and, accordingly, the grounded end will become zero, and unconnected phase. Quote #17 Denis25 10/09/2018 17:20 I quote Volodya:
I bought a gas generator, but the light blinks! and the stabilizer doesn't help. Maybe capacitors will help the problem? Thank you
Only an online type inverter will help.
Quote #16 Tram 03/26/2018 14:42 excellent article! Everything is straight forward, with clear examples. Thanks a lot)
Quote
#15 Topic 01/10/2017 18:23 What will be the total (equivalent) electrical capacitance if two 10F capacitors are connected in parallel in an electrical circuit: 5F? 10F? 15F? 20F?
Quote
#14 Ivan 07.25.2016 16:19 With a series connection, the capacitance of the capacitor does not increase, and in this case, 1+1+1=1 in series because the voltage supplied to this assembly increases!!!!
Quote
#13 Administrator 09.30.2015 15:05 I quote Humanoid:
Class. how much will it be if you connect three conducers in parallel at 1 μF = 3 μF, and how much will it be if you connect them in series 1/total=1/1+1/1+1/1=1+1+1= = 3 μF some kind of noodle
Interesting arithmetic, of course I apologize, but where is this taught?
Can I count, remembering third grade mathematics: 1/Tot = 1/1+1/1+1/1=1+1+1= = 3 that is, 1/Tot = 3 Now let’s express Tot = 1/3 = 0.3333333333 etc. In my opinion, it couldn’t be simpler. I think it is not electrical engineering that is to blame, but mathematics. So they came up with the idea of dividing... multiplying... So where are the noodles...? I’m sorry, I didn’t read the name. You’re a HUMANOID, then everything is clear! Quote #12 Humanoid 09.29.2015 22:44 Cool. how much will it be if you connect three conducers in parallel at 1 μF = 3 μF, and how much will it be if you connect them in series 1/total=1/1+1/1+1/1=1+1+1= = 3 μF some kind of noodle
Quote
#11 Andey 12/14/2014 11:32 Normal explanation
Quote
#10 Administrator 12/11/2014 19:59 Do I understand the alternator? If so, then the capacitors have nothing to do with it. The light bulb may blink due to the fact that you have not set the nominal engine speed, so the current frequency is less than 50 Hz, that is, it will be noticeable to the eye as blinking. I think we need to turn up the speed
Quote
Volodya 12/11/2014 19:02 I bought a gas generator, but the light is blinking! and the stabilizer doesn't help. Maybe capacitors will help the problem? Thank you
Quote
Larisa 04/02/2014 16:48 thank you!!! great, but they explained it!!!
Quote
Sergey 02/19/2014 07:20 The 3rd formula somehow doesn’t work well with the 2nd. The 2nd one turns out much more. Or am I confusing something?
Quote
Just Dmitry 02/11/2014 17:47 thank you for the information, this is exactly what I needed, otherwise there are no radio stores in my city, but I needed to get 400 from 470 microfarads, and in theory, using the formula, this is possible! author respect to you
Quote
Crater-2 12/12/2013 11:12 simply more intelligible than in institute textbooks
Quote
Vitalik 12/09/2013 18:16 but in my opinion all the formulas are the same except for the symbols themselves, like C1, like R1, and so on.
Quote
xXx 12/05/2013 07:05 if you think about it, it’s not that difficult
Quote
Power 04.12.2013 05:59 what a weak technician you have
Quote
Danilp 12/04/2013 05:35 Cool article, read it, understood it and got 10 in the technical department
Quote
Update list of comments