We know that a body can be given a charge. If you do not touch the body after this, the resulting charge will remain on this body, that is, it will not move.
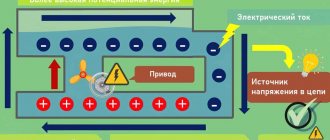
But if you make the charge move, you can observe interesting phenomena. Because it is moving charges that create:
- performing work with electric motors;
- heat generation in electric heating devices;
- glow of incandescent lamps.
Rice. 1. What happens due to the movement of charges
Speed of thermal movement of free electrons
We know that the total charge of a body consists of a large number of elementary charges.
For example, in solids, positive charges are the nuclei of atoms, or ions. And negative ones are electrons.
And in liquids or gases, positive and negative charges are ions.
Note: An ion is an atom that has an excess of electrons, or, conversely, fewer electrons than a neutral atom.
Let's consider a solid conductor; it contains free charges. These are electrons that have broken away from their atom and travel freely throughout the entire volume of the conductor.
Rice. 2. Differences between free and bound electrons in a conductor
Note: A conductor is a body in which there are many free electrons.
As is known from molecular kinetic theory (MKT), the smallest particles of matter are in continuous motion. This movement occurs under the influence of temperature, therefore, it is often called thermal. Such movement is disordered, that is, chaotic.
Rice. 3. Under the influence of temperature, free charges move randomly
Let's calculate the speed at which electrons in a conductor move randomly under the influence of temperature.
To do this, we use the formula for the root-mean-square velocity of particles from molecular physics:
\[\large v = \sqrt{\frac{3kT}{m}}\]
Let's substitute the following numerical values into the formula:
\(\large T = 300 \left( K\right)\) – room temperature +27 degrees Celsius;
\(\large k = 1.38 \cdot 10^{-23} \left( \frac{\text{J}}{K}\right) \) – Boltzmann constant;
\(\large m = 9.1 \cdot 10^{-31} \left(\text{kg}\right) \) – electron mass;
After calculations we obtain a speed approximately equal to
\[\large v \approx 117 \left(\frac{\text{km}}{\text{sec}}\right) \]
As you can see, this is a very high speed, more than 100 kilometers per second.
Rice. 4. Speed of free electrons in copper
Note: Physicists consider free electrons in a conductor to be particles of an ideal gas. This is what they call electron gas.
However, I would like to emphasize once again that thermal motion is chaotic. With the help of such a movement, an electric current cannot be created. Because current is the directional movement of charges.
Conductors and dielectrics
Some divide the world into black and white, while we divide it into conductors and dielectrics.
- Conductors are materials that conduct electric current. The best conductors are metals.
- Dielectrics are materials that do not conduct electric current. Easy!
| Conductors | Dielectrics |
| Copper, iron, aluminum, tin, lead, gold, silver, chromium, nickel, tungsten | Air, distilled water, polyvinyl chloride, amber, glass, rubber, polyethylene, polypropylene, polyamide, dry wood, rubber |
Just because a dielectric doesn't conduct electricity doesn't mean it can't store charge. The accumulation of charge does not depend on the ability to transfer it.
What is electric current
Electric current is the directed movement of electric charges.
Negative charges - electrons - move in metal conductors.
And in other conductors, for example, in liquid electrolytes, positive and negative ions can move directionally.
Inside semiconductors, charge is carried by electrons and holes.
Note: A hole is a pseudoparticle, a vacant place for an electron. It has a positive charge and can be considered as a bubble in an electron gas.
Rice. 5. In various environments, electric current is created by such charges
We see that electric current can be created by the movement of both positive and negative particles.
In this case, positive particles will be attracted to the negative pole of the current source and move along the circuit towards it.
And negative particles will be attracted and move towards the positive pole of the current source.
Note: To determine the direction of movement of charged particles, you can use the analogy with the flow of water: Charges, like water, move from where there are many of them to where there are few of them. At the dawn of the study of electricity, it was believed that during the flow of current in bodies, some kind of electrical fluid flows. Therefore, for electric current, an analogy with the flow of water is used. Later it turned out that there was no electrical liquid in the bodies.
If the charges move in a direction, then the current will have a direction.
Where is the current directed?
How to choose the direction of electric current? Which particles – positive or negative – should we focus on the movement of? It turns out that the direction of the current is a conditional choice.
Physicists agreed that the direction of electric current coincides with the direction of movement of positive charges. This means that the current is directed from the “+” to the “-” terminal of the current source.
Let the direction of the electric field strength vector \(\large \vec{E} \) be known. To determine the direction of the current, we must assume that positively charged particles are moving in this field.
Positive charges will move in the direction of the vector \(\large \vec{E} \), and negative charges will move towards the vector.
Rice. 6. Where is the current directed?
Note: In metals, electrons move from minus to plus, and current flows from plus to minus
Rice. 7. Direction of movement of charges and electric field strength vector
Note: The presence of directional movement of charges can be determined indirectly. Flowing through a conductor, current affects this conductor. The thermal, chemical, or magnetic effects of current are known.
The greater the current, that is, the stronger it is, the more noticeable its effect.
Conversion of internal energy into electrical energy
Now let's consider the transformation of internal energy into electrical energy. To do this, take two wires and solder them to each other. And then we heat this junction (Figure 5).
Figure 5. Conversion of internal energy into electrical energy
As a result of this heating, an electric current will arise in the wire. The device connected to our wire is called a galvanometer. We will look at the principle of its operation later, but for now we will use this device to determine the presence of electric current in a conductor. The arrow deviates - there is current, the arrow remains in place - there is no current.
Such a current source, consisting of a heater and the junction of wires made of different metals, is called a thermoelement .
In thermoelements, internal energy is converted into electrical energy.
What is the cross section of a conductor
Electric current is free charges moving directionally along a conductor. It can be determined when the number of charged particles passing through the conductor is known.
The conductor can be quite long. Therefore, it is inconvenient to take into account the charges located throughout the entire length of the conductor.
To make it easier to calculate the number of charges, select a point on the conductor in any convenient place.
A plane is mentally drawn through this point, positioning it perpendicular to the conductor. Since this plane in the conductor limits the area S, it is often called the cross-sectional area of the conductor.
To calculate the current strength, the charges passing through this section are counted.
How to calculate cross-sectional area
We will consider the conductor to be a round tube, by analogy with a pipe through which liquid flows. Using this analogy, we also assume that charges will move inside such a tube; they are indicated by circles in the figure.
Rice. 8. What is a cross section
Let's select a point on the pipe. Mentally cut a piece of pipe, making the cut perpendicularly. The walls of the tube at the cut point are the boundary of the circle.
The area of the resulting circle can be calculated using the following geometric formula:
\[\large \boxed{ S_{0} = \pi \cdot \frac{D^{2}}{4} = \pi \cdot R^{2} }\]
\(\large S_{0} \left( \text{m}^{2} \right)\) – area of the circle;
\(\large \pi \approx 3.14\) – Pi number;
\(\large D \left(\text{m}\right)\) – diameter of the circle;
\(\large R \left(\text{m}\right)\) – radius of the circle;
The conductor can have more than just a cylindrical shape. The industry produces metal conductors having a square, rectangular, triangular or some other cross-section. It is clear that the areas of such sections must be calculated using other geometric formulas.
Current strength by definition
Current strength (current) is denoted by a capital Latin letter \(\large I\).
Direct current can be considered as a uniform directed movement of charged particles. Uniform means at the same speed.
If the current changes, then the speed of movement of the charges will also change.
Current strength is:
- physical quantity;
- the ratio of the charge passing through the cross section of the conductor to the duration of the period of time during which the charge passed.
The current is equal to the charge passing through the cross-section of the conductor in one second.
For direct current we use the formula:
\[\large \boxed{ I = \frac{\Delta q}{\Delta t} }\]
\(\large I \left(A\right)\) – current (current strength) in Amperes;
\(\large \Delta q \left( \text{Cl}\right) \) – charge in Coulombs passed through the cross section of the conductor;
\(\large \Delta t \left( c\right) \) – the period (slice) of time during which the charge passed;
\[\large \boxed{ 1 A = \frac{1 \text{Cl}}{1 c} }\]
If the electric current does not change either in magnitude or direction, then it is called constant.
If at least one of the characteristics changes, the current is called alternating. It will be different at different times. If you are given an equation that describes how the charge changes, then it is convenient to use the derivative to calculate such a current.
Eliminating confusion with the concept of strength
In physics, there has historically been the use of terms such as
- current strength,
- electromotive force,
- Horsepower.
These units of measurement have the word "force" in their name. It is known from mechanics that force is a vector quantity and is measured in Newtons. However, don't let this fool you.
None of the quantities described are measured in Newtons. The listed quantities have other units of measurement:
- current is measured in Amperes,
- electromotive force - in Volts,
- and horsepower is a unit of power that can be converted to SI Watts.
To avoid confusion, instead of the term “current strength”, you can use the word “current”. Compare the expressions: “Current strength is measured in Amperes” and “current is measured in Amperes.”
As you can see, it is quite possible to get by with the word “current” instead of “current strength”. This will not change the meaning.
Content
In the electrical phenomena we examined, we constantly observed the movement of electric charges. From mechanics you know that if there is any movement, then work is done.
What kind of work is done when electric charges move? Light bulbs in our houses and apartments, all the electrical appliances that we use every day - all this is a consequence of the work done by the electric field to move charges.
The next logical question immediately arises. How do these charges move? What makes them move? You have all heard about electric current , but have not yet looked inside this phenomenon using the tools of physics.
In this lesson you will learn what exactly is called electric current and how it is obtained.
What is 1 Ampere in SI system
The current strength of 1 Ampere was determined in the SI system using the force of mutual action of two current-carrying conductors.
Let's consider two thin conductors (Fig. 9). Each conductor has an infinite length. Let's place them in a vacuum in parallel at a distance of 1 meter from each other.
Rice. 9. Current standard 1 ampere in the SI system
Let us select a piece 1 meter long on each conductor.
If the conductors interact with a force of \(\large 2 \cdot 10^{-7} \) Newton per meter of their length, then a direct current of 1 Ampere flows through each of them.
The ampere is the SI base unit. And the charge Coulomb is a quantity determined using Ampere.
1 A coulomb is a charge passing through the cross-section of a conductor with a current of 1 Ampere in 1 second.
Who invented electricity - history
Particular manifestations of electricity were studied long before our era. But combining them into one theory that explains lightning flashes in the sky, the attraction of objects, the ability to cause fires and numbness of body parts or even the death of a person has proven to be a difficult task.
Since ancient times, scientists have studied three manifestations of electricity:
- Fish that generate electricity;
- Static electricity;
- Magnetism.
In Ancient Egypt, healers knew about the strange abilities of the Nile catfish and tried to use it to treat headaches and other diseases. Ancient Roman doctors used the electric stingray for similar purposes. The ancient Greeks studied in detail the strange abilities of the stingray and knew that the creature could stun a person without direct contact through a trident and fishing nets.
Somewhat earlier it was discovered that if you rub amber on a piece of wool, it will begin to attract hairs and small objects. Later, another material with similar properties was discovered - tourmaline.
Around 500 B.C. Indian and Arab scientists knew about substances capable of attracting iron and actively used this ability in various fields. Around 100 BC Chinese scientists invented a magnetic compass.
In 1600, William Gilbert, court physician to Elizabeth I and James I, discovered that the entire planet was one huge compass and introduced the concept of “electricity” (from the Greek “amber”). In his writings, experiments with rubbing amber on wool and the ability of a compass to point north began to be combined into one theory. In the picture below he is showing the magnet to Elizabeth I.
One Ampere - is it a lot or a little?
1 Ampere is 1 Coulomb divided by 1 second. For most household electrical appliances, this is a fairly large current.
For example, currents of 0.04 - 0.08 Ampere flow through energy-saving lamps.
A large flat-screen TV from an electric lighting network consumes a current of 0.2 Amperes.
Incandescent lamp - approximately 0.5 Ampere.
As you can see, most electrical appliances consume currents of less than one Ampere.
Therefore, submultiple units of measurement are often used for current:
milliamps, microamps, and nanoamps:
1mA (milliamp)= 10⁻³ A
1uA (microamp) = 10⁻⁶ A
1nA (nanoamp) = 10⁻9 A
The charging current for a mobile phone battery can reach 2 Amps.
And through an electric heater, or electric kettle, a current of up to 10 Amperes flows.
Note: Current as low as 0.05 A can be fatal. Be careful with electricity!
At the same time, currents exceeding hundreds of Amperes are also used. For example, in industrial power plants.
For such currents, multiple units are used: kiloampere, megaampere.
1KA (kiloampere) = 10³ A
1MA (megaamps) = 10⁶ A
The relationship between current strength and the speed of movement of charges
Consider a metal conductor. Let us mentally select two sections in it with an area \(\large S\) at a certain distance \(\large \Delta x\) from one another. The sections are located transverse to the conductor.
In metals, electric current is created by electrons. Let us denote \(\large e_{0}\) the charge of each electron.
Rice. 10. Free charges in the volume of a conductor
Charges in a conductor, under the influence of an electric field of intensity \(\large \vec{E} \) will move codirectionally, from section to section.
At the same time, they will travel the path \(\large \Delta x\) between the two sections.
If the current is constant, then the speed of movement of the charges will not change.
In this case, the distance \(\large \Delta x\) and the speed \(\large v\) of electron motion will be related by the formula of uniform motion.
\[\large \Delta x = v \cdot \Delta t\]
\(\large \Delta x \left( \text{m}\right) \) – distance between two cross sections;
\(\large v \left( \frac{\text{m}}{c}\right) \) – the speed with which charges move in a conductor in the same direction; This speed is significantly less than the speed of thermal motion.
\(\large \Delta t \left( c \right) \) – time interval during which the distance \(\large \Delta x\) between two cross sections is covered;
Let us express the time of movement from this formula:
\[\large \Delta t = \frac {\Delta x}{v} \]
We will need this expression further.
The sections \(\large S\) and the distance between them \(\large \Delta x\) form a cylindrical volume in the conductor:
\[\large V = S \cdot \Delta x\]
\(\large V \left( \text{m}^{3}\right) \) – volume of the cylinder;
This volume contains a certain number of electrons. Let's denote this quantity: \(\large N \) pieces.
The number of pieces \(\large N\) located in the volume \(\large V\) is called concentration:
\[\large n = \frac{N}{V} \]
\(\large n \left( \frac{\text{pieces}}{\text{m}^{3}}\right) \) – concentration of charges in the volume;
Let us find the total charge of all charged particles located in the volume \(\large V\) between two cross sections:
\[\large \Delta q = e_{0} \cdot N\]
Let's multiply the right side of the equation by one, which we represent as a fraction \(\displaystyle \frac{V}{V}\), in which \(\large V\) is the volume in question. Then the total charge can be written as follows:
\[\large \Delta q = e_{0} \cdot N \cdot 1 = e_{0} \cdot N \cdot \frac{V}{V}\]
Let's swap the numerator V of the fraction and the number N of particles.
\[\large \Delta q = e_{0} \cdot V \cdot \frac{N}{V}\]
Let's substitute the expression for volume into this formula:
\[\large \Delta q = e_{0} \cdot S \cdot \Delta x \cdot \frac{N}{V}\]
We replace the fraction on the right side with the concentration symbol “n”:
\[\large \Delta q = e_{0} \cdot S \cdot \Delta x \cdot n\]
Average speed of joint directed motion of charges \(\large v\).
Let's apply the definition of current strength:
\[\large I = \frac {\Delta q}{\Delta t} \]
Let us substitute into this expression the formula for the total charge passing through the cross section of the conductor:
\[\large I = \frac {\Delta q}{\Delta t} = \frac {e_{0} \cdot S \cdot \Delta x \cdot n}{\Delta t} \]
For convenience, the expression can be rewritten as follows:
\[\large I = e_{0} \cdot S \cdot \Delta x \cdot n\cdot \frac {1}{\Delta t} \]
We have expressed the time \(\large \Delta t\) in advance:
\[\large \Delta t = \frac {\Delta x}{v} \]
Let's find its inverse:
\[\large \frac {1}{\Delta t} = \frac {v}{\Delta x} \]
Let's substitute it into the formula for current:
\[\large I = e_{0} \cdot S \cdot \Delta x \cdot n \cdot \frac {v}{\Delta x}\]
The distance \(\Delta x\) is in the numerator and in the denominator, it will cancel. Finally, we obtain an expression for the relationship between the current strength and the speed of movement of charges:
\[\large \boxed{I = e_{0} \cdot S \cdot n \cdot {v}}\]
Now it can be argued that
- the more charges are placed in the volume,
- the faster they move in the same direction
- and, the thicker the conductor (the larger the cross-sectional area),
the greater the current.
Ammeter
We know where the current is directed, how the current is measured, how to calculate it, knowing the charge and the time during which this charge has passed. All that remains is to measure.
A device for measuring current is called ammeter . It is included in an electrical circuit in series with the conductor in which the current is measured .
Ammeters come in very different operating principles: electromagnetic, magnetoelectric, electrodynamic, thermal and induction - and these are just the most common.
We will only consider the principle of operation of a thermal ammeter, because to understand the principle of operation of other devices it is necessary to know what a magnetic field and coils are.
The thermal ammeter is based on the property of current to heat wires. It is designed like this: a thin wire is attached to two fixed clamps. This thin wire is pulled down by a silk thread connected to a spring. Along the way, this thread loops around the fixed axis on which the arrow is attached. The measured current is supplied to the fixed clamps and passes through the wire (arrows in the figure show the current path).
Under the influence of current, the wire will heat up slightly, causing it to elongate, as a result of which the silk thread attached to the wire will be pulled back by a spring. The movement of the thread will turn the axis, and therefore the arrow. The arrow will indicate the measurement value.
Calculation of the speed of directional movement of electrons
To do this, you can use the resulting formula:
\[\large I = e_{0} \cdot S \cdot n \cdot {v}\]
From this we can express the speed:
\[\large \boxed{\frac{I}{e_{0} \cdot S \cdot n} = v}\]
To find the speed at which electrons move in a conductor, you need to: divide the current (I) by the charge (e) of the electron, the concentration (n) of electrons and the cross-sectional area of the conductor (S).
Most connecting conductors are made of copper or aluminum. Let's choose a copper conductor that has a cylindrical shape.
Let's choose the cross-sectional area equal to 1 millimeter squared:
\[\large S = 10^{-6} \left( \text{m}^{2}\right) \]
The number of atoms in a volume - concentration, is related to the density of the substance (link). For copper, the atomic concentration is easy to calculate. She
\[\large n = 8.5 \cdot 10^{28} \left( \frac{\text{pieces}}{\text{m}^{3}}\right) \]
equal to the electron concentration.
Note: Each copper atom gives up one of its valence electrons and it becomes a free electron. Therefore, the number of free electrons located in the selected volume of copper will be equal to the number of atoms in this volume.
The electron charge is known:
\[\large e_{0} = 1.6 \cdot 10^{-19} \left(\text{Kl}\right) \]
Suppose a current of 1 Ampere flows in a conductor.
Then, the speed of electron movement:
\[\large v = \frac{1}{1.6 \cdot 10^{-19} \cdot 10^{-6} \cdot 8.5 \cdot 10^{28}} \]
\[\large v = 7 \cdot 10^{-5} \left( \frac{\text{m}}{c}\right) \]
This is less than 0.1 mm per second.
Rice. 11. Electrons in copper move slowly in a direction
The speed of propagation of the electric field and the speed of movement of charges - what are the differences?
It is necessary to distinguish between the speed at which the electric field propagates when a current source is connected to a conductor and the speed of movement of charged particles in the conductor.
The speed with which the electric field of intensity \(\large \vec{E}\) propagates is equal to the speed of light:
\[\large c = 3 \cdot 10^{8} \left( \frac{\text{m}}{c} \right)\]
And the speed of directional movement of charges is much less - less than 0.1 mm per second.
Rice. 12. Charges simultaneously participate in two movements
Note: As the speed of directional movement of free charges, choose the average speed at which the charges move during the flow of current. It is also called drift speed.
At the same time, at room temperature, the speed of random thermal motion of electrons is slightly more than 100 kilometers per second.
That is, the charges move quickly chaotically, but at the same time, they move consistently and quite slowly in a certain direction.
This movement can be compared to the movement of a stream of ants on a forest path. Each ant in the stream moves chaotically. But at the same time, the entire flow moves in concert in the chosen direction.
Rice. 13. The movement of ants and the movement of charges during the flow of electric current can be compared
Using an analogy from the surrounding nature, the movement of charged particles during the flow of electric current can be compared to the movement of ants.
Each particle moves chaotically under the influence of temperature and at the same time, all particles are displaced in one direction in the general flow under the influence of an electric field.
Conversion of radiation energy into electrical energy
Let's consider another interesting transformation of energies. Let's take a plate of silicon (or copper oxide, selenium). Let's point the switched-on lamp at it (Figure 6).
Figure 6. Conversion of radiation energy into electrical energy
Again we will see that current flows through the conductor. In this case, the plate loses its negative electrical charge and loses electrons.
This is how radiation energy (light from a lamp) turns into electrical energy. This phenomenon is called photoelectric effect , and such a current source is called a photocell .
You will study thermocouples and photocells in more detail in high school.
Conditions for the existence of direct current
Let me remind you that a current is called constant if its strength does not change over time.
To denote direct current, mathematicians use the following abbreviated notation:
\[\large \boxed{ I = const }\]
For a current to exist, several conditions must be met.
Rice. 14. For current to exist, the following conditions must be met
It is necessary that an electric field continuously exist between oppositely charged bodies. Also, there must be free charge carriers in the circuit. And the electrical circuit itself must be closed.
Let's consider these conditions in more detail.
A few words about the “polarity” of alternating voltage
Complex numbers are useful for analyzing AC circuits because they provide a convenient method of symbolically recording the phase shift between AC parameters such as voltage and current. However, most people have a hard time understanding the equivalence between abstract vectors and real circuit parameters. Earlier in this chapter, we saw how AC voltage sources are specified by voltage values in complex form (amplitude and phase angle), as well as polarity designations.
Because alternating current does not have a "polarity" parameter like direct current does, these polarity designations and their relationship to phase angle can be misleading. This section is written to clarify some of these issues.
Voltage, at its core, is a relative quantity. When we measure voltage, we have a choice of how to connect a voltmeter or other measuring device to the voltage source, since there are two points between which there is a potential difference, and two test leads on the device that need to be connected.
In DC circuits, we explicitly designate the polarity of voltage sources and voltage drops using "+" and "-" symbols, and we also use color-coded test leads (red and black). If a digital voltmeter shows a negative DC voltage, we know that its test leads are connected "reverse" to the voltage (the red wire is connected to "-" and the black wire is connected to "+").
Battery polarity is indicated by battery-specific symbols: the short line of the battery is always the negative (-) terminal, and the long line is always the positive (+):
Figure 1 – Common battery polarity designation
While it would be mathematically correct to represent the battery voltage as a negative value with reverse polarity notation, this would be clearly unusual:
Figure 2 - Completely non-standard polarity designation
Interpretation of such designations might be easier if the polarity designations "+" and "-" were considered as reference points for the test leads, meaning "red" and "-" meaning "black". A voltmeter connected to the above battery with the red probe on the bottom terminal and the black probe on the top terminal will indeed indicate a negative voltage (-6 volts).
In fact, this form of notation and interpretation is not as unusual as you might think: it is often found in DC circuit analysis problems, where the polarity signs "+" and "-" are first drawn according to an educated guess and then interpreted as correct or “reverse” in accordance with the mathematical sign of the calculated value.
However, in AC circuits we are not dealing with “negative” voltage values. Instead, we describe the extent to which one voltage is in or out of phase with another: i.e. by the time shift between two signals. We never describe an AC voltage as having a negative sign because polar notation allows vectors to point in opposite directions.
If one AC voltage is directly opposite to another AC voltage, we simply say that one voltage is 180° out of phase with the other.
However, the voltage between two points is relative, and we have a choice of how to connect the meter to measure the voltage between these two points. The mathematical sign of a DC voltmeter reading only matters in the context of its test lead connections: which terminal the red probe is connected to, and which terminal the black probe is connected to.
Additionally, the phase angle of the AC voltage only matters in the context of knowing which of these two points is considered the "reference". Therefore, to give the stated phase angle a reference point, diagrams often include polarity symbols "+" and "-" on the AC terminals.
We create a short-term current and find out the conditions for its existence
You can create an electric current using two oppositely charged bodies.
Current is the movement of charges. Therefore, it is necessary to ensure that the charges can move. That is, you need to create a path between the bodies along which charges will begin to move from one place in space to another.
The occurrence of current for a short period of time can be demonstrated using two electrometers charged oppositely.
Let's first try to connect two charged bodies with a piece of dielectric (Fig. 15).
Rice. 15. If two charged bodies are connected with a dielectric, no electric current occurs
As you can see, after connecting, the charge of each of the electrometers did not change.
This means that no current occurred. The fact is that in a dielectric all the electrons are bound to their atoms and there are no free electrons.
It is the free charges that will move and we will call their coordinated directional movement electric current.
Therefore, one of the conditions for the existence of current will be the presence of free charges. That is, the presence of a conductor containing such charges.
Condition 1. For current to exist, there must be free charges.
However, just having a conductor is not enough. Indeed, there are free charges in a conductor. But in order for these charges to begin moving together in a certain direction, they need to be acted upon by a force that will move them in this direction.
A force will act on a charged particle if it is placed in an electric field.
An electric field exists in space around charged bodies.
If you connect two bodies with opposite charges with a conductor, then an electric field will act on the free particles in the conductor. This field will pick up and force electrons to move in a certain direction.
Therefore, another condition for the occurrence of current is an electric field.
Rice. 16. After connecting with a conductor, the electrometers charged with opposite charges were discharged
Condition 2: For current to exist, an electric field must be present.
Rice. 17. Electrons move against the direction of the electric field
Current flows in the direction of movement of positive charges.
By connecting two charged metal bodies with a conductor, we receive current only for a short period of time. This time will be a fraction of a second.
In addition, at the initial moment of time the current strength will be greatest. And then it will decrease as the bodies are discharged and their potentials (link) are equalized.
We want the current to flow constantly, or at least for a sufficiently long period of time, chosen at our discretion. And so that during the flow of current its strength does not change.
How to achieve this? We are very close to the third condition for the existence of constant electric current.
Galvanic cell
One of the most common sources of current is a galvanic cell . We will use it in various experiments. Therefore, we will look at it in more detail.
What is a galvanic cell in simple words? This is a well-known battery to all of us.
Let's take a look inside it (Figure 7) to figure out how it works.
Figure 7. Galvanic cell
This element is basically (Figure 7, b) a zinc body 2 , inside of which there is a carbon rod 3 . At the upper end of this rod there is a metal cover 1 .
The rod is surrounded by a mixture of 4 manganese (IV) oxide $MnO_2$ and crushed carbon $C$. Between this mixture and the body itself there is a jelly-like solution of salt 5 (ammonium chloride $NH_4Cl$).
What's the point? The fact is that the zinc $Zn$, which the body is made of, interacts with ammonium chloride $NH_4Cl$. A chemical reaction is taking place. The zinc vessel acquires a negative charge.
But manganese oxide has a positive charge . The carbon rod transfers it to the metal lid.
So, we have a negatively charged body and a positively charged rod. These will be called electrodes . An electric field arises between them.
The very concept of an electrode is synonymous with the concept of a pole. “Electrode” is more often used when describing electrical phenomena and devices, while “pole” is more often used when talking about magnets.
Let's connect these two electrodes with a conductor. Electric current will flow through it. This is how the energy of chemical reactions is converted into electrical energy .
How to create a long-lasting current and what is needed for this
A positive charge is a lack of electrons, and a negative charge is an excess of them. At the moment the bodies are connected by a conductor, negative electrons rush to the positively charged body.
And in the end, the current stopped because the charges of the bodies were compensated and the bodies became electrically neutral. We know that neutral bodies do not create an electric field.
This means that the current exists as long as the electric field exists. Therefore, the electric field must be maintained in some way. And for this it is necessary that one of the bodies has an excess negative charge. That is, you need to maintain a negative charge on one of the bodies, and a positive charge on the other. As long as the charges of the bodies are maintained, the current will exist.
In order to maintain this charge on a body with a positive charge, you need to remove the electrons that have come running there from this body and send them back to the negatively charged body.
This design resembles a fountain in which a pump maintains a pressure difference. The pressure in the pipe injecting water is greater than in the pipe through which water flows back to the pump.
Rice. 18. The water flow circulates thanks to a pump that maintains a pressure difference
It is thanks to this difference that water splashes up from one pipe, and the water collected in the bowl flows back into the pump. At the same time, the same amount of water circulates through the circuit, that is, the water circuit is closed. And the water flow in this circuit is supported by a special device - a pump. It does work against gravity.
Rice. 19. The water pump in the fountain does work against gravity
Why is AC current dangerous for humans?
As already mentioned, a feature of AC voltage is the uniform flow of particles from one pole to the other. In comparison with DC current, it is considered less dangerous since in most cases it has a spasmodic effect on the human body. The spasm goes away immediately after the tension is relieved, which reduces the likelihood of critical results.
However, the absence of danger to the body is observed only in the case of a low value of direct current. The higher its value, the greater the likelihood of critical consequences. For example, when contacting voltages exceeding 500 V, current may be more dangerous than alternating current. However, in everyday life such values are absent and are used in transformers or substations, access to which is open only to specially trained people.
Important! The main difference between the effects of high-voltage current on a person is the strong knockback effect (compared to alternating current).
What is more dangerous for humans?
Variable AC poses a great danger to the human body. Under its influence, sharp fibrillation of the heart ventricles occurs. But this does not mean that direct current can be considered safe. People caught under such tension suffer severe injuries as a result of kickback and mechanical shock.