Magnetic and electric fields are often considered together, being, so to speak, two sides of the same coin. Both of these fields have a lot in common. For example, they both create electric charges . Any electrically charged body is affected by the Coulomb force. It is also called the force of electrostatic interaction. It is directly proportional to the product of the charge modules (the signs of the charges determine only the direction of the force: attraction or repulsion) and inversely proportional to the square of the distance between these bodies. In the case of spheres or balls, the square of the distance from the centers of the bodies is calculated.
What is an electric field?
In physics, this concept is usually understood as a vector field that is formed around particles or bodies with a certain charge. The electric field is considered one of the two integral components of the electromagnetic field.
To better understand the nature of this phenomenon, you need to remember what the Coulomb force is. Coulomb's law serves to determine the degree of interaction between each of a pair of point electric charges. At the same time, it takes into account information about the interval between them.
To understand the tension of the phenomenon, it is worth turning to this example:
- There are 2 bodies that have a charge. In this case, one of them is stationary, and the second moves around the first.
- The Coulomb force in this case is equal to the product of charge and voltage.
- The tension will include the central charge parameter and the square of the distance from the center to the second body.
It is noteworthy that for each point of the electric field the Coulomb force parameter and direction will be different. Due to the difference in directions at different points, the concept is considered vector.
What common
Unfortunately, the first common property of the concepts we are considering is their incomplete research. Despite the works of James Clerk Maxwell, which examined in detail the interaction and relationship between electric and magnetic fields, their nature cannot still be called fully understood, and in fact, humanity today can only use their known properties for practical purposes. However, scientists know a lot today.
For example, both fields are integral components of the electromagnetic field, and both are also forces. The latter concept implies that at any point within the radius of the field, a certain force acts on the charge, and when the position changes, the value of the force will change.
What is a magnetic field?
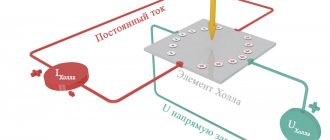
In physics, this term is understood as a force field that affects exclusively moving bodies, particles or charges. Each element is characterized by a magnetic moment. The force in this case depends less on the movement of the charge. In this case, electrons act as charged particles. As for the strength of this type of field, the value will be in direct proportion to the speed of the charge and its parameters.
The best example is planet Earth. Its central part consists of red-hot iron. Like other metal objects, it can move electrons around it. That is why the largest magnetic field on Earth is formed by the planet itself, or its center, to be more precise. If this field disappears, there is a high probability of disasters and even the death of living organisms.
Expert opinion
Karnaukh Ekaterina Vladimirovna
Graduated from the National University of Shipbuilding, majoring in Enterprise Economics
A more standard example of such a concept is electromagnets. They typically involve wires that are wrapped around ferromagnets. These elements are a series of substances that acquire magnetic characteristics only if their temperature is below a specific level. The last parameter is called the Cure temperature in physics. In fact, ferromagnets are considered unique elements. They interact with the magnetic field, but do not carry moving charges.
Examples of magnetic fields
An electromagnet consists of a wire wrapped around a ferromagnet. When current passes through a wire, a magnetic field appears. A ferromagnet is a substance that can behave like a magnet below a certain temperature, called the Curie temperature . Under normal conditions, ferromagnets behave like magnets only in the presence of a magnetic field. In an electromagnet, the field is created by an electric current, and the ferromagnet begins to behave like a magnet. Another interesting example is the Earth's magnetic field .
Earth's magnetic field
In the center of our planet, according to scientists, there is a core consisting of liquid iron. Iron is a metal, and electrons move freely in it. This nucleus is not static, that is, it moves, and therefore electrons move and create a magnetic field. If the Earth's core were to stop moving, as it did in John Amiel's film The Earth's Core, the Earth's magnetic field would actually disappear, with catastrophic consequences.
What is the difference between an electric field and a magnetic field?
Both concepts under consideration are considered power. This means that at each point in space where the field acts, a specific force affects the charge. At another point its value will be different. The electromagnetic field affects charged bodies and particles. Moreover, it acts on all charges, while the magnetic field acts exclusively on moving ones.
There are substances that interact with a magnetic field, but do not involve moving charges. These include, in particular, ferromagnets. This concept differs from the electric field, since similar substances do not exist for it. Magnets, natural or magnetized bodies, have 2 poles. They are called southern and northern.
Expert opinion
Karnaukh Ekaterina Vladimirovna
Graduated from the National University of Shipbuilding, majoring in Enterprise Economics
Ordinary electric charges are considered relatively homogeneous. They do not include poles. Moreover, such charges are characterized by 2 types - positive and negative. The sign affects the direction of the Coulomb force. As a consequence, this affects the interaction of two charged particles. The sign will not affect the interaction of other charged particles with the magnetic field. It will only reverse the poles.
The graphic representation of the physical phenomena under consideration is also different. Electric field lines have a beginning and an end. They can be visualized. An example is quinine crystals in oil. The induction lines are closed. They can also be visualized. An example of this is metal filings.
Separately, it is worth mentioning the electromagnetic field, which has the characteristics of both an electric and a magnetic field. This means that it is capable, under certain conditions, of turning the compass needle and moving electrically charged particles. Both components have a close relationship with each other. Each of them differs in its energy reserve. It is this that influences the energy of the entire electromagnetic field.
Expert opinion
Karnaukh Ekaterina Vladimirovna
Graduated from the National University of Shipbuilding, majoring in Enterprise Economics
The emergence of an electromagnetic field is possible with any, even small, change in current in conductors. At the same time, it influences the adjacent zones of space and transfers its own energy to them. As a result, an electromagnetic field also appears in these places.
Textbooks
Belkin I.K. Electric and magnetic fields // Quantum. - 1984. - No. 3. - P. 28-31.
By special agreement with the editorial board and editors of the journal “Kvant”
Around any electric charge q
There is, as is known, an electrostatic field.
If this charge is a point charge, then the field strength at any point at a distance r
from it is determined by the formula:
\(~E = \frac{1}{4 \pi \varepsilon_0} \frac{q}{r^2}\) .
(1) The force of interaction between two point charges at rest q
and
q'
, located at a distance
r
from each other, is equal to
\(~F_{el} = \frac{1}{4 \pi \varepsilon_0} \frac{qq'}{r^2}\) .
(2) If the charges are the same ( q
=
q'
), then
\(~F_{el} = \frac{1}{4 \pi \varepsilon_0} \frac{q^2}{r^2}\) .
(2′) This is the well-known Coulomb law. We emphasize that this law was established experimentally. ε included in formulas (1), (2)
0 is an electrical constant equal to 8.85·10-12 C2/(N·m2).
Let us now discuss the situation with a charge moving at a constant speed \(~\vec \upsilon\). We measure the speed \(~\vec \upsilon\) relative to a pre-selected reference system, which we conventionally consider stationary and denote by the letter K
.
A moving charge is an electric current, and there is a magnetic field around the current. This means that the movement of the charge leads to the appearance of a magnetic field in the reference frame K
.
Please note that if we were to move to the reference frame K'
, moving relative to the system
K
with a speed equal to the speed of the charge \(~\vec \upsilon\),
there would be no magnetic field in this system
(the magnetic needle (compass) placed there would remain at rest).
Let us, however, return again to the K
. From experience we know that a magnetic field acts on an electric current, and therefore on a moving charge. It is clear that there must be a close connection between electrical and magnetic phenomena.
Let's look at a simple example. Let charge q
moves with speed \(~\vec \upsilon\) (in the
K
).
Then in the space around it there are both electric and magnetic fields. Let now at some point where the electric and magnetic fields are created by a charge q
, there appears to be another charge
q'
, which moves with a speed \(~\vec \upsilon\).
Obviously, electric and magnetic forces will act on it. A similar statement can be made about the charge q
.
If the charges move at speeds that are not too high (what “not too high” means will be clarified below), then their electric fields at each moment of time are practically no different from the case of charges at rest, and the force of electrical interaction between charges can be calculated according to Coulomb’s law (2) .
We will further restrict ourselves to the case of not very fast moving charges. In this case, you can compare magnetic and electric forces quite simply. We will do this for two charges, the velocities of which at some moment turned out to be parallel to each other and perpendicular to the line connecting these charges (Fig. 1).
Rice. 1
First, we need to understand how the magnetic field induction \(~\vec B\) created by a moving charge is related to the magnitude of this charge q
and its speed \(~\vec \upsilon\).
Let us draw from the point where the charge q
to the point at which we want to calculate the induction \(~\vec B\), the radius vector \(~\vec r\).
A generalization of the experimental results shows that the magnitude of the induction is determined by the formula \(~B = kq \frac{\upsilon}{r^2} \sin \varphi\) .
(3) Here \(~r = |\vec r|\), φ
is the angle between the vectors \(~\vec \upsilon\) and \(~\vec r\), and
k
is a constant determined experimentally and equal to 10-7 N·s2/Cl2.
For the case of interest to us, φ
= 90° and
\(~B = k\upsilon \frac{q}{r^2}\) .
(3′) The direction of the vector \(~\vec B\) is determined by the gimlet rule (right screw): if you rotate the vector \(~\vec \upsilon\) towards the vector \(~\vec r\) in that plane , in which they both lie, and so that the rotation occurs in the direction of the minimum angle between \(~\vec \upsilon\) and \(~\vec r\), then the vector \(~\vec B\) is directed perpendicular to the indicated plane and “looks” where a screwed-in gimlet would “look” (Fig. 2).
Rice. 2
Formulas (3) and (3′) include as a factor the same ratio \(~\frac{q}{r^2}\), which is also contained in the expression for the electric field strength created by charge q
at the point where the charge
q'
.
This remark allows us to establish a connection between the
quantities
B
and
E. Taking into account (1), we obtain \(~B = k\upsilon \frac{q}{r^2} = 4 \pi \varepsilon_0 k \upsilon E\) .
Let us also denote \(4 \pi k = \mu_0\), then
\(~B = \varepsilon_0 \mu_0 \upsilon E\) . (4)
μ included in (4)
0 is called the magnetic constant (by analogy with the electrical constant
ε
0).
Knowing k
, we calculate
μ
0 = 1.256·10-6 N·s2/Cl2.
Now that we can calculate the magnetic induction B
at the point where the charge
q'
, it is possible to determine the magnetic force (Lorentz force) acting on the charge
q'
.
In the general case, the Lorentz force \(~F_L = q' \upsilon' B \sin \alpha\) .
(5) Here α
is the angle between the vectors \(~\vec \upsilon'\) and \(~\vec B\). The direction of the vector \(~\vec F_L\) is also determined by the right gimlet rule (the vector \(~\vec \upsilon\) must be rotated in the direction of the minimum angle to the vector \(~\vec B\) in the plane in which both of these vectors, the vector \(~\vec F_L\) is perpendicular to this plane).
In our case, angle α
is also equal to 90°, and
the force \(~F_m = q' \upsilon' B\) acts
q' .
Substituting B
from (3′), we obtain
\(~F_m = k \frac{q q' \upsilon \upsilon'}{r^2} = \frac{\mu_0}{4 \pi} \frac{q q' \upsilon \upsilon '}{r^2}\) .
(6) Electric force acting between q
and
q'
, is determined by formula (2).
Let us now also assume that q
=
q'
, and \(~\vec \upsilon = \vec \upsilon'\).
Then the relation \(~\frac{F_m}{F_{el}} = \varepsilon_0 \mu_0 \upsilon^2\) .
ε determined from experience
0 and
μ
0, we can easily verify that
\(~\frac{1}{\varepsilon_0 \mu_0} = 0 \cdot 10^{16} \frac{m^2}{c^2}\) .
But the quantity on the right in this formula is the square of the speed of light! This is, of course, not a coincidence. Denoting the speed of light with the letter c
, we finally get:
\(~\frac{1}{\varepsilon_0 \mu_0} = c^2\) .
(7) and
\(~\frac{F_m}{F_{el}} = \left( \frac{\upsilon}{c} \right)^2\) . (8)
These are very important formulas. Formula (7) once again confirms the existence of a deep connection between electrical and magnetic phenomena. From formula (8) we can conclude that the ratio of magnetic and electric forces for many practically interesting cases turns out to be small. For example, the usually directional speed of electrons in a metal during the passage of current is tenths of a millimeter per second. In such cases, the magnetic interaction of electrons is approximately 1024 times less than their electrical interaction. Is it worth considering the magnetic interaction of moving charges at all? It turns out it’s worth it and here’s why. In any conductor there are equal numbers of charged particles of both signs. Therefore, current-carrying conductors are electrically practically neutral. Electrical forces in conductors are compensated with great precision, and in their absence, even very small magnetic forces are significant. In electric motors, electrons moving in their windings interact precisely with magnetic forces. It is due to magnetic forces that motors are driven.
Let us now remember that in our reasoning we made the assumption that “speeds are not too high.” Now we can say that we meant the small speed of particles compared to the speed of light. We needed this so that there would be no need to use more complex (relativistic) formulas. For example, at velocities of charged particles close to the speed of light, it is no longer possible to write the formula for the electric field strength in the form (1).
Just like other physical quantities, for example, the speed and acceleration of particles, the forces acting on them, the electric field strength and magnetic field induction depend on the frame of reference in which we consider (measure) them. We have already said above, for example, that if a magnetic field exists in the system K
, then this does not mean that it will be preserved in the
K'
.
The system K'
, which we considered, moved with a speed equal to the speed \(~\vec \upsilon\) of the charge we were interested in.
And there was no magnetic field in the K'
.
In the general case, both the vector \(~\vec E\) and the vector \(~\vec B\) are relative
, that is, they depend on the reference system in which the observer is located with instruments that measure these quantities.
What is important for us is that at speeds small compared to the speed of light, the electric field changes little. The quantitative characteristic of this smallness is precisely the ratio \(~\left( \frac{\upsilon}{c} \right)^2\).
Note, however, that formula (8) also turns out to be valid at speeds close to the speed of light. Therefore, for relativistic particles moving at a speed only slightly less than the speed of light, the magnitudes of magnetic and electric forces may not differ very much.
To summarize our calculations, we emphasize once again that for many problems the magnetic interaction turns out to be significant, even if it is small compared to the electrical one.
Let us dwell very briefly on other differences between magnetic and electric (electrostatic) fields. One of these differences is that the lines of action of electric forces (electrostatic field lines) have a beginning and an end. They start on positive charges and end on negative ones. The work done when a charge moves between two fixed points in an electrostatic field does not depend on the shape and length of the trajectory, and the work done when the charge moves along a closed path is zero. Thanks to this property of the electrostatic field, each point is characterized not only by tension, but also by potential.
Magnetic induction lines, unlike electrostatic field lines, do not have a beginning and an end. They are closed and cover the current. Each point of the magnetic field is characterized by the magnitude of magnetic induction, but not by potential.
The force acting on a charge in an electrostatic field is always directed tangentially to the lines of force. If we consider sections of current-carrying conductors or moving charged particles in a magnetic field, then the forces acting on them are directed not tangentially to the induction lines, but perpendicular to them (Lorentz force, Ampere’s law). The force in a magnetic field may not cause translational motion at all, but only rotation (a frame with current in a uniform magnetic field; movement of a charge in a circle in a plane perpendicular to a uniform magnetic field).
All of the above suggests that the electrostatic field is in many ways unlike the magnetic field. The connection between electric and magnetic fields does not mean that there are no differences between them.
comparison table
The main features and differences of the concepts under consideration are shown in the table:
| Criterion | Electric field | A magnetic field |
| Field Source | Electric charge. | Magnet, current. |
| Field detection | When charged particles interact. | When magnets and conductors interact with current. |
| Graphic image | Lines of force or lines of tension. | Lines of force or lines of magnetic induction. |
| Character of lines | They have a beginning and an end. The beginning of the lines of force is on positive charges, and the end is on negative charges. | They are closed. The lines leave the north pole and enter the south pole. They are closed in the magnet. |
| Interaction of elements | Charged particles of the same name attract, charged particles of the same name repel. | Opposite magnetic poles attract, like magnetic poles repel. |
| Power characteristic | The tension vector is measured in newtons per coulomb. | Magnetic induction vector, unit of measurement is tesla. |
| Field indicators | Small pieces of paper Electric Sultan Electric sleeve. | Metal filings Magnetic needle Closed circuit with current. |
| Superposition principle | The field strength at a certain point is equal to the vector sum of the field strengths that are created by each of the charges separately. | The magnetic induction of the resulting field is the vector sum of the induction of fields that are created by each source separately. |
What is the difference
As is clear from all of the above, there are also many distinctive features between the phenomena we are considering. First of all, electric fields are capable of influencing all charged particles, while the magnetic field “works” only on those particles that are in motion. If we talk about the EF force, then it will be proportional to the charge, and the MF will be proportional not only to the charge, but also to the speed of its movement.
Reference. In this case, the units of measurement also differ: for EP it is volts per meter, and the MF voltage is expressed in tesla (T) or gauss (G).
Another interesting property is the fact that in an electromagnetic field both of its components will oscillate at right angles relative to each other. This and other features of the interaction were reflected in his equation by James Clerk Maxwell, who spent many years studying magnetic and electric fields.
So we looked at the main difference and common features between two closely related concepts in physics. We hope that in this material you were able to learn something new and interesting for yourself.