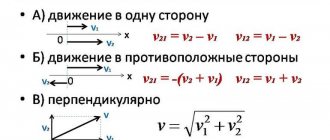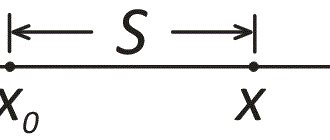Linear speed
Each point on the circle moves at a certain speed. This speed is called linear. The direction of the linear velocity vector always coincides with the tangent to the circle.
For example, sparks from under a grinding machine move, repeating the direction of instantaneous speed.
Consider a point on a circle that makes one revolution, the time spent is the period T
. The path that a point travels is the circumference.
Rotation angle and period of revolution
Consider point A on an object rotating around its axis. When circulating over a certain period of time, it will change its position on the circle line by a certain angle. This is the rotation angle. It is measured in radians, because the unit is a segment of a circle equal to the radius. Another value for measuring the angle of rotation is a degree.
When, as a result of the rotation, point A returns to its original place, it means that it has completed a full rotation. If its movement is repeated n times, then we speak of a certain number of revolutions. Based on this, you can consider 1/2, 1/4 turn and so on. A striking practical example of this is the path that a cutter takes when milling a part fixed in the center of the machine spindle.
Attention! The rotation angle has a direction. It is negative when the rotation occurs clockwise and positive when it rotates counterclockwise.
If a body moves uniformly around a circle, we can talk about a constant angular velocity during movement, ω = const.
In this case, the following characteristics are used:
- period of revolution – T, this is the time required for a full revolution of a point in a circular motion;
- circulation frequency – ν, this is the total number of revolutions that a point makes along a circular path in a unit time interval.
Interesting. According to known data, Jupiter revolves around the Sun every 12 years. When the Earth makes almost 12 revolutions around the Sun during this time. The exact value of the round giant's orbital period is 11.86 Earth years.
Centripetal acceleration
When moving in a circle, the acceleration vector is always perpendicular to the velocity vector, directed towards the center of the circle.
Using the previous formulas, we can derive the following relationships
Points lying on the same straight line emanating from the center of the circle (for example, these could be points that lie on the spokes of a wheel) will have the same angular velocities, period and frequency. That is, they will rotate the same way, but with different linear speeds. The further a point is from the center, the faster it will move.
The law of addition of speeds is also valid for rotational motion. If the motion of a body or frame of reference is not uniform, then the law applies to instantaneous velocities. For example, the speed of a person walking along the edge of a rotating carousel is equal to the vector sum of the linear speed of rotation of the edge of the carousel and the speed of the person.
Linear and angular velocities
Linear speed
Definition and formulas
Linear speed is the ratio of the distance traveled to the time during which this path was traveled. Denoted by the letter v. The unit of measurement is m/s.
l is the length of the trajectory along which the body moved during time t
Linear speed can be expressed in terms of a period. In one period, the body makes one revolution, that is, it travels a path equal to the length of the circle. Therefore its speed is:
R is the radius of the circle along which the body moves
If linear speed can be expressed in terms of a period, then it can also be expressed in terms of frequency - the reciprocal of the period. Then the formula will take the form:
Expressing the frequency in terms of the number of revolutions and the time during which the body made these revolutions, we obtain:
Angular velocity
Definition and formulas
Angular velocity is the ratio of the angle of rotation of a body to the time during which the body made this rotation. Denoted by the letter ω. The unit of measurement is radian per second (rad/s).
ϕ is the angle of rotation of the body. t is the time during which the body rotated through an angle ϕ
Useful facts
Radian is the angle corresponding to an arc whose length is equal to its radius. The total angle is 2π radians.
In one full revolution, the body rotates 2π radians. Therefore, the angular velocity can be expressed in terms of a period:
Expressing the angular velocity in terms of frequency, we get:
Expressing the frequency in terms of the number of revolutions, the formula for angular velocity takes the form:
Let's compare two formulas:
Let's transform the formula for linear speed and get:
From here we obtain the relationship between linear and angular velocities:
Useful facts
- For rotating cylinders pressed against each other, the linear velocities of points on their surface are equal: v1 = v2.
- For rotating gears, the linear velocities of points on their surface are also equal: v1 = v2.
- All points of a rotating rigid body have the same periods, frequencies and angular velocities, but different linear velocities. T1 = T2, ν1 = ν2, ω1 = ω2. But v1 ≠ v2.
Example No. 2. The period of revolution of the Earth around the Sun is one year. The radius of the Earth's orbit is 150 million km. What is the approximate speed of the Earth's orbital motion? Round the answer to whole numbers.
There are 365 days in a year, one day has 24 hours, one hour has 60 minutes, and one minute has 60 seconds. Multiplying all these numbers together, we get the period in seconds.
Every second the Earth travels a distance of approximately 30 km.
Connection to Newton's second law
According to Newton's second law, the cause of any acceleration is force. If a moving body experiences centripetal acceleration, then the nature of the forces that cause this acceleration may be different. For example, if a body moves in a circle on a rope tied to it, then the acting force is the elastic force.
If a body lying on a disk rotates with the disk around its axis, then such a force is the friction force. If the force stops its action, then the body will continue to move in a straight line
Cyclic speed (reversal)
A scalar quantity that measures the frequency of rotational motion is called cyclic speed. This is the angular frequency, which is not equal to the angular velocity vector itself, but to its magnitude. It is also called radial or circular frequency.
Cyclic rotation frequency is the number of body revolutions in 2*π seconds.
For AC electric motors, this frequency is asynchronous. Their rotor speed lags behind the rotation speed of the stator magnetic field. The value that determines this lag is called slip - S. During the sliding process, the shaft rotates because an electric current arises in the rotor. Slip is permissible up to a certain value, exceeding which leads to overheating of the asynchronous machine, and its windings may burn out.
The design of this type of motor differs from the design of DC machines, where a current-carrying frame rotates in the field of permanent magnets. The armature contained a large number of frames, and many electromagnets formed the basis of the stator. In three-phase AC machines the opposite is true.
When an asynchronous motor operates, the stator has a rotating magnetic field. It always depends on the parameters:
- mains frequency;
- number of pole pairs.
The rotation speed of the rotor is in direct relation to the speed of the stator magnetic field. The field is created by three windings, which are located at an angle of 120 degrees relative to each other.
Read also: Repair of Audi 100 dispenser
Movement along a cycloid*
In the reference frame associated with the wheel, the point rotates uniformly along a circle of radius R with a speed that changes only in direction. The centripetal acceleration of a point is directed radially towards the center of the circle.
Now let's move to a stationary system connected to the ground. The total acceleration of point A will remain the same both in magnitude and direction, since when moving from one inertial reference system to another, the acceleration does not change. From the point of view of a stationary observer, the trajectory of point A is no longer a circle, but a more complex curve (cycloid), along which the point moves unevenly.
Instantaneous speed is determined by the formula
Period, frequency and number of revolutions
Let the body move in a circle continuously. When it makes one revolution, some time will pass. When the body makes another revolution, the same amount of time will pass. This time will not change, because the body moves with a constant modulus speed. This time is called a period.
A period is the time of one complete revolution. Denoted by the letter T. The unit of measurement is seconds (s).
t is the time during which the body made N revolutions
During the same period of time, a body can pass only part of a circle or make several units, tens, hundreds or more revolutions. It all depends on the circumference and speed module.
Frequency is the number of revolutions made per unit of time. Denoted by the letter ν (“nu”). The unit of measurement is Hz.
N is the number of revolutions made by the body during time t.
Period and frequency are reciprocal quantities , determined by the formulas:
The number of revolutions is expressed by the following formula:
Example No. 1. The ball on the string rotates in a circle. In 10 seconds it made 20 revolutions. Find the period and frequency of rotation of the ball.
Uniformly accelerated motion in a circle with an initial angular velocity
The initial velocity of the body, equal to ?0 at the moment t = 0, changes uniformly by the amount ??. (The angular acceleration is constant.)
?0—initial angular velocity? — final angular velocity ? — angular displacement of the body during time t in radians t — time ? — angular acceleration is constant during time t
Since on the velocity graph the angular displacement corresponds to the area of the trapezoid under the velocity curve, we have:
Since the area of a trapezoid is equal to the sum of the areas of the triangle and rectangle forming it, we obtain:
Further from the speed graph it follows
Combining the formulas we get
After the transformation we get an expression that does not contain time:
Speed concept
When we compare the movement of any bodies, we say that some bodies move faster, while others move slower. We use such simple terminology in everyday life, talking, for example, about traffic. In physics, the speed of movement of bodies is characterized by a certain value. This quantity is called speed. General definition of speed (if the body is moving uniformly):
Velocity during uniform motion of a body is a physical quantity that shows how far the body has traveled per unit time.
By uniform motion of a body it is meant that the speed of the body is constant. The formula for finding speed: $v=frac$, $s$ is the path traveled by the body (that is, the length of the line), $t$ is the time (that is, the period of time during which the path was traveled).
According to the international SI system, the unit of linear speed is derived from two basic units - the meter and the second, that is, it is measured in meters per second (m/s). This means that the unit of speed is the speed of such uniform motion that a body travels a distance of one meter in one second.
Try asking your teachers for help
Speed is also often measured in km/h, km/s, cm/s.
Let's look at a simple example of a speed calculation problem.
Task. Moving uniformly, the train covers 219 km in 4 hours. Find its speed of movement.
Solution. $v=frac<219 km><4 h>=54.75frac<km><h>$. Let's convert kilometers into meters and hours into seconds: $54.75frac<km><h>=frac<54750 m><3600c>approx 15.2frac<m>$.
From the example we see that the numerical value of the speed differs depending on the selected unit of measurement.
In addition to the numerical value, speed has a direction. The numerical value of a quantity in physics is called the modulus. When a physical quantity also has a direction, then this quantity is called a vector quantity. That is, speed is a vector physical quantity.
In writing, the velocity module is denoted by $v$, and the velocity vector by $vec v$.
In turn, such quantities as path, time, length and others are characterized only by a numerical value. Then they say that these are scalar physical quantities.
Read also: Audi Q5 operating regulations
Ask a question to the experts and get an answer within 15 minutes!
In the case when the movement is uneven, the concept of average speed is used. Average speed formula: $v_<aver>=frac$, where $s$ is the entire path traveled by the body, $t$ is the entire time of movement. Let's look at an example of a medium speed problem to understand the difference.
Task. Some vehicles cover a distance of 213 km in 2.5 hours. Find it $v_<av>$.
Answer. $85.2 frac<km><h>$ or $23.7frac<m> <s>$.