Where:
- N – number of revolutions around an axis or in a circle,
- t is the time during which they were completed.
In the SI system it is denoted as – s-1 (s-1) and is referred to as revolutions per second (rps). Other units of rotation are also used. When describing the rotation of planets around the Sun, they speak of revolutions in hours. Jupiter rotates once every 9.92 hours, while the Earth and Moon rotate every 24 hours.
Rated rotation speed
Before defining this concept, it is necessary to determine what the nominal operating mode of a device is. This is the order of operation of the device in which the greatest efficiency and reliability of the process are achieved over a long period of time. Based on this, the nominal rotation speed is the number of revolutions per minute when operating in nominal mode. The time required for one revolution is 1/v seconds. It is called the rotation period T. This means that the relationship between the rotation period and frequency has the form:
For your information. The rotational speed of the asynchronous motor shaft is 3000 rpm, this is the rated rotation speed of the output shaft shank at the nominal operating mode of the electric motor.
How to find or find out the rotation frequencies of various mechanisms? For this, a device called a tachometer is used.
Speed
A characteristic of all types of rotation is the number of revolutions n or an equivalent characteristic - frequency f. Both quantities characterize the number of revolutions per unit time.
SI unit of frequency (or number of revolutions)
In engineering, the number of revolutions is usually measured in revolutions per minute (rpm) = 1/min.
Thus, the reciprocal of the number of revolutions is the duration of one revolution.
If n is the number of revolutions, f is the frequency, T is the duration of one revolution, period, ? — angular displacement, N — total number of revolutions, t — time, duration of rotation, ? is the angular frequency, then
Period
Angular movement
The angular movement is equal to the product of the total number of revolutions by 2?:
Angular velocity
From the formula for one revolution it follows:
Please note: • the formulas are valid for all types of rotational motion - both for uniform motion and for accelerated motion. These can include constant values, average values, start and end values, and any instantaneous values. • contrary to its name, the number of revolutions n is not a number, but a physical quantity. • a distinction must be made between the number of revolutions n and the total number of revolutions N.
Angular velocity
When a body moves in a circle, not all its points move at the same speed relative to the axis of rotation. If we take the blades of an ordinary household fan that rotate around a shaft, then the point located closer to the shaft has a rotation speed greater than the marked point on the edge of the blade. This means they have different linear rotation speeds. At the same time, the angular velocity of all points is the same.
Angular velocity is the change in angle per unit time, not distance. It is denoted by the letter of the Greek alphabet – ω and has a unit of measure: radians per second (rad/s). In other words, angular velocity is a vector tied to the axis of rotation of the object.
The formula for calculating the relationship between rotation angle and time interval is:
Where:
- ω – angular velocity (rad/s);
- ∆ϕ – change in the angle of deflection when turning (rad.);
- ∆t – time spent on deviation ©.
The designation of angular velocity is used when studying the laws of rotation. It is used to describe the motion of all rotating bodies.
Angular velocity in specific cases
In practice, they rarely work with angular velocity values. It is needed in the design development of rotating mechanisms: gearboxes, gearboxes, etc.
You can calculate it using the formula. To do this, use the connection between angular velocity and rotational speed.
Where:
- π – number equal to 3.14;
- ν – rotation speed, (rpm).
As an example, the angular velocity and rotational speed of the wheel disk when moving a walk-behind tractor can be considered. It is often necessary to reduce or increase the speed of the mechanism. To do this, a device in the form of a gearbox is used, with the help of which the speed of rotation of the wheels is reduced. At a maximum speed of 10 km/h, the wheel makes about 60 rpm. After converting minutes to seconds, this value is 1 rpm. After substituting the data into the formula, the result will be:
Rotational movement of the body, formulas
During the rotational motion of a rigid body, all elements of its mass that do not lie on the axis of rotation move in a circle. Similarly, a material point located at a distance r > 0 from the axis of rotation also moves in a circle, like any body sufficiently distant from the axis of rotation.
Linear displacement Sl, linear speed uL and linear acceleration al during such movement are interconnected by the usual relations for translational motion.
In addition, these quantities are related in a certain way to the angular displacement ?, angular velocity ? and angular acceleration ?.
| Sl | moving a body along a trajectory, | meter |
| Ul | body speed when moving along a trajectory, | meter/second |
| al | acceleration of a given body when moving along a trajectory, | meter/second2 |
| r | trajectory radius, | meter |
| d | trajectory diameter, | meter |
| ? | angular movement of the body, | radian |
| ? | angular velocity of the body, | radian/second |
| ? | angular acceleration of the body, | radian/second2 |
| f | frequency, | Hertz |
Note: The formulas are valid for constant, instantaneous and average quantities, in all cases of body motion in a circle.
Rotation angle and period of revolution
Consider point A on an object rotating around its axis. When circulating over a certain period of time, it will change its position on the circle line by a certain angle. This is the rotation angle. It is measured in radians, because the unit is a segment of a circle equal to the radius. Another value for measuring the angle of rotation is a degree.
When, as a result of the rotation, point A returns to its original place, it means that it has completed a full rotation. If its movement is repeated n times, then we speak of a certain number of revolutions. Based on this, you can consider ½, ¼ turn and so on. A striking practical example of this is the path that a cutter takes when milling a part fixed in the center of the machine spindle.
Attention! The rotation angle has a direction. It is negative when the rotation occurs clockwise and positive when it rotates counterclockwise.
If a body moves uniformly around a circle, we can talk about a constant angular velocity during movement, ω = const.
In this case, the following characteristics are used:
- period of revolution – T, this is the time required for a full revolution of a point in a circular motion;
- circulation frequency – ν, this is the total number of revolutions that a point makes along a circular path in a unit time interval.
Interesting. According to known data, Jupiter revolves around the Sun every 12 years. When the Earth makes almost 12 revolutions around the Sun during this time. The exact value of the round giant's orbital period is 11.86 Earth years.
Uniformly accelerated motion in a circle without initial angular velocity
The body begins to move from a state of rest, and its angular velocity uniformly increases.
? — instantaneous angular velocity of the body at time t? — angular acceleration, constant during time t? - angular displacement of the body during time t, (? in radians) t - time
Since on the velocity graph the angular displacement is equal to the area of the triangle, we have:
Since the rotation of the body begins from a state of rest, the change in angular velocity ?? equal to the angular velocity ? achieved as a result of acceleration. Therefore, the formula takes the following form:
Cyclic speed (reversal)
A scalar quantity that measures the frequency of rotational motion is called cyclic speed. This is the angular frequency, which is not equal to the angular velocity vector itself, but to its magnitude. It is also called radial or circular frequency.
Cyclic rotation frequency is the number of body revolutions in 2*π seconds.
For AC electric motors, this frequency is asynchronous. Their rotor speed lags behind the rotation speed of the stator magnetic field. The value that determines this lag is called slip - S. During the sliding process, the shaft rotates because an electric current arises in the rotor. Slip is permissible up to a certain value, exceeding which leads to overheating of the asynchronous machine, and its windings may burn out.
The design of this type of motor differs from the design of DC machines, where a current-carrying frame rotates in the field of permanent magnets. The armature contained a large number of frames, and many electromagnets formed the basis of the stator. In three-phase AC machines the opposite is true.
When an asynchronous motor operates, the stator has a rotating magnetic field. It always depends on the parameters:
- mains frequency;
- number of pole pairs.
The rotation speed of the rotor is in direct relation to the speed of the stator magnetic field. The field is created by three windings, which are located at an angle of 120 degrees relative to each other.
Video
The work on rebuilding the electric motor is nearing completion. Let's start calculating the machine's belt drive pulleys. A little terminology on belt drives.
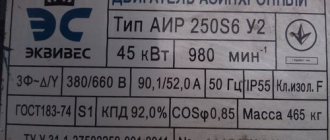
Our main initial data will be three values. The first value is the rotation speed of the rotor (shaft) of the electric motor 2790 rpm. The second and third are the speeds that need to be obtained on the secondary shaft. We are interested in two ratings: 1800 and 3500 rpm. Therefore, we will make a two-stage pulley.
The note! To start a three-phase electric motor, we will use a frequency converter, so the calculated rotation speeds will be reliable. If the engine is started using capacitors, the rotor speed will differ from the nominal value downward. And at this stage it is possible to reduce the error to a minimum by making amendments. But to do this you will have to start the engine, use a tachometer and measure the current shaft rotation speed.
Our goals have been determined, let’s move on to choosing the type of belt and moving on to the main calculation. For each of the belts produced, regardless of the type (V-belt, poly-V-belt or other), there are a number of key characteristics. Which determine the rationality of use in a particular design. The ideal option for most projects is to use a serpentine belt. It got its name polycuneiform due to its configuration; it is like long closed grooves located along the entire length. The name of the belt comes from the Greek word "poly", which means many. These furrows are also called differently - ribs or streams. Their number can be from three to twenty.
A poly-V-belt has a lot of advantages over a V-belt, such as:
- Due to good flexibility, work on small pulleys is possible. Depending on the belt, the minimum diameter can range from ten to twelve millimeters;
- high traction capacity of the belt, therefore the operating speed can reach up to 60 meters per second, versus 20, maximum 35 meters per second for a V-belt;
- The adhesion force of a poly V-belt to a flat pulley at a wrap angle greater than 133° is approximately equal to that of a grooved pulley, and as the wrap angle increases, the adhesion force becomes higher. Therefore, for drives with a gear ratio of more than three and a small pulley angle of 120° to 150°, a flat (without grooves) larger pulley can be used;
- Due to the light weight of the belt, vibration levels are much lower.
Taking into account all the advantages of multi-V belts, we will use this type in our designs. Below is a table of the five main sections of the most common V-belts (PH, PJ, PK, PL, PM).
| P.J. | PK | P.L. | P.M. | ||
| Fin pitch, S, mm | 1.6 | 2.34 | 3.56 | 4.7 | 9.4 |
| Belt height, H, mm | 2.7 | 4.0 | 5.4 | 9.0 | 14.2 |
| Neutral layer, h0, mm | 0.8 | 1.2 | 1.5 | 3.0 | 4.0 |
| Distance to neutral layer, h, mm | 1.0 | 1.1 | 1.5 | 1.5 | 2.0 |
| Minimum pulley diameter, db, mm | 13 | 20 | 45 | 75 | 180 |
| Maximum speed, Vmax, m/s | 60 | 60 | 50 | 40 | 35 |
| Length range, L, mm | 1140…2404 | 356…2489 | 527…2550 | 991…2235 | 2286…16764 |
Drawing of a schematic designation of the elements of a poly-V-belt in section.